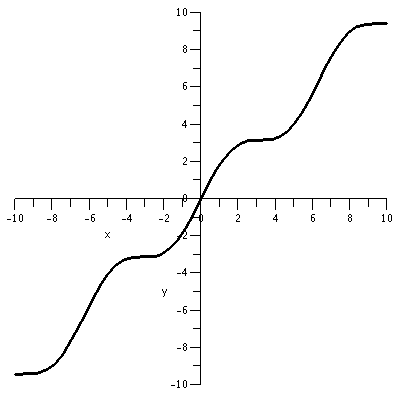
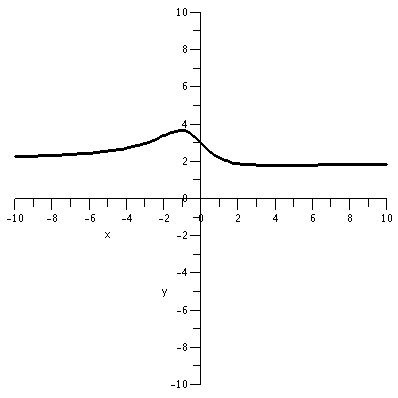
For Monday, August 14
Please finish reading chapter 10. Do as many of problems 5 through 15 as you can (in terms of time, energy, and appetite!).

For Thursday, August 10
Skim chapter 9. Then begin to read chapter 10. The material in chapter 10 will be covered in today's lecture and Thursday's lecture. Please do problems 1, 2, 3, 4, 16, and 17 in chapter 10.

For Tuesday, August 8
Finish reading chapter 8. Begin to look at chapters 9 (short!) and 10: this is the climax of the course.

For Tuesday, August 8
Please read 8.1 and 8.2. Do these problems: chapter 8: 1, 6, 7, 8, 9.

For Thursday, August 3
I distributed copies of the formula sheet which students will have on the second exam and a copy of the second exam given by the coordinator of Math 135 in the fall 2005 semester. Please reserve 80 minutes and work on the exam. You can check your answers here.

For Thursday, August 3
I distributed copies of the formula sheet which students will have on the second exam and a copy of the second exam I gave the last time I taught Math 135. Please reserve 80 minutes and work on the exam. You can check your answers here.
My "vision" of the material covered by the second exam may be helpful.

For Monday, July 31
Please do these problems.

For Thursday, July 27
Please read chapter 7. Do these problems: 15, 17, 23, 25, and 26 in chapter 7. I hope that students will present them at the board on Monday.

I also hope that I will return the take-home semi-exam on Monday.

For Wednesday, July 26
A plethora of optimization problems were assigned to various (12!) different students.

For Tuesday, July 25
Find all horizontal and vertical asymptotes of f(x)=5x/|x-1| and y=sin(x)/x.

For Monday, July 24
Earn as much as 40 points towards the course grade by handing in these problems which are due at the beginning of class on Monday, July 24. So this is, essentially, a take-home semi-exam. You must use calculus to explain your answers!

As a help to those who may not feel as comfortable with "machinery" as one would hope, here is the output of what I've been calling Step 0 for each of the functions in problems 1 through 4. These are the graphs in the window -10≤x≤10 and -10≤y≤10. Please note that these graphs are not definitive. They may not show certain things in enough detail, and they might suggest things which may not be true. The formulas I gave you were generally not selected with malice, but graphing devices are not supernatural -- they won't reveal everything that you might want to see!

Problem 1 f(x)=(x-1)/e2x	Problem 2 f(x)=(x3+1)/x
Problem 3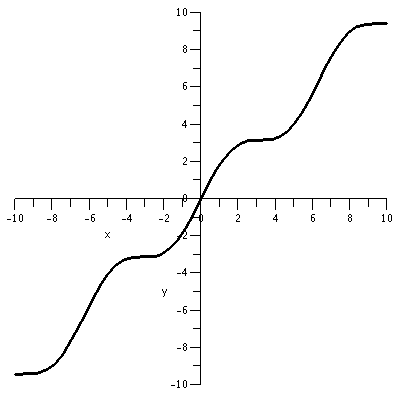 f(x)=x+sin(x)	Problem 4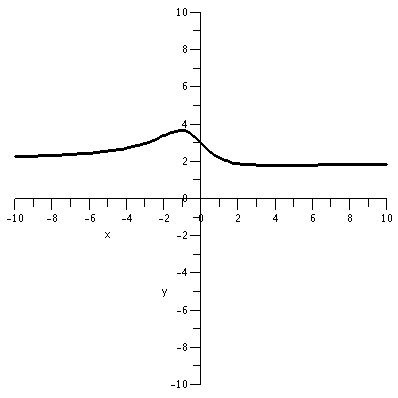 f(x)=(2x2+9)/(x2+x+3)

For Wednesday, July 18
Please graph the following functions, according to the procedures used in class. I would like you to answer the following questions:
On the FUNC level
What is the domain of f(x)? What is the range of f(x)? What is the behavior of f(x) "near" points not in the domain of f(x)? (Geometrically, this means discussing the vertical and horizontal asymptotes of y=f(x).)
On the FUNC' level
What (if any!) are the critical numbers and critical points of f(x)? Are they {relative|absolute} {max|min}'s? In what intervals does f(x) increase? In what intervals does f(x) decrease?

Here are the functions.

The first function is f(x)=(3x+5)/sqrt(x2+2) This is a quotient, and the / separates the top and the bottom. The result of what I've been calling Step 0 (a graph in the window with -10≤x≤10 and -10≤y≤10) is shown to the right.

The second function is f(x)=(x2+1)/[(x+2)(x-3)] This is a quotient, and the / separates the top and the bottom. Again, a possible Step 0 is shown to the right. I have been "kind" because I chose an option on my graphing software that essentially advises it to look for and discard bad information which may result from discontinuities. Otherwise, it would draw, in addition to what's shown, two vertical lines!

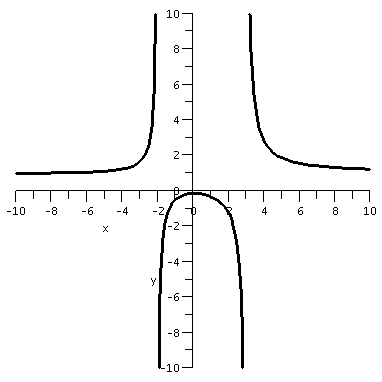
For Tuesday, July 18
Please do 6.5: 1, 5, 12, 14, 15.

For Thursday, July 13
I gave out copies of the formula sheet which students will have on the first exam and the first exam given by the coordinator of Math 135 in the fall 2005 semester. I urged students to set aside 80 minutes and work on the exam. You can check these answers.
I also remarked that students should review their performance on the earlier "semiexam" given in this course. If I asked the question once, I will likely still find the question "interesting".

For Wednesday, July 12
I gave out copies of the formula sheet which students will have on the first exam and a Math 135 exam. I urged students to set aside 80 minutes and work on the exam. You can check these answers.

For Tuesday, July 11
Do these problems from Chapter 5 of the text (section 5.5, pp.101-102): 14, 15, 16, 20, 21, 23, 25.
Also please do these, which throw in the trig functions and the exponential function: Find f´(x) if
A) f(x)=sin(x²)
B) f(x)=cos(5x-3)/e^{cos x}
C) f(x)=(3x⁷+5e^2x)(88x+1)⁶
D) f(x)=cos([2-x⁴]²²)
E) f(x)=([2x+7]/[3-5x²])³
If you would like to check your answers to A and B and C and D and E (but please try them, first!), look here.

For Monday, July 10
First read this.
Do these problems from Chapter 5 of the text (section 5.5, pp.101-102): 1, 3, 4, 5, 6, 9, 10, 11, 13.
Also please do these, which throw in the trig functions: Find f´(x) if
A) f(x)=56+17sin(x)
B) f(x)=-3tan(x)(4x+5sqrt(x))
C) f(x)=cos(x)/[x²-9x]
D) f(x)=(3+7sin(x))(6x³-9tan(x))
If you would like to check your answers to A and B and C and D (but please try them, first!), look here.

For Thursday, July 6
Please read chapter 4 and sections 5.1 and 5.2 of the text. Do these problems: 4.6: 6, 8:
Compute the derivatives of the following functions, using only the definition of derivative:
6. g(t)=1/(t²+1)
8. p(t)=sqrt(4t-2).

For Monday, July 3
There will be an exam. Review problems were handed out, and some solutions will be discussed Monday. Some hints are here. Send e-mail to me if you have further questions, please. Solutions will be given by student volunteers (indeed!). This will be followed by a bit of lecture, then a sort of half an exam.
I fell behind in writing notes. I will try to catch up in the next half day (written late on Thursday afternoon).

Due Thursday, June 29
Please read sections 2.3, 2.4, 3.1, and 3.2 of Gootman's book.

A problem from chapter 2 (p. 45):
Gootman's book states: "For exercises 9-12, you can use the following formula for the derivative of a quadratic function: If f(x)=ax²+bx+c, then f´(x)=2ax+b."
11. Let f(x)=x²-4x+4.
a. At which point on the graph of f is the tangent line horizontal?
b. At what point on the graph of f does the tangent line have slope 10?
c. At what point on the graph of f does the tangent line have slope 1/10?

From chapter 3, p. 65:
Gootman's book states: "... either evaluate the given limit (as a real number or as infinity or -infinity), or state that the limit does not exist."
2. lim_x-->2{x²+4}/{x+8}.

3. lim_x-->1sqrt{7x+9}.

Due Wednesday, June 28
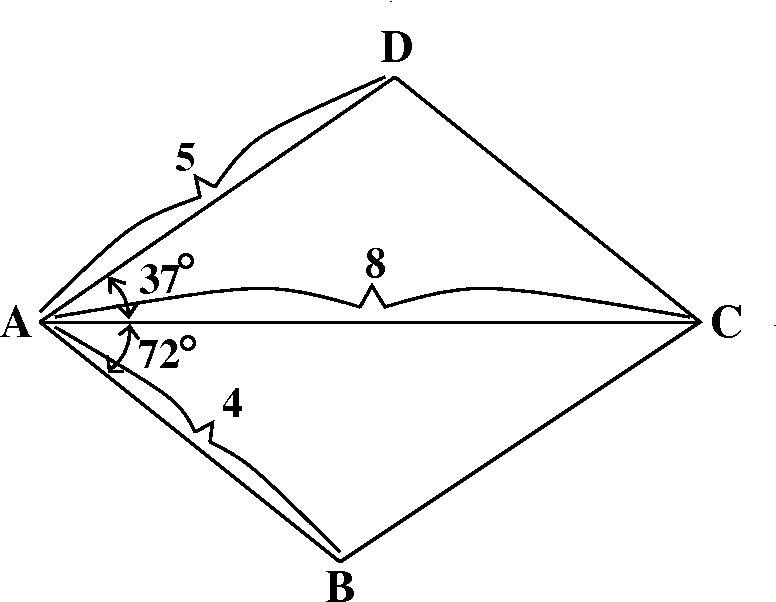Please read sections 2.1 and 2.2 of Gootman's book. There is some review of trig in sections A.1 and A.2 (appendix A, in the back of the book).

1. Consider the quadrilateral ABCD shown to the right.

• Edge facts
  • The edge AB is 4 units long.
  • The edge AC is 8 units long. The edge AD is 5 units long.

• Angle facts
  • The angle BAC is 72°
  • The angle CAD is 37°

b) Compute the distance from B to D.
Note I don't know or particularly want the answers to these questions (!) but I hope you will show me your methods of doing them. Then I can assess your knowledge of trigonometry.
I apologize sincerely. The problem given in class specified 56^o for the angle which is given as 72^o (angle BAC) in the diagram and specifications shown above. I am sorry.

2. Suppose f(x)=7x²-2x.
a) Compute f(3).
b) Compute f(3+h).
c) Compute {f(3+h)-f(3)}/h (the average rate of change of f(x) on the interval [3,3+h]).
d) Simplify your answer.
e) What happens to your simplified answer as h gets very small? (This is the instantaneous rate of change of f(x) at 3.)
Note Please do these computations in the simple (unsophisticated) method used in class today. There are more rapid methods which we will learn about, but I'd like to see if you can work with the basic idea.

Due Tuesday, June 27
Please read chapter 1 of Gootman's book. Ask questions, either in person or by e-mail, about any material which is confusing in the text or in lecture.
Please hand in solutions to the following problems tomorrow (from Page 14 of Gootman's book). Note that bare answers are in the back of the book. I would like to see your methods, not just your answers, so please write your solutions in detail. This will help me to teach you better.

3. For each of the following equations, first solve for y as a function of x and then solve for x as a function of y. In each case, state the domain of the function.
4x³y-6y=5.

4. For the function f(x)=2x²-4x+1, evaluate and simplify each of the following:
a. f(4)
b. f(-3)
c. f(x+1)
d. f(2x)
e. f(a)
f. f(x+h)

6. In freehand, draw a curve that can be thought of as the graph of a function.

7. In freehand, draw a curve that cannot be thought of as the graph of a function.

Answers to some questions due on Tuesday, July 11:
A) f´(x)=cos(x²)(2x)
B) f´(x)=

{-sin(5x-3)(5)ecos x}-{cos(5x-3)ecos  x(-sin(x)}
----------------------------------------------
              (ecos x)2

Answers to some questions due on Monday, July 10:
A) f´(x)=-+17cos(x)
B) f´(x)=-3(sec(x))²(4x+5sqrt(x))+(-3tan(x))(4x+5(1/2)x^-1/2)
C) f´(x)=

-sin(x)(x2-9x)-(2x-9)cos(x)
---------------------------
           (x2-9x)2

Maintained by greenfie@math.rutgers.edu and last modified 7/11/2006.