Exam results for Math 421:03, fall 2004
The first exam
| | Problem
#1 |
Problem
#2 |
Problem
#3 |
Problem
#4 |
Problem
#5 |
Problem
#6 |
Problem
#7 |
Extra credit
(RREF) |
Total |
|---|
| Max grade |
16 |
14 |
12 |
12 |
20 |
14 |
12 |
5 |
103 |
|---|
Min grade |
0 |
2 |
0 |
2 |
0 |
0 |
0 |
0 |
8 |
Mean grade |
7.56 |
10.08 |
9.39 |
6.42 |
10.17 |
10.94 |
7.47 |
4.03 |
66.06 |
Median grade |
6 |
12.5 |
11 |
3.5 |
10 |
14 |
8.5 |
5 |
67 |
36 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. Here are
approximate letter grade assignments for this exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [85,100] | [80,84] | [70,79] |
[65,69] | [60,64] | [55,59] | [0,54] |
|---|
Discussion of the grading
Generally arithmetic errors will be penalized only minimally. If,
however, your error makes the problem much simpler, more credit will
be deducted.
Some comments which students should read are given in red
below.
The alert student will notice a certain resemblance between the
exam given here and the first exam given
in my section of Math 421 last spring.
|
The Oxford English Dictionary records the first printed
appearance of the word lazy in 1549. The OED states that the
primary meaning of lazy is
"Averse to labour, indisposed to action or effort; idle; inactive,
slothful." |
Problem 1 (16 points)
a) (10 points) A routine computation using integration by parts,
although errors are easy! It will help in part b) if you get the
correct answer. Of course, a small part of the purpose of part b) is
to check your answer. Students were specifically asked to use the
definition to compute the Laplace transform in this problem. Other
methods did not earn any points, but a correct answer was eligible for
points in part b).
b) (6 points) Careful use of l'Hopital's rule is a major part of
justifying some of the computations of the "operational calculus".
Comment Several students got incorrect answers to a) and then
proceeded to get answers to b) which, through garbled computation, led
to 1/2. These students have deficiencies in integrity or ability or
both, perhaps.
Problem 2 (14 points)
This is a standard ODE problem which can be solved with various
techniques (several from 244, for example). I think this problem is a
bit easier than the corresponding one in the previous exam I gave.
a) (10 points) 4 points for taking the Laplace transform of the
equation. 4 points for solving the partial fractions problem and
setting up the equation for the inverse Laplace transform. 2 points
for "reading off" the solution correctly from the tables.
b) (4 points) The points are for checking that the initial conditions
are satisfied. Of course, this should help check your answer (as part
b) was intended to help in the first question).
Comments Of course one basis of the solutions of the ODE
y´´-y=0 is {et,e-t}. Another basis is
{cosh(t),sinh(t)} which some students used in this problem. Students
should know the relationshops between these bases (in particular, what
are cosh and sinh in terms of et and
e-t). Students who used the sinh/cosh basis should know how
to differentiate these functions, and should also know their values
at, say, t=0. This was covered on the first day of class. The answer
in terms of sinh/cosh is 5cosh(t)+4sinh(t)-2. I penalized
students who wrote solutions in terms of cos(i t) and
sin(i t): they had better show me that they knew what they
were discussing in terms of standard functions.
I note also that several students wrote formulas for y(t) which
obviously did not satisfy the initial conditions, yet, in part b),
these students just wrote that the initial conditions were indeed
satisfied. Perhaps this is a joke, but to me again this may indicate a
serious deficiency of either ability or integrity (or both!). Would
you want to hire a person who did something like that, or work
with such a person?
Problem 3 (12 points)
Straightforward application of one of the translation theorems and the
table of formulas. Essentially I tried to grade using the following
guidelines: correct appearance of e-2s would get 3
points. Correct statement of an applicable translation theorem earns 3
more points. Use of the translation theorem is 3 points, and finally,
writing the (correct!) inverse Laplace transform is worth the last 3
points.
Problem 4 (12 points)
This problem is a bit more difficult than the corresponding problem in
my old exam. I increased the point value a bit. 3 points for knowing
that the Laplace transform of the convolution is the product of the
convolutions (this is on the formula sheet, so you need to have
instantiated the result with the appropriate functions). The
result is NOT the convolution, as a number of students
seemed to think, but is the Laplace transform of the convolution. The
inverse Laplace transform of this product must be computed. So 3
points for realizing the correct form of the partial fraction
decomposition necessary, 3 more for finding the correct values of the
constants, and a final 3 points for finding the correct inverse
Laplace transform.
Problem 5 (20 points)
This problem is worth one-fifth of the exam. I don't think I could
solve this ODE easily without the Laplace transform, or even
understand a mathematical statement of the problem very well without
using the Dirac and Heaviside functions!
a) (10 points) Here taking the Laplace transform earns 4 points, 4
points for "massaging" the Laplace transform (mostly another partial
fractions exercise), and 2 points for writing the inverse
transform. If a serious error is made in this part which "trivializes"
(makes much easier!) some or all of the successive parts of the
problem, then I will not give points for those parts.
b) (6 points: 2 points for each part) I am happy to accept an
unsimplified formulas in various intervals although that will make c)
and d) more difficult. Especiallly interesting to me are students who
told me that y(t) was not 0 before Pi/2: this ideal spring
starts in equilibirum (consider the initial conditions) and nothing
happens to it until Pi/2. It should not move at least until Pi/2!
c) (2 points) This is actually a very simple graph. You can get
enough information by evaluating at 0, Pi/2, Pi, and 3Pi/2. Even if
you didn't simplify previously, you should be "suspicious" about the
graph.
Comment The "geometry" of the axes supplied was
supposed to be a hint, also. I will not consciously try to mislead
students by supplying ludicrously inappropriate coordinate axes in
such a problem.
d) (2 points) Maybe this is the theoretical part of the exam, although
maybe engineers should be concerned about shocks. That y(t)
is not differentiable at only one value of t is subtle to me.
Certainly the solution is differentiable everywhere except Pi/2
and Pi.
Problem 6 (14 points)
I gave 5 points for writing the symbolic linear combination. Then
correct use of a RREF or other method to get a solution earns the
remainder of the problem's credit, and I reserved (at least) 2 points
for a clear statement of the correct solution. I do not believe that
the New Brunswick RREF can be used to solve this problem.
To get a significant number of points, I need to be satsified that the
student knows what a linear combination is, in the context of this
problem. The problem is very easy (two lines or three?) with the use
of Piscataway.
Comment I believe that sometimes computation can be an
effective teaching and learning device, I don't like to subject
students to large amounts of pointless computation, especially on an
exam. If you find yourself doing that on one of my exams, it may be
time to think about your methods.
Problem 7 (12 points)
Students should know and "manifest" what is needed to verify linear
independence. Then the linear system has to be set up and solved. 4
points for knowing about linear independence. 8 points for manipulating
the system correctly and showing that the functions are
linearly independent.
Engineers You can indeed survive an exam in an upper-level math
course where one of the questions has the word "Prove" in its
statement.
Extra credit (5 points)
I gave 5 points to students who were able to take a "random" (?)
matrix produced by Maple and convert it to RREF. Ample
opportunities for retakes were offered.
Comment Only 29 of 36 students got 5 points
this way.
The second exam
| | Problem
#1 |
Problem
#2 |
Problem
#3 |
Problem
#4 |
Problem
#5 |
Problem
#6 |
Problem
#7 |
Extra credit
(BLOCK) |
Total |
|---|
| Max grade |
12 |
22 |
16 |
12 |
18 |
12 |
8 |
5 |
93 |
|---|
Min grade |
2 |
4 |
0 |
2 |
5 |
0 |
0 |
0 |
34 |
Mean grade |
7.82 |
17.76 |
4.15 |
6.97 |
12.62 |
9.92 |
4.68 |
2.35 |
66.26 |
Median grade |
7 |
20 |
2.5 |
7 |
13 |
11 |
4 |
0 |
68.5 |
34 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. Here are
approximate letter grade assignments for this exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [85,100] | [80,84] | [70,79] |
[65,69] | [60,64] | [55,59] | [0,54] |
|---|
Discussion of the grading
Generally arithmetic errors will be penalized only minimally. If,
however, your error makes the problem much simpler, more credit will
be deducted. I wrote the following, which I hoped students would
accept as axioms for my exams, at least.
Please
- Do my problems, not those you invent.
- I cannot read your mind or imagine your answers: what you write is
what I will read (and grade).
- If you do extensive computation, you are probably not doing my
problem (see 1) or you are doing my problem inefficiently.
Problem 1 (12 points)
6 points for each part. I will read what was written. I
can not guess what you meant to write. I required that what was
written be responsive to what was asked. In part a), an important "If
... then ..." needed to be inferred from what you wrote (for example,
"If [a certain linear combination is 0] then [all the
coefficients are 0]". Or "The only way a certain linear combination is
0 is if all the coefficients are 0". I also accepted statements about
none of the vectors being linear combinations of the others. But I
read what you wrote, and tried to read it carefully.
I took 2 points off in part b) if the definition used AX=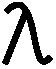 X and did not specify that X must be
non-zero. This specification is important. If a definition using the
equation det(A-
X and did not specify that X must be
non-zero. This specification is important. If a definition using the
equation det(A-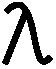 In)=0
was given, there was no such risk. Again, I read what students wrote,
and did not guess at what they did not write.
In)=0
was given, there was no such risk. Again, I read what students wrote,
and did not guess at what they did not write.
Comment Since we spent a major portion of the
course on linear algebra, and linear independence and
eigenvalue are principal ideas in what we covered,
students should be able to tell me what the phrases mean.
Problem 2 (22 points)
a) (2 points) Take the determinant of A-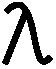 I. You may compute this determinant in any way you like.
I. You may compute this determinant in any way you like.
b) (2 points) You may have already simplified the characteristic
polynomial in part a), or you can do it here. The roots should be
obvious.
c) (4 points) You need to solve three (3) homogeneous systems of
linear equations. But they all are rather simple.
d) (2 points) You are merely asked to write P and D, which you
certainly should be able to do after parts b) and c). Verification of
your statements occurs in the next few parts of the problem. If
incorrect results from b) and c) are used correctly here, I
gave full credit.
e) (3 points) You may find P-1 in any way you like. The
answer is easy enough to check, so I gave 1 point out of 3 for an
incorrect answer. The exam I handed out, by the way, had two parts labeled
d. That is fixed in the posted version.
f) (2 points) Compute the product requested.
g) (2 points) Compute the product requested. If you do not get a
correct diagonal matrix, I gave no points.
h) (5 points) 2 points for setting up the requested relationship
A6=PD6P-1. 1 point for computing
D6 and 2 points for computing A6 (information
was given allowing you to check your answer).
Problem 3 (16 points)
a) (8 points) A restatement of the definition in the language of the
problem (that is, writing an arbitrary linear combination of
the functions of the problem, setting this equal to 0, etc.) earns 2
points. The balance is earned when the answer contains verification
that the coefficients of the linear combination must be 0.
b) (8 points) The correct answer (No) gets 2 points. The cover
page states, "An answer alone may not receive full credit." Correct
supporting evidence is needed for the other 6 points.
Comment Please see the lecture of October 5
for analysis of a similar example. There are also similar examples in
some review problems.
Problem 4 (12 points)
In this problem, I expected students to evaluate the determinant (8
points). A few students tried other strategies (using RREF), and one
or two of these students were successful. Students who "plugged in"
values for a and b and c were simplifying the problem too much and can
earn at most 3 of these 8 points.
I hoped the determinant evaluation would be combined with the
knowledge that a matrix is singular (not invertible) exactly
when the determinant is 0 (2 points). This then could be matched up
with the perpendicularity condition to get the desired conclusion (2
points).
Problem 5 (18 points)
It is possible to make mistakes in a) and have serious effects on work
in b) and c). If the result of a) were as complex as the correct
answer, points were only taken off in a). A similar approach was
followed for errors in b).
a) (5 points) One application of integration by parts. Keep track
of the n's and the signs.
b) (4 points) Evaluate the antiderivative. Notice that sin(nPi) and
sin(n 0) are 0 and that cos(nPi)=(-1)n and
cos(n 0) is 1. I took off 2 points for errors which really fouled
up the answers in c) (examples: omitting the sign "flip" or omitting
the Pi).
c) (2 points) The points were earned if the result of b) was used
correctly.
d) (7 points)
The left-hand graph (4 points)
In the graph of the partial
sum, I looked for the following qualitative behavior:
- Continuity on [0,Pi] with matching values at 0 and Pi
- The value 0 at both 0 and Pi.
- Closeness (with "wiggling") to the line segment inside the
interval.
- Gibbs phenomena (overshoot) at both ends
I took off a point for each feature that was missing.
The right-hand graph (3 points)
This is supposed to be the graph of the sum of the whole Fourier sine
series. Here the behavior required was much simpler:
- Identical to x+1 except at the ends (1 point)
- 0 at both ends (1 point each)
Problem 6 (12 points)
With orthogonality, there is almost no computation in this problem
(yes, other than small integers). I'll take off 1 point if the
normalization constant is misquoted (I did this in my own solution of
the problem, so I would have scored 99 at most). If the integrals are
computed as the sum of squares of the coefficients with no constant
(or, better, with the constant=1) I will take 2 points off.
Some students antidifferentiated instead of differentiating, and this
will lose 2 points.
I took off 3 points for an error I didn't anticipate, an answer which
essentially declares that the integral of (a negative
number)2 is negative. I can't read people's minds, and I
don't know on what level this error was made: through fatigue and
nervousness under exam conditions, or because of serious
misunderstanding.
Problem 7 (8 points)
I am not satisfied with the statement of this problem. The statement
might have made the problem more difficult for students. I wanted to
ask: what is the polynomial formula when x<0 for the odd
(respectively, even) extension of the polynomial x+x4? I
think now I should just have asked exactly that. The statement
of the question(s) seemed to invite misinterpretation.
4 points for each part, with 2 points for the specification of x<0,
1 point for x>0, and 1 point for identifying which Fourier
coefficients must be 0.
I'll give 1 point on each part to people who correctly write the
Fourier sine (respectively, cosine) series for F(x) (respectively,
G(x)).
I'll put a "cleaner" version of the question in the posted exam, but
I'll also show the wording I actually used, since I believe that
weakened student efforts on this problem.
Extra credit (5 points)
I gave 5 points to students who presented answers to some questions about matrices in block
form.
Comment Only 16 of 34 students got 5 points
this way.
The final exam
| Problem |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
Extra credit
(Maple pictures) |
Total |
|---|
| Max grade |
10 |
22 |
18 |
20 |
20 |
20 |
12 |
16 |
18 |
18 |
5 |
174 |
|---|
Min grade |
2 |
3 |
3 |
1 |
0 |
0 |
0 |
0 |
0 |
1 |
0 |
42 |
Mean grade |
8.45 |
12.45 |
15.06 |
13.81 |
13.67 |
8.74 |
10.94 |
7.77 |
9.42 |
11.87 |
3.42 |
115.61 |
Median grade |
10 |
12 |
18 |
13 |
14 |
6 |
12 |
8 |
9 |
14 |
5 |
119 |
31 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. Here are
approximate letter grade assignments for this exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [147.9,174] | [139.2,147.9] | [121.8,139.2] |
[113.1,121.8] | [104.4,113.1] | [95.7,104.4] | [0,95.7] |
|---|
Problem 1 (10 points)
2 points for getting the e-2s as a result of
U(t-2). Then 2 points for rewriting the remainder in terms of
t+2. 2 points for "expanding" (t+2)3 correctly, and 2
points for clarifying the exponential as
e10e5t. Finally, 2 points for writing out the
complete answer.
Problem 2 (22 points)
a) (14 points) 4 points for taking the Laplace transform of the ODE. 6
points for changing it algebraically (including partial fractions)
into a more manageable form. 4 more points for the inverse Laplace
transform.
b) (2 points) 1 point each for correct formulas.
c) (4 points) 1 point for y(0), 2 points for the correct derivative,
and 1 more point for y´(0).
d) (2 points) 1 point for the correct answer, and 1 point for some
reasonable explanation.
Problem 3 (18 points)
a) (6 points) A correct definition of linearly independent should be
given. In particular, I again looked for an appropriate "If ... then
..." statement (or one which could be recognized as such).
b) (12 points) I looked for a verification that the given
functions were linearly independent. There are various ways to do
this while considering a linear combination of the functions. For
example, we could consider special values of x (such as x=1 and
x=2). Or we could consider the coefficients of the quadraric
polynimials and examine the
coefficient matrix of the resulting homogeneous system.
Problem 4 (20 points)
Although the individual computations in this problem are easy,
the chance of making at least one mistake while doing the problem
seems large.
a) (2 points) Get the characteristic polynomial.
b) (3 points) Get the eigenvalues. It is easy to guess one root, and
then "deflate" the results (lower the degree by one).
c) (4 points) Get eigenvectors for each eigenvalue.
d) (2 points) Write D and P.
e) (5 points) Find the inverse of the P declared in the previous
section. The computation can be done either by row reduction or using
the adjoint. If the suggested P and P-1 are much simpler
than the correct ones, only 3 of 5 points can be earned.
f) (2 points) Compute Z=AP. The points are earned by doing a
correct matrix multiplication.
g) (2 points) Compute P-1Z. No points are earned unless the
result is D.
Problem 5 (20 points)
a) (8 points) 4 points for correctly using the supplied Maple
formula, and incorporating the needed integral of 1. 1 point each for
the first four terms of the Fourier sine series, simplified as
requested.
b) (12 points)
The left-hand graph (7 points)
In the graph of the partial
sum, I looked for the following qualitative behavior:
- Continuity on [0,Pi] (1 point).
- The value 0 at both 0 and Pi (2 points).
- Closeness (with "wiggling") to the line segment inside the
interval (2 points).
- Gibbs phenomena (overshoot) at both ends (2 points)
The right-hand graph (5 points)
This is supposed to be the graph of the sum of the whole Fourier sine
series. Here the behavior required was much simpler:
- Identical to the function except at the ends (3 points)
- 0 at both ends (1 point each)
Problem 6 (20 points)
a) (9 points) 2 points for separating correctly, with little or no
other correct work. 4 points for writing correct X(x)'s and
corresponding eigenvalues. 3 points for writing correct Y(y)'s. 2
more points for writing the product solution. If a product solution
was not written, I also looked in part b) for a correct product.
b) (11 points) 2 points for writing a "formal" sum of the
solutions gotten in a) with unknown coefficients. Then 3 points for
setting t=0, and recognizing that the result is a Fourier sine series
for the initial condition given, and using this information to compute
the general form of the coefficient in the series. 2 points for using
this coefficient in the series. 1 point each is earned for each of the
first four terms.
Problem 7 (12 points)
The point (2,3) was written as (Pi/2,3) on the actual exam. Neither the
students who took the exam nor the examiner noticed this! The picture,
as was intended, supplied the initial data.
a) (6 points) 2 points for connecting the dots at the ends. The
resulting graph should be a smooth function (2 points), below the
initial conditions (2 points). 1 point taken off if the graph is above
on one side.
b) (6 points) Again, 2 points for connecting the dots at the
ends. 2 points for drawing an increasing function. 2 points for
something that is straight or nearly straight.
Problem 8 (16 points)
a) (5 points) Write the D'Alembert solution for the PDE. No
boundary conditions are given, so use of Fourier series
techniques is not valid and earns 0 points. "Clarification" of f(x) by
finding formulas for its pieces is not necessary.
b) (5 points) Sketch two pieces of the graph, at half the amplitude
and the same width of the original shape, etc. If two separate bumps
are given, 2 points are earned. If they are the same shape as the
original profile, 1 more point is earned.
c) (4 points) The answer t=13.5 with some work shown earns the points.
The answer 14 earns 3 points, and the answer 15 earns 2 points.
d) (2 points) 1 point for the correct answer, and 1 point for some
reasonable explanation (it would be nice if the explanation included a
word like "shock").
Problem 9 (18 points)
a) (4 points) Write the correct answer.
b) (2 points) The results are the same (1 point) because cosine is
periodic (1 point).
c) (4 points) A useful and valid overestimate is desired. I looked for
15, with some explanation involving maximum values of sine and cosine.
d) (8 points) 6 points for some computation of the integral of
(u(x,y,t))2, somehow indicating how orthogonality is
used. 3 points off if no evidence is offered. The max value earns a
point, as does a value of t when this max value is attained.
Problem 10 (18 points)
2 points for recognizing that |x| is even so that the sine
coefficients are all 0. 10 points for computing the cosine
coefficients an for n>0, with all details. 2 points for
computing a0. 4 points for evaluating both sides of
Parseval's formula as much as possible (this includes evaluating the
integral).
Extra credit ( points)
23 students earned points by completing some part of the desired Maple graphs.
Course grades
I weighed each of the first two exams equally and the final exam twice
as much as either of these. I also constructed an "exam score" using
the Entrance Exam (20%), the QotD (30%), and textbook homework
(50%). I then averaged these four "exams" (with the final weighted
twice) to get one number, which I used to give a final grade. This
final letter grade was given using standards close to the conversion
table used three times above (from numerical grades to letter grades).
Maintained by
greenfie@math.rutgers.edu and last modified 12/23/2004.