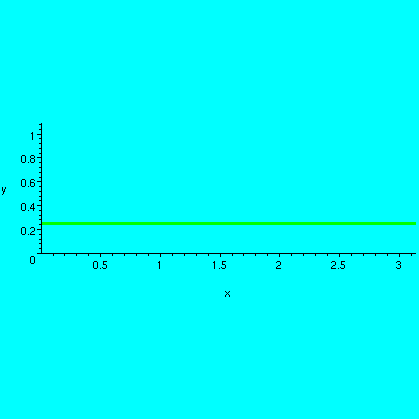Exam results for Math 421:02, spring 2004
The first exam
| | Problem
#1 |
Problem
#2 |
Problem
#3 |
Problem
#4 |
Problem
#5 |
Problem
#6 |
Problem
#7 |
Total |
|---|
| Max grade |
16 |
14 |
12 |
8 |
20 |
14 |
16 |
99 |
|---|
Min grade |
0 |
3 |
2 |
2 |
2 |
0 |
0 |
15 |
Mean grade |
6.59 |
10.09 |
8.16 |
7.06 |
12 |
10.5 |
10.44 |
64.84 |
Median grade |
5.5 |
11 |
12 |
8 |
11.5 |
12.5 |
8 |
65.5 |
32 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. The grade
distribution was remarkably bimodal. Please look at some comments about the results of
the exam. Here are approximate letter grade assignments for this
exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [85,100] | [80,84] | [70,79] |
[65,69] | [60,64] | [55,59] | [0,54] |
|---|
Discussion of the grading
Generally arithmetic errors will be penalized only minimally. The
instructor lost 2 points of this kind (in two separate errors:
problems 3 and 7!) when
he took the exam, so he would have gotten a 98. If, however, your
error makes the problem much simpler, more credit will be deducted.
Problem 1 (16 points)
a) (10 points) A routine computation using integration by parts. It
will help in part b) if you get the correct answer! Of course, a small
part of the purpose of part b) is to check your answer.
b) (6 points) Careful use of l'Hopital's rule is a major part of
justifying some of the computations of the "operational calculus".
Problem 2 (14 points)
This is a standard ODE problem which can be solved with various
techniques (several from 244, for example).
a) (10 points) 4 points for taking the Laplace transform of the
equation. 4 points for solving the partial fractions problem and
setting up the equation for the inverse Laplace transform. 2 points
for "reading off" the solution correctly from the tables.
b) (4 points) The points are for checking that the initial conditions
are satisfied. Of course, again this should help check your answer.
Problem 3 (12 points)
Straightforward application of one of the shifting theorems.
Problem 4 (8 points)
Easily done in at least two ways.
Problem 5 (20 points)
This problem is worth one-fifth of the exam. I don't think I could
solve this ODE easily without the Laplace transform!
a) (10 points) Here taking the Laplace transform earns 4 points, 4
points for "massaging" the Laplace transform (mostly another partial
fractions exercise), and 2 points for writing the inverse
transform.
b) (6 points: 2 points for each part) I am happy to accept an
unsimplified formula in the interval t>Pi.
c) (2 points) This is actually a very simple graph. You can get
enough information by evaluating at 0, Pi/2, Pi, and 3Pi/2. Even if
you didn't simplify previously, you should be "suspicious" about the
graph. Note also that "geometry" of the axes supplied was a hint.
d) (2 points) Maybe this is the theoretical part of the exam, although
maybe mechanical engineers should be concerned about shocks. That y(t)
is not differentiable at only one value of t is subtle to me.
Certainly the solution is differentiable everywhere except Pi/2
and Pi.
Problem 6 (14 points)
I'll give 6 points for taking the Laplace transform correctly and then
2 points for organizing the equations to be linear in X(s) and
Y(s). The remaining 6 points will be for finding an expression for
X(s). The intent of this problem was to get students thinking
about solving linear equations even when the darn coefficients are
"weird".
I have learned while grading this problem that I should avoid the use
of 5 in Laplace transform problems. 5 and s (the Laplace transform
variable) are difficult to distinguish in many students' handwriting.
Problem 7 (16 points)
Students should know and "manifest" what is needed to verify linear
dependence. Then the linear system has to be set up and solved. 4
points for knowing about linear dependence. 8 points for manipulating
the system correctly. 4 points for producing an explicit satisfactory
solution. I will give only 8 points for giving an explicit
linear combination when no process is shown.
The second exam
| | Problem
#1 |
Problem
#2 |
Problem
#3 |
Problem
#4 |
Problem
#5 |
Problem
#6 |
Problem
#7 |
Total |
|---|
| Max grade |
14 |
18 |
12 |
14 |
16 |
16 |
12 |
99 |
|---|
Min grade |
3 |
2 |
0 |
1 |
0 |
4 |
0 |
46 |
Mean grade |
9.23 |
15.2 |
9.63 |
6.53 |
13.03 |
11.33 |
7.57 |
72.53 |
Median grade |
10 |
18 |
11 |
5.5 |
15.5 |
12 |
8 |
71.5 |
30 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. Here are
approximate letter grade assignments for this exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [85,100] | [80,84] | [70,79] |
[65,69] | [60,64] | [55,59] | [0,54] |
|---|
Discussion of the grading
There were several ways to check the answers to most problems on this
exam, so even arithmetic could be checked easily. The exam was a bit
harder to grade than I had expected. Most people seemed to do most
problems correctly, but the errors that occurred were rather
idiosyncratic, and I tried diligently to grade consistently. This
meant, at times, regrading and checking many papers so that similar
errors received similar penalities.
Problem 1 (12 points)
6 points for each part. I read what was written. I
required that what was written be responsive to what was asked. Thus a
discussion of span or subspace was irrelevant in part a)
if linear combination was not correctly defined, and, once that
was correctly defined, such discussions were superfluous.
I took 2 points off in part b) if the definition used AX= X and did not specify that X must be
non-zero. This specification is important. If a definition
using the equation det(A-
X and did not specify that X must be
non-zero. This specification is important. If a definition
using the equation det(A- In)=0 was given, there was no such risk.
In)=0 was given, there was no such risk.
Problem 2 (18 points)
a) 2 points for correctly computing the characteristic polynomial.
b) 5 points for the student's finding the correct roots of the
characteristic polynomial (1 point) and then finding two correct
eigenvectors. If two correct eigenvectors were displayed I gave credit
for a basis. If an answer labeled basis was incorrect even
though two correct eigenvectors were given, I took off 1 point. You
can't get credit for the eigenvectors unless the correct eigenvalues
are found, because with an incorrect eigenvalue, you can't find
non-trivial solutions to AX= X. Therefore several mistakes had to be made,
and that's too much for partial credit.
X. Therefore several mistakes had to be made,
and that's too much for partial credit.
c) 2 points: only correct answers received credit here, because
incorrect answers resulted from several previous mistakes.
d) 5 points: I gave credit for correctly finding an inverse for the
student's P. 1 point for the answer alone.
e) 2 points for the correct product of A and the student's P.
f) 2 points for the correct product and answer. I deducted 1 point if
the student gave the correct answer for the product of the student's
P-1 and the student's Z but the answer was not
diagonal. There was enough time to check and recheck answers on the
exam and numerous ways to discover errors.
Problem 3 (12 points)
Many people got this right. "A matrix is invertible if its determinant
is not 0." Very few students attempted to do row operations to
simplify the matrix before evaluating the determinant which
significantly reduces the work.
I took off 1 point if the strategy and the evaluation
proceeded well, except for a "silly" arithmetic error. And another 1
point if I spotted a distinctly different error. I took off more if I
thought there was an error more serious than simple arithmetic.
Some students attempted to find A-1 and to discover which
values of x make this possible. I consider this sa difficult
strategy. Attempting to do this would earn 8 points (no one completed
it successfully!). I took points off from there.
There is probably an optimal (?) sequence of row/column operations
applied to the original matrix which would make the answer "clear" but
I don't know it. Again, one or two row/comn operations make
evaluating the determinant much easier. Very few students did this!
Problem 4 (14 points)
Student performance implies that this was the most difficult problem
on the exam. I wanted correct answers and supporting reasoning in each
part of the problem. I tried diligently to grade the problem
consistently, so I actually had to regrade some students' work several
times.
Part a) will get 6 points: 3 for remarking that there are many
solutions to AX=0 (that X=0 is a solution will earn only 1 point), and
3 for analyzing the dimension of S. Again, especially in this problem,
correct assertions will earn minimmal credit if unsupported by
verfication. Somewhere there should be a mention that the two
solutions to AX=0 supplied are linearly independent.
Part b) is worth 4 points. I seem not to have taught effectively the
content of the following sentence. The larger the rank, the
smaller the dimension of S will be, and the larger the
dimension of S, the smaller the rank will be.
Part c) is again worth 4 points. If the rank is not 5, then there are
Y's for which AX=Y has no solution.
Problem 5 (16 points)
Here I looked for correct implementation of the method which takes
advantage of diagonalization:
A5=PD5-1 where P is the matrix of
eigenvectors written as column vectors, and D is the corresponding
diagonal matrix of eigenvalues. A direct computation of A5
earned little.
The correct outline will earn 8 of the 16 points. One error which lost
3 points was interchanging the roles of P and P-1 in the
formula for A-1.
Implementation counts for the other 8 points. Creating a candidate for
D is not difficult, and checking a candidate for P-1, given
the information in the note, can also be done. The wrong
P-1 lost 3 points. Several people took advantage of the
symmetry of A to get a P which was orthogonal (by normalizing the
given eigenvectors) and then computation of P-1 was easy:
P-1=Pt. This was nice. A wrong answer loses at
least a point.
Problem 6 (16 points)
a) 8 points, which must include a specific citation. Useful birds here
are Goldfinch and Nuthatch, and their rank (3) should be
used. Inadequate verification will earn only 4 of 8 points.
b) This was worth 8 points. Here I believe there's only one relevant
RREF, Nuthatch. A citation would include verifying that the
ocordinates of the student's candidate do not satisfy the
compatibility/consistency conditions in the last two rows of the RREF
of Nuthatch. I'll only give 4 of 8 points for inadequate
verification. The suggestion that a vector in R3 is an
answer cannot be correct!
Problem 7 (12 points)
I agree: knowledge of what a polynomial of degree 3 is is
necessary in this problem. I do not apologize.
I do regret not making the last column of the augmented matrices in
the BIRD list have entries written as y1, y2,
etc. I used A+Bx+Cx2+Dx3 when I did the
problem, but I still got very confused, with A and a, B and b,
etc.
A common error seemed to be writing the coefficients in reverse
order. I took off 2 points for this, since the error can be detected
(although not with p(1)=2, as several students pointed out).
Otherwise,
4 points for realizing that the "general" cubic polynomial gives some
linear equations with the four conditions specified, 4 points for
translating the 4 equations correctly with a reference to
Helicopter, and 4 more points for solving the equations correctly.
The third exam
| | Problem
#1 |
Problem
#2 |
Problem
#3 |
Problem
#4 |
Problem
#5 |
Problem
#6 |
Problem
#7 |
Total |
|---|
| Max grade |
10 |
12 |
16 |
16 |
14 |
16 |
16 |
96 |
|---|
Min grade |
2 |
2 |
4 |
0 |
0 |
0 |
0 |
23 |
Mean grade |
7.76 |
6.69 |
13.03 |
8.21 |
7.48 |
9.66 |
7.34 |
60.17 |
Median grade |
9 |
7 |
14 |
8 |
9 |
11 |
6 |
62 |
29 students took this exam. Numerical grades will be retained for use
in computing the final letter grade in the course. Overall, the grades
were a bit lower than I had hoped, perhaps because this exam came so
late in the exam period and people were tired. Here are approximate
letter grade assignments for this exam:
Letter
equivalent | A | B+ | B | C+ |
C | D | F |
|---|
| Range | [85,100] | [80,84] | [70,79] |
[65,69] | [60,64] | [55,59] | [0,54] |
|---|
Discussion of the grading
Problem 1. (10 points)
5 points for each part. I expected this to be totally
straightforward. No integral computations were needed, only
recognition of various orthogonality relationships (on the formula
sheet, if the orthogonality had not "installed" mentally).
Problem 2. (12 points)
This problem's results were disappointing. Perhaps I should have
written the question differently. I expected the following mental
connections: the Fourier sine series converges to the odd extension of
f(x), and the Fourier cosine series converges to the even extension of
f(x). With these observations in mind, the problem is also
straightforward, requiring practically no computation.
Each correct answer in part a) received 1 point. Correct answers in
parts b) and c) were each awarded 2 points.
Problem 3. (16 points)
Certainly the result of a) is needed in b), and the result of b) is
needed in c). If an error was made somewhere in the process, I tried
to "read with" the student's work so that ideally the answer would
lose an appropriate amount of credit only for the initial error (if
the result did not simplify the problem ridiculously).
a) 5 points for all aspects of a). The diversity of errors was
interesting. I did not subtract for an omitted "+C" although it was an
indefinite integral!
b) (3 points) I wanted people to recognize with appropriate
evaluations cos(nPi) and sin(nPi). We have done these over and over
again in this course.
c) (4 points) Exact values were requested.
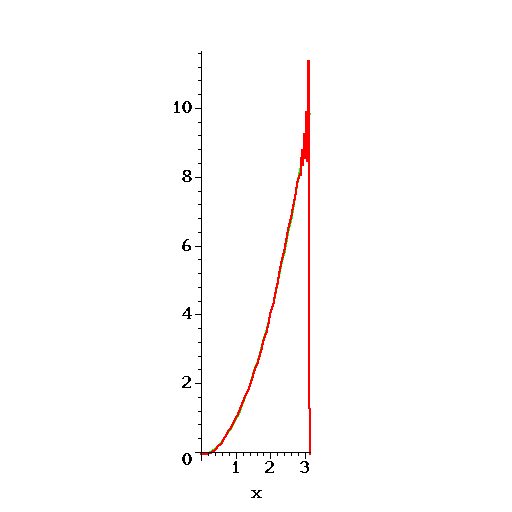 d) (4 points) Here recognition of g(x) as a (high) partial sum of the
sine series for f(x) (that is, the Fourier series of the odd extension
of f(x) to [-Pi,Pi]) is needed. To the right is a Maple graph of
g(x), which entirely obscures f(x) on this scale. Note that the
drawing jumps down to 0 at x=Pi and has a Gibbs overshoot compared to
x2 near and to the left of x=Pi. I forgot the jump all the
way down to 0, so I would have lost a point in my solution to this
problem.
d) (4 points) Here recognition of g(x) as a (high) partial sum of the
sine series for f(x) (that is, the Fourier series of the odd extension
of f(x) to [-Pi,Pi]) is needed. To the right is a Maple graph of
g(x), which entirely obscures f(x) on this scale. Note that the
drawing jumps down to 0 at x=Pi and has a Gibbs overshoot compared to
x2 near and to the left of x=Pi. I forgot the jump all the
way down to 0, so I would have lost a point in my solution to this
problem.
Problem 4. (16 points)
Students should be acquainted with e-x2. This
function is very important in statistics, and presumably your labs
have told you how to analyze data statistically. This is the
"Gaussian" bump. The function y(x,0) specified in the problem is 4
times as high, and shifted to center at 3.
a) (4 points) I hoped you would use the D'Alembert solution. You must
state what f is, since there is no f(x) specified in the statement of
the problem. I deducted 2 points if no f(x) was specified here, but I
would give a point back if I could reasonably deduce later in the
problem that you "meant" the correct f(x).
I graded b) (4 points) and c) (4 points) together. 2 points were
deducted for each error: bumps not symmetric, bumps not rounded, bumps
not the proper amplitude, and bumps not properly centered. Also each
of the four bumps should have more or less the same shape (no
diffusion).
d) (4 points) If an incorrect answer alone was given, the grade was 0
for this part. I gave part credit even for an incorrect answer if some
correct aspect of the process was shown.
Problem 5. (14 points)
a) (6 points) A correct answer alone would get full credit, since
no integration computation is necessary. Writing the formula from the
formula sheet and doing no further work earns no credit. If
that approach is taken, computations to get the answer must be
performed.
b) (4 points) The graph must be the same as what is shown. Some
explanation depending on periodicity or on cos((even integer)Pi)=1
must be given.
c) (4 points) Please note that any explanation based on the
graph displayed must be incorrect. Consider the following much simpler
example: y(x,t)=A sin(x)cos(t) is a solution of
ytt=yxx for any constant A. A graph of y(x,Pi/2)
will show a horizontal line with 0 displacement. The maximum
displacemen t is A, which I can choose to be any number. Therefore you
can't make deductions based on the displacement alone at any
specific time. Perhaps the problem statement should have read, "Use
your answer to a) to estimate the maximum displacement at any time
..." Answers I considered correct used information about the max of
|sine| and |cosine| and the triangle inequality, even if only
implicitly.
Problem 6. (16 points)
a) (5 points) The formula to be used is on the formula sheet (just
copying this does not earn credit!). One computation is for
c0. Then the other coefficients must be gotten through a
simple integration.
b) (5 points) The trig functions should be evaluated where possible, and
the first 5 non-zero terms written out.
Below are Maple pictures of the 250th (!) partial
sums at t=0 and t=1/100 and t=100. The first picture shows the usual
Gibbs stuff. The second picture is nice and smooth, and the third
picture seems to be like the graph of a constant function.
| Graph of the partial sums up to the
term involving cos(250x) |
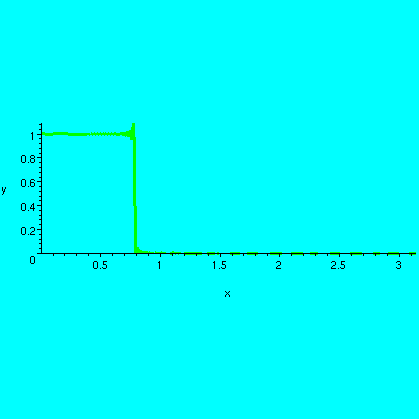 |
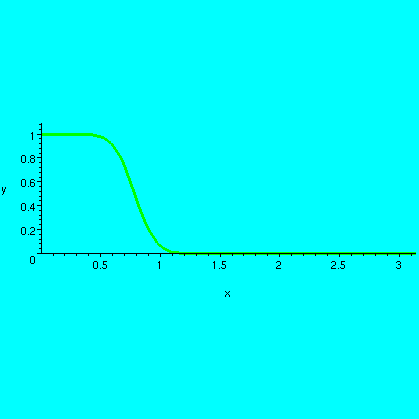 |
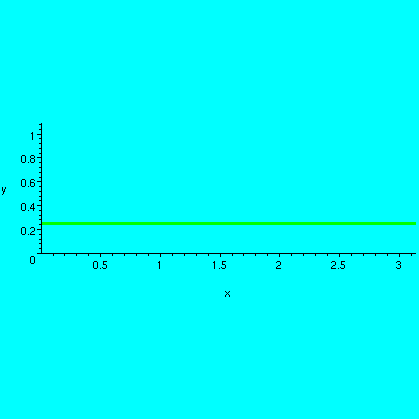 |
| t=0 | t=1/100 | t=100 |
|---|
c) (4 points) The graph should be rounded and smooth, decreasing and
less than the original graph, and positive. The corners should be
replaced by curves.
d) (2 points) A horizontal line at height 1/4.
Problem 7. (16 points)
a) (6 points) 3 points for writing T'/T=(X''/X)+1, and then 3 more
points for successfully separating (the constants in the two ODE's
must be linked).
b) (7 points) The deductions X(0)=0 and X(Pi)=0 earn 2 points. Solving
the boundary value problem for X(x) earns 3 points, and then solving
for T(t) earns 2 points. At times the work for this part and part a)
were intertwined and I tried to recognize this with appropriate
grades.
c) (3 points) The correct infinite series with coefficients earns 3
points.
Additional exam 1 and exam 2 problems
The additional Laplace transform and linear algebra problems
were graded similar to problems of the type in exams 1 and 2. I did
not compile statistics on the additional problems.
Adjusting the grades in exam 1 and exam 2
I used the following Maple function to "adjust" grades in the
first two exams. Here x was the old grade, and y was the additional
grade achieved on the parts given out during the final.
G:=(x,y)->evalf(min(100,x+(y*(60-max(x-50,0)+max(x-90,0))/60)));
The "outside" min guarantees that no one can get better than 100. The
old grade, x, gets an addition proportional to y. The constant of
proportionality is 1 for grades 50 or below, and is 1/3 for grades 90
or above. The constant of proportionality changes linearly between
these extremes. Here are some sample evaluations. Remember, x is the
old grade, in the interval [0,100] and y is the additional grade, in
the interval [0,24].
G(40,12);
52.
G(60,12);
70.
G(80,12);
86.
G(90,12);
94.
I used the adjusted grades in place of the initial grades when giving
course grades.
Course grades
I weighed each of the three exams equally (using the adjusted grades if necessary). I also
constructed an "exam score" using the Entrance Exam (20%), the QotD
(40%), and textbook homework (40%). I then averaged these four "exams"
to get one number, which I used to give a final grade. This final
letter grade was given using standards close to the conversion table
used three times above (from numerical grades to letter grades).
Maintained by
greenfie@math.rutgers.edu and last modified 3/14/2004.
 d) (4 points) Here recognition of g(x) as a (high) partial sum of the
sine series for f(x) (that is, the Fourier series of the odd extension
of f(x) to [-Pi,Pi]) is needed. To the right is a Maple graph of
g(x), which entirely obscures f(x) on this scale. Note that the
drawing jumps down to 0 at x=Pi and has a Gibbs overshoot compared to
x2 near and to the left of x=Pi. I forgot the jump all the
way down to 0, so I would have lost a point in my solution to this
problem.
d) (4 points) Here recognition of g(x) as a (high) partial sum of the
sine series for f(x) (that is, the Fourier series of the odd extension
of f(x) to [-Pi,Pi]) is needed. To the right is a Maple graph of
g(x), which entirely obscures f(x) on this scale. Note that the
drawing jumps down to 0 at x=Pi and has a Gibbs overshoot compared to
x2 near and to the left of x=Pi. I forgot the jump all the
way down to 0, so I would have lost a point in my solution to this
problem.