Klein's Quartic

'

Klein Quartic Talk
Greg Egan's Equation Page
Greg Egan's Curve Page
John Baez's Page

Resources

The Eightfold Way: The Beauty of Klein’s Quartic Curve, edited Silvio Levy
Noam D. Elkies. The Klein Quartic in Number Theory. In The Eightfold Way, vol 35. MSRI Publications, 1998.

Modular Curve X(7)

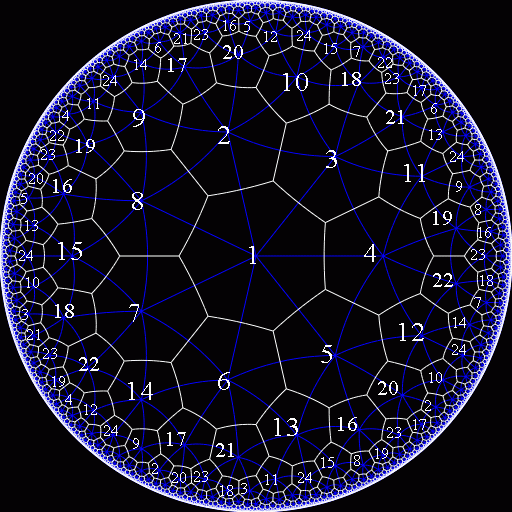
Symmetry Group

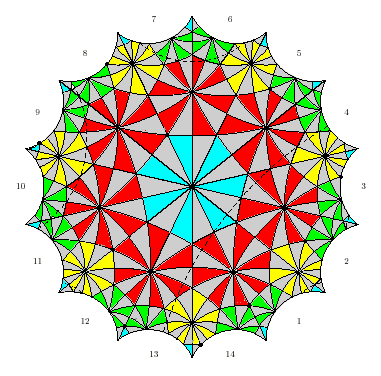
The symmetry group (orientation preserving automorphsims) is of size 168. It is isomorphic to G ≅ PSL 2 ⁡ ( F 7 ) ≅ G L 3 ( F 2 ) The character table is given by c 1 A 2 A 3 A 4 A 7 A 7 B # c 1 21 56 42 24 24 χ 1 1 1 1 1 1 1 χ 3 3 − 1 0 1 α α ¯ χ ¯ 3 3 − 1 0 1 α ¯ α χ 6 6 2 0 0 − 1 − 1 χ 7 7 − 1 1 − 1 0 0 χ 8 8 0 − 1 0 1 1

Klein Model

The ring of invariant polynomial of the three dimensional representation is generated by Φ 4 := X 3 Y + Y 3 Z + Z 3 X Φ 6 := − 1 54 H ( Φ 4 ) = X Y 5 + Y Z 5 + Z X 5 − 5 X 2 Y 2 Z 2 Φ 14 = 1 9 | ∂ 2 Φ 4 / ∂ X 2 ∂ 2 Φ 4 / ∂ X ∂ Y ∂ 2 Φ 4 / ∂ X ∂ Z ∂ Φ 6 / ∂ X ∂ 2 Φ 4 / ∂ Y ∂ X ∂ 2 Φ 4 / ∂ Y 2 ∂ 2 Φ 4 / ∂ Y ∂ Z ∂ Φ 6 / ∂ Y ∂ 2 Φ 4 / ∂ Z ∂ X ∂ 2 Φ 4 / ∂ Z ∂ Y ∂ 2 Φ 4 / ∂ Z 2 ∂ Φ 6 / ∂ Z ∂ Φ 6 / ∂ X ∂ Φ 6 / ∂ Y ∂ Φ 6 / ∂ Z 0 | Φ 21 = ∂ ( Φ 4 , Φ 6 , Φ 14 ) 14 ∂ ( X , Y , Z ) = 1 14 | ∂ Φ 4 / ∂ X ∂ Φ 4 / ∂ Y ∂ Φ 4 / ∂ Z ∂ Φ 6 / ∂ X ∂ Φ 6 / ∂ Y ∂ Φ 6 / ∂ Z ∂ Φ 14 / ∂ X ∂ Φ 14 / ∂ Y ∂ Φ 14 / ∂ Z |

Ramanujan Theta Series on X(7)

The map from the modular curve X(7) to the Klein Quartic is given by (a: b: c) where a ( z ) = − ∑ n ∈ Z ( − 1 ) n q ( 14 n + 5 ) 2 / 56 b ( z ) = ∑ n ∈ Z ( − 1 ) n q ( 14 n + 3 ) 2 / 56 c ( z ) = ∑ n ∈ Z ( − 1 ) n q ( 14 n + 1 ) 2 / 56

This page has been accessed at least several times since January 2019.

suryatejagavva