
Like most before me, my introduction to physics was classical mechanics. Before this I was very depressed, had no long term goals or ambitions, and was consistently failing my primary school classes. In my final year of high school I took two classes that changed my trajectory: calculus and classical mechanics. The mechanics class was not meant for an audience with calculus knowledge. This gave me the opportunity to form connections between the subjects on my own. The feeling of "discovery" that came from my studies started to bring me a joy I had not known. It gave me the motivation to pursue self-improvement not for the sake of others, but for my own. For this reason, classical mechanics will always occupy a special place in my heart.

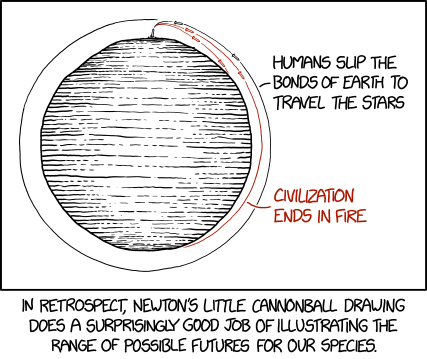
The theory of classical mechanics concerns itself with the motion of massive particles, rigid bodies, and fluids. The motion of massive objects is dictated by Newton's second law, which states that the acceleration of an object is the sum of the forces acting on the object divided by the object's mass. If you know all the forces acting on an object, you can use this law to solve for the object's trajectory. This has proven a very useful tool for a veriety of purposes, most notably in the slaughter of humans by means of cannonball or rocket. I would like to note that knowledge of the forces is not sufficient to determine the object's trajectory. One also needs to know the initial position and velocity of the object. Those who have a thrown a football knows that the trajectory will depend heavily on the initial height(on the ground vs from a roof) and with what initial velocity(upwards or downwards, 1mph or 200mph) it is thrown with. The belief that evolutions of isolated systems are uniquely derivable from some laws of motion along with such "initial conditions" is called determinism.
Vladamir Alexandrovitch Fock
These trajectories can get pretty complicated, but physicists were quick to realize that certain quantities are left unchanged as the system evolved. The sum of the "kinetic energies" of the objects (which are directly related to how fast the objects are going) plus the "potential energy" of the system is always preserved. So, if one wants to speed an object up then either the potential energy needs to decrease or another object must sacrifice some of its kinetic energy. In some situations this puts a "cap" on the maximum distance that an object can travel.
Physicists now view kinetic energy as a physical property of massive objects. It is just as fundamental as position, velocity, and mass. Potential energy is not given the same treatment. It does not "live" anywhere in physical space, in contrast with kinetic energy which exists in physical space wherever moving matter is present. The quantity associated to potential energy cannot be fundamental either, since adding any number to the potential energy of a system does not alter the system's dynamics. For these reasons potential energy is viewed as a mathematical tool that one uses to study the evolution of physical quantities in Newtonian mechanics. It is not itself a physical quantity.
The transition from Newtonian mechanics to Relativistic mechanics was historically pretty fucking crazy, and the theory of Maxwell that ultimately led to this transition gave rise to a number of other revolutionary ideas. Most notable was the concept that "electromagnetic forces", or rather the "things" that gave rise to those forces, exist in physical space in the same way that matter does. Compare this with Newtonian theory, where the only things that really exist is matter, and we describe the motion of that matter using mathematical tools like potential energy.

James Clerk Maxwell
In modern electromagnetic theory there are two forms of energy-momentum that can manifest in space. The first is matter, whose energy-momentum is distributed wherever matter is present. The second form is electromagnetic, which is distributed wherever electromagnetic fields are present. Like Newton's law of motion for matter, Maxwell's equations play the role of governing the motion of electromagnetic fields. Also like Newton's law, Maxwell's equations imply a conservation law for electromagnetic energy-momentum. But there is no energy term that is considered "potential", all matter and field energies are physical.
Maxwell's equations state that charged matter effects the motion of electromagnetic fields. Due to this interaction, electromagnetic energy-momentum is not conserved in the presence of charged matter. However, energy-momentum of the field-matter system must be conserved, so any increase/decrease of the field energy-momentum must be balanced by a decrease/increase of the matter's energy-momentum. We are led to the conclussion that there must be some electromagnetic force acting on the charged matter, defined precisely so that the rate of energy-momentum change for matter is equal and opposite to the rate of energy-momentum change of the field. For charged matter that is smoothly distributed in space, a simple computation shows that this force must be the Lorentz force law.

To summarize: Maxwell's theory states that there are electromagnetic fields in space which evolve according to Maxwell's equations. If there is charged matter, then the evolution of this charged matter is dictated by the Lorentz force law, which can be derived from Maxwell's equation along with the principle of energy-momentum conservation. Charged matter does not directly interact with other matter, in constast with Newtonian theory where matter directly interacts with other matter via forces. Instead charged matter interacts with the electromagnetic fields, and the fields then act on the charged matter via the Lorentz force.
Despite my love of Newtonian Mechanics, I was a very proor student of Electromagnetism. This was not the fault of my teachers. My academic grip was slipping when I entered the course, and I quickly fell into a cycle of not meeting my own standards, resenting myself for it, then losing the motivation to keep trying.
In hindsight, my conceptual issues with E&M came from three factors. The first is that my mathematical competence appears to only apply to problems related to the dynamics of some physical quantities. I can't do combinatorics or graph theory, those who know me know I can't visually count past four. Abstract algebra almost made me quit grad school. Even in the realm of PDE theory, I highly dislike studying elliptic PDEs unless I can form a mental relationship between the elliptic PDE and some moving physical system. Going back to my old E&M textbook, I see that about 2/3rd of the course focused on electrostatics and magnetostatics.
Also relevant was insufficient pre-requisite knowledge of multivariable calculus. Divergence, Curl, line integrals, volume integrals, Stokes theorem, Gauss's theorem, I had to learn about all of these along the way. I was not alone in this. Plenty of my classmates were learning math on the fly, and the advice they gave me when struggling was to rely on my physical intuition. This bring us to the final factor: I didn't understand what the fuck was going on.
Sheldon Goldstein, 2020
Take energy. In Newtonian mechanics, energy appears in two ways: kinetic and potential. Kinetic is the "real" energy, potential is a mathematical tool we use. In electromagnetism, there's still kinetic and potential energy for the particles (except not really because they're relativistic), but now there's also an electric potential, electric potential energy (which is charge times the electric potential), electric kinetic energy (the pure kinetic energy of the electric field), and magnetic vector potential, and of course the magnetic kinetic energy (the pure kinetic energy of the magnetic field). Which of these are a "real" energy, and which are just mathematical tools? All were presented to me as equally physical, but they all had different circumstances in which they were applicable. The theory felt very fragmented to me, and that's hardly surprising given that the development of electromagnetism was very fragmented. There were many researchers contributing to the subject from a variety of perspectives, and I found myself unable to distinguish the various frameworks at play.

It was not until much later that I relearned electromagnetism from the ground up. Instead of struggling to piece together fragmented bits of knowledge from different physical phenomenon to obtain a unified picture of the universe, I started with a unified theory (Maxwell's equations) which I could unravel and apply to different physical situations. Some would say that piecing together fragmented data from different physical phenomena is a crucial skill for any physicist to have. It is historically how many physical equations, like Maxwell's equations, were discovered in the first place. So, am I a bad physicist for not having this talent? I used to think so, which is why I changed my major from physics to mathematics. In hindsight I see that there are many ways one can contribute to the physics community: there is more than discovering. I also now know that the game has changed since Maxwell's time: many physical theories from the past century, like General Relativity, was derived from simple physical concepts combined with advanced mathematical knowledge.
It came as a great shock when I learned there is a fundamental flaw in Maxwell's theory, an inconsistency which has not been resolved for over one hundred years. It came as a greater shock when I learned that the problem could be traced back to a simple mathematical fact known to even primary school students: we can't divide by zero. It is because of this fact that Maxwell's electrodynamics are not well-defined in the presence of point particle charges. The theory states that the force acting on these point charges depends on the electromagnetic fields evaluated at the point charge positions, but it is precisely at these precisions that the fields are not defined! Point charges generate electromagnetic fields that depends on one over the distance squared from the point charge position. So, as you approach the point charge that distance becomes small, and the electromagnetic fields are very large. When you try to evaluate the field literally at the point charge position, you get 1/0 which is ill-defined. In college courses we are taught to ignore the divergent term, they say a point charge's field can't act back on the point charge, it only acts on other charges. But this was a made up rule, nothing in Maxwell's theory says this.
Detlef Durr and Sergio Albeverio, late 1980s
In fact, Maxwell's theory implies that there must be a self-action for point charges! This is because of radiation-reaction. When charge distributions move, they radiate energy and momentum through the electromagnetic fields that they source. For the total energy-momentum of the system to be conserved, the field sourced by the point charges must act back on the point charge. Unfortunately, there are divergent quantities that always seem to appear when trying to calculate this re-action term. Although the fundamental axioms of Maxwell's theory are not consistent (for point charges), the results of the theory speak for themselves.

Todd Howard
Hans Reichenbach
In the mid 1930s physicists realized that they could no longer ignore the infinities appearing in Maxwell's theory. It was mucking up their groundbreaking work in QED, so Dirac went back and tried to resolve the inconsistency. His main techniques were an infinite bare-mass renormalization that is mathematically ill-defined, along with an ad-hoc averaging of the fields in a neighborhood of the charge. The equation of motion he derived was third order in time for the particle’s position, and consequently nearly all solutions exhibit a run-away effect. It is generally expected that these equations of motion will agree with empirical data in regimes where radiation-reaction is weak in comparison to external fields. However, these equations and their derivations do not constitute a consistent theory of point charges evolving with their sourced fields.
Shadi Tahvildar-Zadeh, 2020
The state of affairs went largely unchanged until a breakthrough occured in 2019. Michael Kiessling showed that postulating energy-momentum conservation of the field-particle system yields a unique and admissible force law, provided integrability conditions on the electromagnetic field. Electromagnetic fields satisfying Maxwell's laws in the presence of point charges do not satisfy these integrability conditions because of the divergences. But if one "modifies" the Maxwell's laws slightly, then Kiessling showed that you can get a well-defined force law.
John Herschel
Now that we have a well-defined evolution law for the electromagnetic fields and their point charge sources, our goal is to study the "joint evolution problem" for the system: Given an initial position and velocity for the point charges, along with some initial data for the field, is there a unique joint evolution of the particles and field?

Shadi Tahvildar-Zadeh, Samuel Leigh, and I were able to write down a simple closed form formula for the self-force which a charged 1-d particle "exerts on itself". Surprisingly, we did so without needing any "modification" for the field equation because the singularities sourced by point charges in one space dimension are milder than those in three space dimensions. In a follow-up paper, I studied the joint evolution problem for point charges and their sourced field. Strangely, I found that the evolution exists if and only if the initial data for the particles satisfy a "compatibility condition" with the initial data for the field. This compability condition implies that almost all physical properties of the point particles: namely their initial position, velocity, and even charge are uniquely determined from the initial field data, but the initial field data is allowed much more freedom.
Norbert Wiener
This result reinforces the view point of Weyl’s “agens theory” of matter, as it instructs us to think of the field as the fundamental object existing on space-time, with the point charge trajectories simply being the locus of singularities of the field. It is very likely that if we study the joint evolution problem for charged particles acting as singularities in both a field and a space-time(like in General Relativity), then the mass of point charges will probably be uniquely determined by the initial data for the space-time. At this point every physical property of the point charges will be uniquely determined by the surrounding fields and space-time, so we should regard these as the fundamental objects.
Gif of moving charged particle