u(0,t)=0 and u(Pi,t)=0
sin(n x)
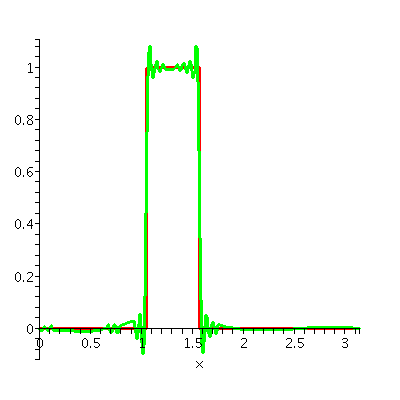
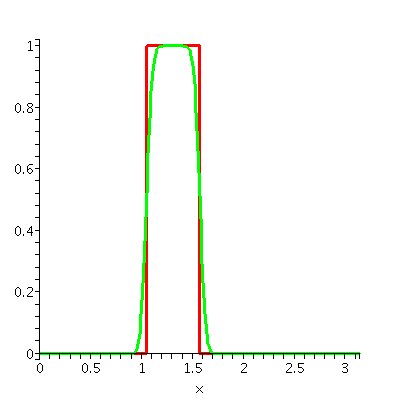
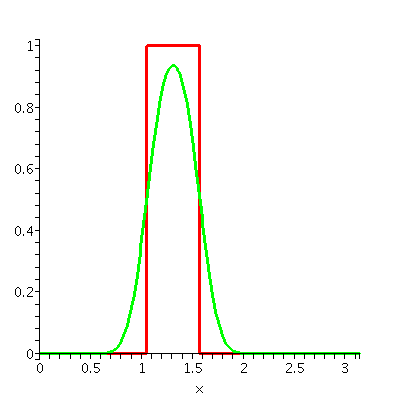
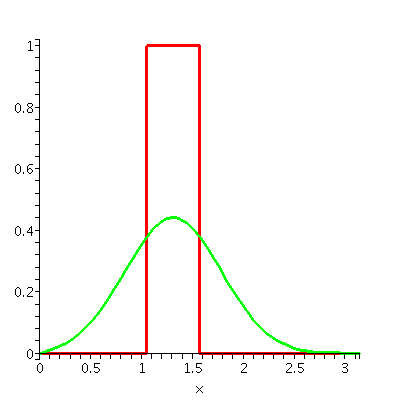


Flux 0 at 0 and Pi
ux(0,t)=0 and ux(Pi,t)=0
cos(n x)
For n=0, this is the function 1.
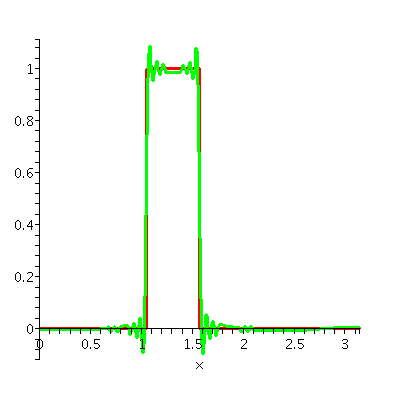
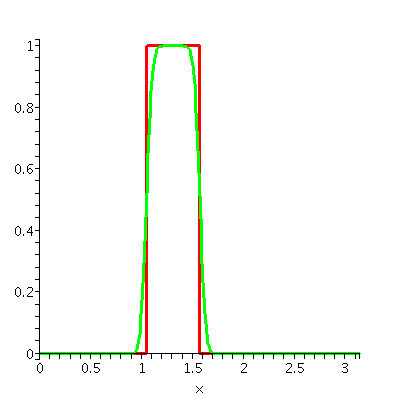
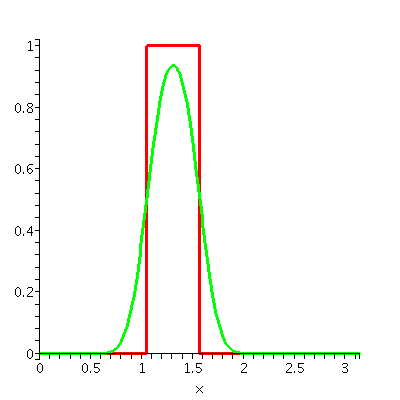

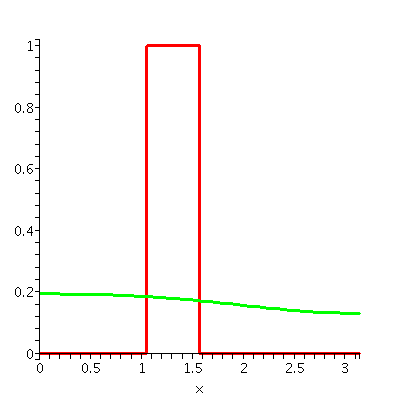
u(0,t)=0 and ux(Pi,t)=0
(n at least 0)
sin((1/2)(2n+1)x)

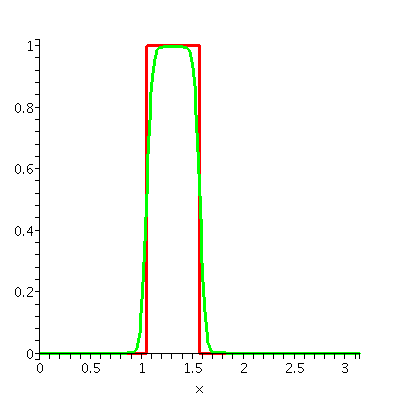
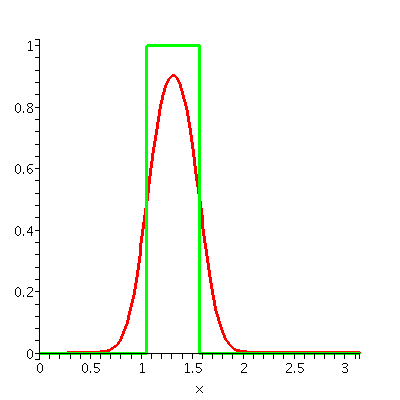
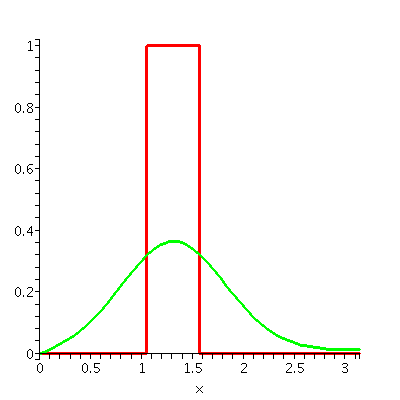
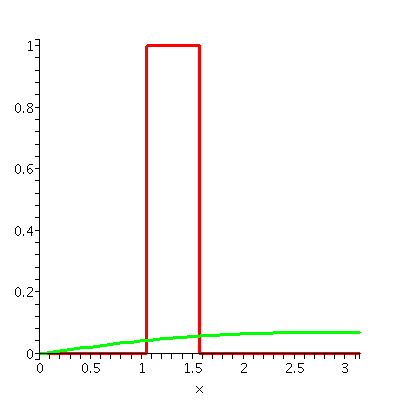
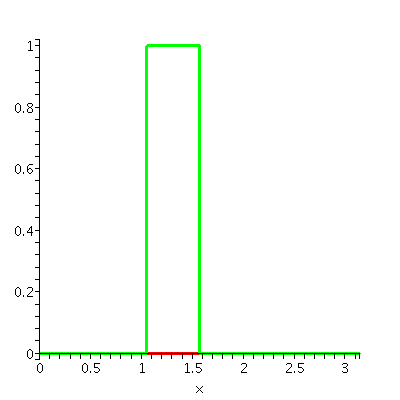
The pictures in each column are not the same! Things differ in subtle ways. You need to look closely. Material similar to the first example was handed out in class. All of the pictures used similar Maple commands, also available in the class handout.
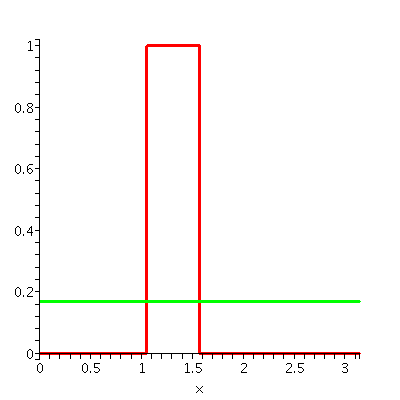
| The boundary value problem | Its eigenvalues and associated eigenfunctions | Graph of the 100th partial sum of the Fourier-like series associated with this eigenfunction expansion | Behavior at t=.001 | Behavior at t=.01 | Behavior at t=.1 | Behavior at t=1 | Behavior at t=10 |
|---|---|---|---|---|---|---|---|
| Temperature 0
at 0 and Pi u(0,t)=0 and u(Pi,t)=0 |
Positive integers, n. sin(n x) |
 |
 |
 |
 |
 |
 |
| When t gets large, the heat oozes out the ends, and the temperature drops to 0 in the whole interval. | |||||||
| The boundary value problem | Its eigenvalues and associated eigenfunctions | Graph of the 100th partial sum of the Fourier-like series associated with this eigenfunction expansion | Behavior at t=.001 | Behavior at t=.01 | Behavior at t=.1 | Behavior at t=1 | Behavior at t=10 |
|
Insulated ends Flux 0 at 0 and Pi ux(0,t)=0 and ux(Pi,t)=0 |
Nonnegative integers, n. cos(n x) For n=0, this is the function 1. |
 |
 |
 |
 |
 |
 |
| When t gets large, the heat tends to even out over the whole interval, and the temperature approaches a constant (determined by the total area). | |||||||
| The boundary value problem | Its eigenvalues and associated eigenfunctions | Graph of the 100th partial sum of the Fourier-like series associated with this eigenfunction expansion | Behavior at t=.001 | Behavior at t=.01 | Behavior at t=.1 | Behavior at t=1 | Behavior at t=10 |
| Temperature 0 at 0 and
insulated end at Pi u(0,t)=0 and ux(Pi,t)=0 |
Half of odd positive integers, (1/2)(2n+1). (n at least 0) sin((1/2)(2n+1)x) |
 |
 |
 |
 |
 |
 | When t gets large, the heat oozes out the left end, while the temperature curve always has a horizontal tangent at the right end because there is no heat flow there. The temperature eventually drops to 0 throughout the interval. |
Maintained by greenfie@math.rutgers.edu and last modified 8/27/2004.