$maple
|\^/| Maple 10 (IBM INTEL LINUX)
._|\| |/|_. Copyright (c) Maplesoft, a division of Waterloo Maple
Inc. 2005
\ MAPLE / All rights reserved. Maple is a trademark of
<____ ____> Waterloo Maple Inc.
| Type ? for help.
> evalf((10^10)^(10^(-10)),20);
1.0000000023025850956
Was that difficult?
Problem 2
I was asked by several people if the sketch could be gotten
from the output of a calculator. Sure, I replied, sure. But think about it. Maybe, just maybe, your
calculator is misleading you. A calculator computes
some values and then, with the help of your eyes and brain, connects
the dots.
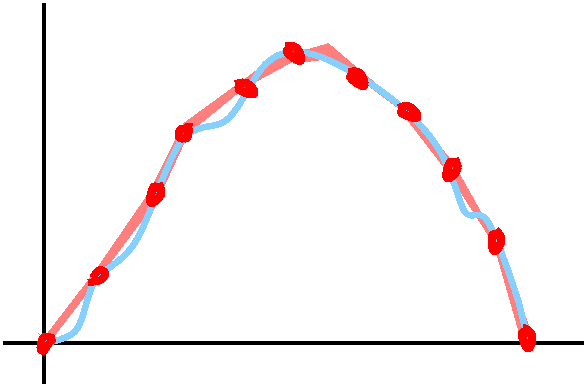
Look at the picture below. Maybe the true curve is in light blue and
somewhat complicated. The calculator has computed the blobby red dots
as sample points. Then you, together with the calculator,
believe that the curve is the light red object. What's correct?
Therefore you, using calculus, should be prepared to
justify the shape of any curve you draw: yes, the calculator
certainly may provide supporting evidence, but you need to
justify your assertions (using calculus this won't be very
difficult!). You should learn to think about the output of
"intelligent" machines.

|
Maintained by greenfie@math.rutgers.edu and last modified 9/16/2005.