Mapmaking and its History
Dan Sullivan
History of Mathematics
Rutgers, Spring 2000
A map is a small-scale, flat-surface representation of some portion of the surface of the earth, whose purpose of a map is to express graphically the relations of points and features on the earth's surface to each other. Cartography is defined as the art of making maps. As the earth is roughly spherical, this involves the systematic representation of all or part of a round body on a flat surface; such a representation is called a map projection.
The contemporary science of mapmaking developed very slowly at first, because the exploration of the earth as a whole is itself a relatively recent historical development. "Although the true shape of the earth was in doubt for centuries, many scholars were convinced the earth was spherical" (Snyder 1). This insight was already reached in the classic Greek period. The transition from the Middle Ages to the Renaissance gave rise to a basic change in the concept of map projection. During the Renaissance (particularly in the period 1470-1669), interest in map projections developed substantially and became more sophisticated mathematically. Mapmakers began to build on the work of their predecessors and to innovate. Many projection schemes were developed in this period, including the one most widely known today: "Unquestionably, the most famous projection is the one simply named for the inventor Gerardus Mercator" (Snyder 43).
An ideal map should meet several constraints. It should represent countries with their true shape, and the countries represented should retain their relative sizes in the map. Distances between arbitrary points as shown on the map should bear a constant ratio to their true distances upon the earth. Great circles (lines of longitude) upon the sphere, which are the curves of shortest length on the sphere joining given points, should be represented by straight lines, which are the shortest distances joining the points on the map ("geodesics"). Finally, the geographic latitude and longitude of any place should be easily derivable from its position on the map. Cartographers have worked for centuries to meet these conflicting requirements, but have yet to do so. Slowly over many years they have mastered individual traits of the ideal map, but have not been able to combine them altogether to create the ideal map.
Historically, one of the first steps in preparing a map is to lay down the graticule, or net of meridians and parallels, according to the selected map projection. Different map projections require different graticules. Meridians and parallels can be treated as if they represented precise coordinates, placed as straight lines or smooth curves on the map. The system of meridians and parallels, or lines of longitude and latitude, was developed near the beginning of the history of map projections. Latitude is defined as the angular distance north or south of the Equator. "A parallel of latitude is an imaginary line drawn around the Earth parallel to the Equator and at a constant angular distance to it" (Steers 17). Longitude is a similar angular measurement in an easterly or westerly direction. Unlike latitude, the reference point for longitude is arbitrary. "A meridian of longitude is a line passing entirely around the sphere and through the Poles" (Steers 17). Thus the parallels and meridians of a sphere intersect at right angles.
However, before meridians and parallels could be used as the framework for a flat map representing the globe, it was necessary to develop an artificial pattern of lines of longitude and latitude by which positions on the earth's surface could be given systematic locations. Eratosthenes (ca. 275-194 B.C.) had devised a system similar to meridians and parallels, but Hipparchus (ca. 190-126 B.C.) applied more rigor to relate astronomical measurements to the determination of climata, or latitudinal positions. He was the first to use a formalized system of longitude and latitude in which the meridian and parallel circles were each divided into 360 degrees, each degree into 60 minutes, and each minute into 60 seconds (following the sexagesimal system of the Babylonians). (Snyder 4)
The progress of science during this time revolutionized man's conception of the earth, and more precise methods of fixing position on the surface of the earth were being discovered. "Astronomers were able to deduce latitudes from the proportions between the lengths of the shadow and the pointer of the sun dial. This was the forerunner of the modern method of obtaining latitude" (Crone 19). The problem of the determination of longitude baffled astronomers for a very long time, because unlike the determination of latitude, for which the axis of the earth provides an established reference, no meridian is marked out as an initial one, in the way that the Equator serves as an initial parallel. Eratosthenes arrived at longitudes by "transforming distances into their angular values in relation to the circumference of the globe" (Crone 20). He also attempted to extend two lines of latitude based on the assumption that locations with similar climates and products would lie on or near the same parallel.
There have been several proposed classifications of map projections. One of the most common classifications is based on the association with a developable surface. Such a surface may be laid flat without distortion. The most common projections may be geometrically projected onto a cylinder or cone, and are referred to correspondingly as cylindrical or conic projections. "Probably the projection dominant among those inherited from the pre-Renaissance was a graticule appearing to be rectangular, called equirectangular" (Snyder 6). As we have seen, the idea behind this projection goes back to Eratosthenes.
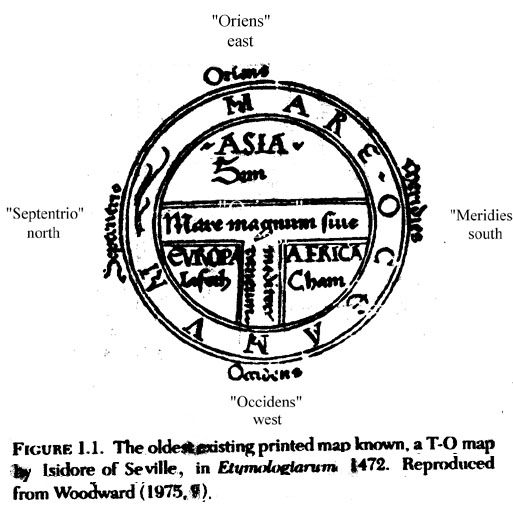
Claudius Ptolemy (90-170?A.D.) provided detailed instructions concerning some methods of map projection, but many of the types of maps dominant before the Renaissance were based on ideas that were philosophical rather than mathematical. These maps include d world maps or mappaemundi, such as the symbolic T-O maps on which the landmasses were neatly fitted into a circle:

Whether the earth was spherical or flat was irrelevant to these maps and their makers. Crone states "the O represented the boundary of the known world, the horizontal stroke of the inset T the approximate meridian running from the Don to the Nile, and the perpendicular stroke the axis of the Mediterranean" (Crone 26). However, Snyder explains, "the T-O design depicts the known continents s eparated by the Mediterranean Sea shaped like a T and surrounded by an O-shaped ocean" (Snyder 2).
With the expansion of the known world through the conquests of Alexander the Great and the Romans, a vast mass of detail accumulated for use by later cartographers, who were thus able to take up the task outlined by Eratosthenes and Hipparchus wit h greater assurance of success. "Ptolemy was possibly the single most influential individual in the development of cartography in Europe and the Middle East at the dawn of the Renaissance, although he lived 1300 years earlier" (Snyder 10). Not much is k nown of Ptolemy, yet we do have much of his technical writings such as his Geography. Ptolemy's Geography is essentially a guide to drawing the world map. The Geography is "an extensive table of the geographical coordinates of some 8000 localities" (Crone 21). Ptolemy obtained the positions of these localities based on his study of itineraries, sailing directions, and topographical descriptions. In several ways, however, his work hindered the development of an accurate map of the world. "One of Ptole my's principle mistakes was the adoption of a value for the length of a degree equivalent to 56.5 miles, in contrast to Eratosthenes' value" (Crone 23). When it came time to transform distances into degrees, the figures he obtained were considerably exag gerated. Many other misrepresentations in Ptolemy's work show up throughout the maps of the Renaissance. These imperfections were gradually eliminated as the exploration of the earth progressed.
During the early Middle Ages, geographical knowledge was fairly meager. Therefore, cartography was merely a routine copying of information; hence, many errors were repeated. By 1300 A.D., cartography began to emerge from its `dark ages'. Features of the mappaemundi were still persistent throughout the Renaissance maps. "Widening horizons presented greater incentives to the cartographer, and spurred him to the solution of more complex problems than had faced his medieval predecessor" (Crone 29). Thus, by the 13th century, advances in mathematics and astronomy spurred cartographers in their work.
Towards the end of the 13th century there came into use in Western Europe a type of chart based on direct observation by means of the mariner's compass. These charts are referred to as portolans. The portolan charts portrayed the coastlines and ports for sailors. Not many of these portolans have survived, but the few that have been preserved have several features in common. First, the area that they cover comprises the Mediterranean and Black Seas, with a portion of the Atlantic coasts of Europe. Second, each of these charts is covered by a system of lines, generally sixteen to thirty-two lines, radiating over the whole chart.(Crone 30) There is no known explanation of this system of direction lines.
Another notable stage was reached in the 14th century when European cartographers made the first attempt since classical times to include the continent of Asia within their maps. The results of these efforts are seen in the Catalan world maps. Catalan maps originated from the Catalan school. The Catalan atlas of 1375 illustrated here was constructed with the use of three sources:

The maps of the 15th century are consistent with the later Catalan maps in that they preserve some medieval features, but differ in that they show the influence of Ptolemy's Geography. Fra Mauro, a monk of Murano, near Venice, presented a world map that is often regarded as "the culmination of medieval cartography" (Crone 53). The last important pre-Columbian representation of the world was Martin Behaim's globe. Martin Behaim was a famous cosmographer from Nuremburg. Behaim's globe was important above all for the simple reason that it was a globe. It is thought that this globe stimulated "controversy over the initiation of Columbus' great design and the subsequent evolution of his ideas on the nature of his discoveries" (Crone 61).
The leaders of overseas exploration made the second great contribution to the revival of cartography. In little more than a century, the oceans of the world had been explored, and seamen were able to provide the chart makers with copious data for their maps. Hence, numerous maps were produced in the 16th century. The Flemish cartographer Gerardus Mercator sought to meet the public's demand for a comprehensive, up-to-date map. Mercator was a maker of mathematical and astronomical instruments. He solved the problem of the representation of the lines of constant bearing, or loxodromes, by straight lines on a chart. Since meridians and parallels are represented by straight lines at right angles, any straight line drawn in any direction crosses all parallels at a constant angle, and also all meridians at a constant angle. Such a line is called a loxodrome. If a line is to preserve a constant corrupt lines here * m to follow a single compass setting based on the bearing of the straight line connecting the point of departure and destination. How Mercator did this is as follows: To represent the loxodromes as straight lines on a flat map, the meridians and parallels must be arranged so that the loxodromes cut the meridians at constant angles. Since the meridians converge, the effect of this is to distort east-west distances, and therefore direction and area at any given point. If, however, the distances between parallels are increased proportionately to the increase in the intervals between the meridians from the Equator towards the Poles, the correct relationships of angles ar e preserved. This was the solution obtained by Mercator. (Crone 106)

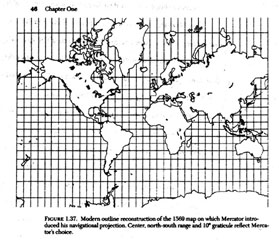
Mercator's principle achievements were his globe of 1541 and his world map of 1569, an outline of which is shown above. On his globe of 1541 Mercator first laid down the loxodromes, and in 1569 he projected these lines onto a chart, As illustrated below.. Mercator's map was intended not only to be used by navigators, but also to represent land surfaces as accurately as possible. "The projection is a regular cylindrical projection, with equidistant, straight meridians, and with parallels of latitude that are straight, parallel, and perpendicular to the meridians" (Snyder 45). Instead of stretching the Polar Regions north and south, Mercator limited the stretching in latitude to an equality with the stretching in longitude. Therefore we get a conformal projection in which any small area is shown with practically its true shape, but in which large areas will be distorted. For example, according to the Mercator map Greenland is bigger than South America, when in fact South America is nine times larger than Greenland.
In the seventeenth century, there was a desire to apply new theories of the physical universe to accurately determine the dimensions of the earth. The invention of new instruments enabled scientists to make the necessary observations. The instruments included the pendulum clock, the telescope, as well as more theoretical tools like tables of logarithms, the differential and integral calculus, and the law of gravity. The advance of cartography was furthered by the measurement of an arc on the earth's surface. In the eighteenth century, the advances in mathematics and astronomy associated with the work of Sir Isaac Newton helped perfect the method of determining longitude within one degree. The results of these technical advances were seen in the increased accuracy of the general outline of the continents and their precise positions. Maps of North America and the Indian sub-continent began to be sketched. The progress of the settlement of North America, the organization of colonies, and a continuing Anglo-French rivalry created an increasing demand for maps of greater reliability. Maps also played a key role in the peace between the American colonies and Britain.
The first map projections were based on little supporting information. The subject was basically mathematical, but although geometry and the beginnings of algebra and trigonometry had been developed by the time of Ptolemy, these were only minimally applied to map projections until after the Renaissance. Projections serve as tools to help display geographical data, but the placement of lands over the face of the earth not known amplified by very limited exploration until the Renaissance. After the surge of i nterest by Eratosthenes, Hipparchus and Ptolemy, there were centuries of stagnation resulting from wars and religious intolerance. The Renaissance, however, reawakened mathematicians and cartographers alike. Mercator has provided generations upon generations with perhaps the single most important navigational as well as geographical tool available to this date. "Whatever the future may hold, it is at least clear that the problems and opportunities facing the cartographer will not decrease" (Crone 171).