The Oxford English Dictionary effortlessly defines zero as "Nought or nothing, reckoned as a number, denoted by the figure 0; the total absence of a quantity, considered as a quantity," (OED, p. 802) but it took ancient mathematicians m illenia to come to accept this "absence of a quantity" as a number. Although the question of the time and place of the first introduction of a true zero sign remains unsettled, the history of the development of the notion of zero proves to be quite fascinating. We will attempt to follow this process from the time of Old Babylonia to the introduction of the system of Hindu-Arabic numerals to the West.
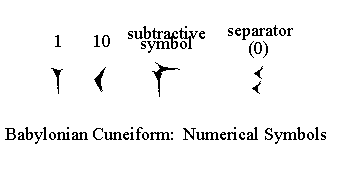
The earliest positional numeral system known to historians of mathematics is the notation found in Old Babylonian texts. Numbers less than 60 were represented by a base ten scale, while numbers greater than 60 were built from these "digits" in a sexagesimal scale. This positional numeral system was developed by the ancient Babylonians between 1800 and 1600 B.C. (Neugebauer, p. 15, 29) and expressed numbers using three symbols in a style of writing called cuneiform from the Latin for "wedge-shaped" as shown below.
These wedge-shaped characters were inscribed with a short stylus onto wet clay tablets which were then baked in an oven, rendering them almost indestructible. Fragments of the semi-permanent records that resulted from this process still exist today and have been decoded by specialists. The symbols used include a subtractive symbol, a symbol for one, and a symbol for ten called the Winkelhaken (Held, p. 1), a German word meaning "corner hook". With the application of the multiplicative and additive principles, and less commonly a subtractive principle, the mathematicians of Old Babylonia could represent arbitrary integers efficiently. as well as many fractions, using these symbols. Some examples of written numbers employing these symbols follow:
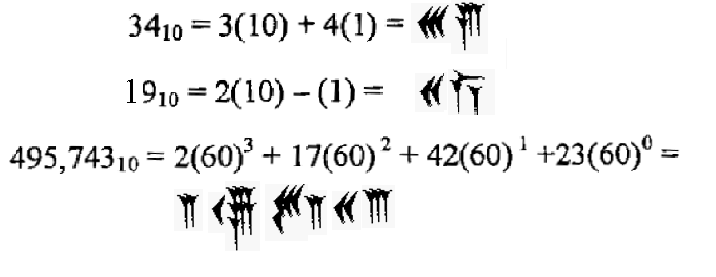
However, it soon became apparent that in this notation the lack of any notation for absent positions, led to ambiguity and resulted in errors. For instance, in the middle of the second row from the bottom of Plimpton 322 (fig. 2), a Babylonian clay tablet with cuneiform numerals from about 1800 B.C., we can read a number which is decoded as 29; (Neugebauer and Sachs, Plate 7). Although this decoding is correct in that context, it is quite possible that these same symbols could be read as 1209 or 36,609, as follows:
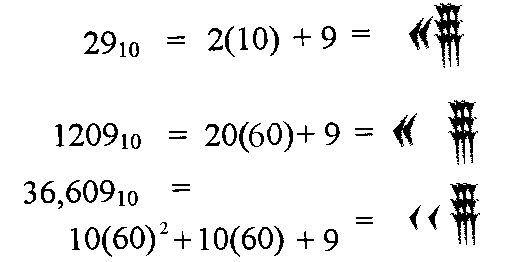
Clearly, the cuneiform characters used to represent the numbers 29 , 1209 , and 36,609 are essentially identical.
Recognizing this problem, the mathematicians of Old Babylonia eventually introduced a separation sign (fig. 1) to indicate the absence of a sexagesimal order, which was less ambiguous than the blank space which may be seen for example in the celebrated "Pythagorean triple" text, Plimpton 322. This separation sign met the difficulties associated with the non-ciphered (lacking zero) positional numeral system of Old Babylonia, and was in use at least by the beginning of the Seleucid period, about 300 B.C. (Neugebauer, p. 29), if not earlier (textual evidence is incomplete). This symbol for zero was used merely to indicate the absence of a sexagesimal unit of a particular order, as illustrated by certain lines of a mathematical tablet from the late third century B.C. whose fragments were found in archeological digs at Uruk (Ifrah, p. 380, fig. 3). Lines 10, 14, and 24 of this tablet make use of the Babylonian zero, a cuneiform symbol of two adjacent slant wedges (fig. 1). The tenth line of this tablet presents the Babylonian zero in writing as shown below:
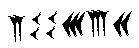
Uses of the Babylonian zero in
the fourteenth line are as follows:
3645 = (1,0,45)
= 
(misprint in figure should be corrected, my error - GC)
and 12961000 = (1,0,0,16,40) =
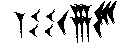
And finally, from the twenty-fourth line we have:
216450 = (1,0,7,30) =
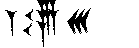and 7215 = (2,0,15) =
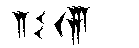
This Seleucid Babylonian "zero" functions essentially as
punctuation, and is generally not considered as the truly "numerical"
zero we are familiar with today. However, the research of the
mathematical historian Otto Neugebauer, one of the outstanding
specialists for the Babylonian period, demonstrated that Babylonian
astronomers differed from other Babylonian mathematicians by
incorporating the zero symbol not only in the midst of a written
number, but also at the end, and even at the beginning of written
numbers (where it might function, for example, as a decimal point).
An astronomical tablet from this period, currently at the British
Museum, exhibits this advanced use of the Babylonian zero (Ifrah, p.
380).
This tablet (figure unavailable) recorded the number 60 written
as:
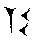
When Babylonia came under Greek domination as a result of the
conquests of Alexander the Great, the Greeks had access to Babylonian
advancements in mathematics and astronomy. They had learned something
of Babylonian geometrical theories in the time of Pythagoras (6th c.
B.C); now they learned their arithmetical methods, and along with them
the concept of the Babylonian zero (Neugebauer, p. 156). Rather than
using the Babylonian cuneiform notation however, the Greeks continued
to use their own alphabetic numeral system for the sexagesimal
"digits", towhich they added a new symbol for 0. The particular
symbol used by the Greeks for zero varied and evolved over time
(Ifrah, p.383-384, fig. 4). Around the second century B.C., the
"hellenistic" zero, a small o, was employed to facilitate the use of
negative powers of 60, or Babylonian sexagesimal fractions, necessary
in applications to astronomy. Greek astronomers, such as Ptolemy,
made much use of this symbol, long thought to be an abbreviation of
the Greek word ouden meaning "nothing", to indicate a missing place and
used it in writing Babylonian sexagesimal fractions (Menninger, p.
399; Neugebauer disagrees). For example, the Greeks would write
decimal approximations of 0.47639 and 0.29694 as
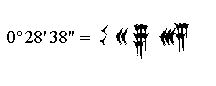and
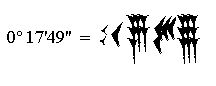
respectively (Ifrah, p. 383). The zero
sign at the beginning of a string of numerals permits the correct
interpretation of the intended fractional values of these numerical
expressions. The Hellenistic Greeks followed the Babylonian
astronomers in their use of the zero to indicate an empty position at
the beginning, middle, or end of a sexagesimal number. They developed
the astronomical theory farther (spherical trigonometry as developed
by Hipparchos), but did not significantly change the numerical notations.
The point at which the symbol zero was transformed into the number zero remains unclear, and is evidently subject to more than one interpretation. As Karl Menninger, a historian of the mathematical aspects of culture, wrote, strikingly: "For the Indians this step [from numeral to number] cannot have been a very big one" (Menninger, p. 399). The "Greco-Babylonian model (Menninger, p. 399) "triggered the final spark" that allowed the Indians to develop their positional (and decimal) numeral system, incorporating zero as a number. Similar to the Babylonian and Greek development of the zero sign, the Indian zero was used at the beginning, in the middle, and at the end of number expressions. In addition to the occurrences found in old texts that allowed zero to indicate magnitude and to hold a place-value in written numbers, we also come across many calculations by Indian mathematicians with the newly acquired number zero. Centuries after the Babylonian and Greek empires, the Indians developed a true zero, as the decipherment of temple walls and ancient scriptures reveals. The Gvalior Inscription dating back to 870 A.D. displays the one of the earliest attested uses of the number zero in Hindu mathematics (Menninger, p. 400, fig. 5), but texts using the number zero have been discovered as early as 598 A.D. (Ifrah, p. 495), in a religious tablet that mentioned the numbers 270 and 50. The zeroes in these numbers are not merely place values within the number, but like the Seleucid zero indicate the magnitude of the number. Although these aspects of the zero sign were already in use during Babylonian and Greek times, the Indian zero was not the special province of specialists, but was part of the generally adopted decimal number system, as witnessed by its use in religious texts and in public inscriptions. We have also referred to its use in mathematical computations. Thus, it may be concluded that the true zero was born in the Hindu mathematical community.
The works of the 9th century Indian mathematician Brahmegupta and the 12th century mathematician Bhaskara incorporate the use of the zero, or as Colebrooke translates it, the cipher (the Sanskrit word used is sunya, meaning "void"). Brahmegupta documented the properties of the zero in his early algebraic text Cuttaca. He correctly stated the additive, multiplicative, and exponential properties of the zero as they are implied in a modern sense (Colebrooke, p. 339-340), and also gave rules for division by 0. Bhaskara's mathematical work in algebra (as the Europeans called it, using an Arabic word) is recorded in the Vija-ganita, and was translated from the Sanskrit into English by Henry Thomas Colebrooke in 1817; there is no modern translation. Some of Bhaskara's calculations specifically called for the number zero. The properties of the number zero were explored in example 15:
In modern notation, Bhaskara's solutions run:
0 × 2 = 0,
0 / 3 = 0,
and 3 / 0 = 3 / 0 [formally].
In this example, Bhaskara explicitly showed that the product of any number multiplied by zero was zero. Zero divided by any quantity was zero, while some quantity divided by zero was simply written as the fraction of the given quantity over zero. Bhaskara did note that "this fraction, of which the denominator is cipher, is termed an infinite quantity (Colebrooke, p. ?? )." This notion of "infinite quantity" could be construed as a step in the direction of the modern theory of limits. In another case, quadratic equations were evaluated, as Bhakara asked in example 135:
In other words, Bhaskara has set up the following quadratic
equation:
(a² + a) × 0 = 90, where a = (x/0 + x - 9)
Bhaskara claimed that the answer is: x = 9; that is, a = 9/0. This answer could be considered correct if (9/0)2 = 81/0, and if division by 0 is construed as a limit, with multiplication by 0 brought within that limit. But these two points of view are irreconcilable; at least, no meaningful interpretation of these calculations is known to me. This calculation does not make sense from a modern point of view. Bhaskara is however attempting to use the zero as an "ordinary" number in these examples; and it is probable that the division by 0 is intended to be taken in the sense of a limit, which could be of use in Indian astronomy.
Once the concept of the zero was grasped by the Hindus, the idea spread, via the Arabs, to the West, with some initial resistance. Leonardo of Pisa, better known as Fibonacci, resided in Italy but traveled extensively in North Africa, and to some degree in the Middle East. As a professional accountant with a strong mathematical gift, he studied with an Arab mentor and learned of the Indian zero. The publication of Fibonacci's Liber abbaci in 1202 introduced this concept, and much else, to a wide circle in Europe (Menninger, p. 401, 425), though the ideas were known to a few scholars in Europe somewhat earlier. Although businessmen and scholars were a bit skeptical of this novel idea, merchants quickly adopted the algorithms described by Fibonacci, and used them to solve problems of interest, profit, and currency exchange. The zero concept came to the Greek (Byzantine) world much later, and not from Western Europe but via a second introduction from the east by Maximus Planudes in the 15th century (M. Cantor). In this way the place-value concept, along with the notion of zero, came into Western mathematics.
In conclusion, the zero concept has undergone a rich and varied devlopment on its way toward becoming a full-fledged number. After its first appearance in Babylonia, the zero journeyed through several cultures, including the Greek, Hindu, and Islamic, before being transmitted to the West. Although its history is at points hazy and conjectural, its acceptance as a number has been essential to further progress in the field of mathematics.