
Spizella passerina
 |
| Chipping Sparrow Spizella passerina |
|---|
Cook College students are supposed to admire all furry and feathered creatures. This includes math faculty members. On the other hand, Douglass College students may be allergic to feathers, and therefore creatures with feathers can be plucked and prepared for consumption by the students' extensive household staffs: chefs, butlers, etc.
| Which of these birds was not seen today in my back yard? | |||
|---|---|---|---|

| 
| 
| 
|
| Fox Sparrow Passerella iliaca | White-throated Sparrow Zonotrichia albicollis | Song Sparrow Melospiza melodia | Phoenix |
Please avoid mistakes
I began by discussing the last QotD. This involved a model of
the pressure, P, volume, V, and temperature, T, of a gas:
PV1.6=kT, where k is a constant. This QotD was certainly
similar to questions which can be asked on Math 135 exams. I would
like you to answer these questions correctly. Therefore, to a certain
extent, if you "need" (?) to make mistakes answering such questions,
please make the mistakes here rather than on an exam. Several
solutions to the problem I asked are given in the previous diary
entry. Here I want to list some common errors. Please guard
yourself from these:
A return to the snowball
A few lectures ago we analyzed a problem about the
melting of a snowball. I felt somewhat dissatisfied with the statement
of the problem, because I didn't think the statement gave enough
background. Let's look again at the problem. The setup is certainly
simplified from "real life". The snowball is a sphere of radius r,
with surface area S=4Pi r2 and with volume
V=[4/3]Pi r3. Put the snowball in a warm
environment. The snowball will melt, of course. But how does it melt?
If you think about it, the volume, V, will decrease, but more
precisely it will decrease as the snowball absorbs heat. Heat is
absorbed through the surface of the snowball. (I don't think in this
model that we should imagine a little machine in the middle of the
snowball radiating heat!) So I think that the rate of change of the
snowball's volume should be directly proportional to the surface
area. That is, there is a constant k so that dV/dt=kS. What happens to
the radius of the snowball? Well, since V=[4/3]Pi r3,
then (r varies!)
dV/dt=[4/3]Pi 3r2[dr/dt]. Match this with dV/dt=kS,
and, wow!, we see that dr/dt=k. So if we believe this model, then (as
the original problem statement specifies) the radius of the snowball
is constantly decreasing.
The surface area of a baby?
The snowball problem is quite relevant to certain aspects of
biology. There are approximate formulas for the surface area of a
baby. Such formulas can be useful if estimations of fluid balance
(sweat) or temperature change are needed. Babies are smaller than
adults, and such balances may be very unstable. If the balances are
not maintained, illness and even death can result.
So how does the radius change?
The snowball problem is quite relevant to certain aspects of
biology. There are approximate formulas for the surface area of a
baby. Such formulas can be useful if estimations of fluid balance
(sweat) or temperature change are needed. Babies are smaller than
adults, and such balances may be very unstable. If the balances are
not maintained, illness and even death can result. Notice that the
crucial number is the ratio between the surface area, S, and the
volume, V: for a sphere this is 4Pi r2 divided by
[4/3]Pi r3, which is 1/3r. When r is large, this is
small, so the surface area "percolation" won't be that important in
fluid or temperature balance, But when r is small, this ratio is much
larger, and surface area considerations can be vital. I don't think
aducts or babies are spheres (!) but still some of the same reasoning
will apply.
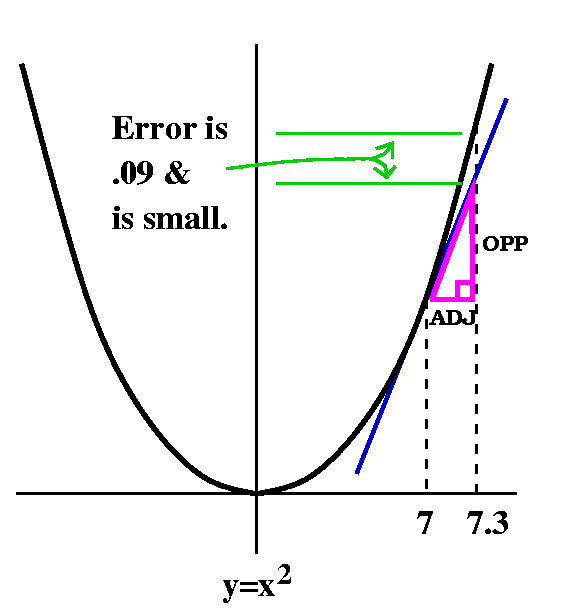 What is (7.3)2?
What is (7.3)2?
Well, (7.3)2 is exactly 53.29 as some people were happy to
tell me. But then I said that there was a way to approximate this
number using calculus. Look at the picture on the right. The true
value of (7.3)2 is the length of the dashed line which,
at 7.3, is perpendicular to the x-axis. Now consider the tangent line
to y=x2 at x=7. Tangent lines to this parabola have slope
equal to 2x (since this is the derivative of x2). Therefore
the slope of the tangent line at x=7 is 14. This slope is the tangent
of the angle that the line makes with the x-axis, and therefore it is
equal to the ratio, OPP/ADJ (abbreviations for "Opposite over
adjacent"). So 14=OPP/ADJ. Now if
we add OPP to the length of the line perpendicular to the
x-axis at x=7, we will get an approximation to (7.3)2.
Notice that OPP=14ADJ.
Here
are the numbers:
f(7) + f´(7)(.3) 72 + 14(.3) = 49+4.2 = 53.2This is an approximation to the true value, 53.29. This scheme has various names. The name I like the best, because it reminds me of the geometry, is the tangent line approximation. You can look at this picture and see that there isn't much error, although the pictures drawn in this lecture may not necessarily be true to the actual proportions
| f(x+h) is approximately f(x)+f´(x)h |
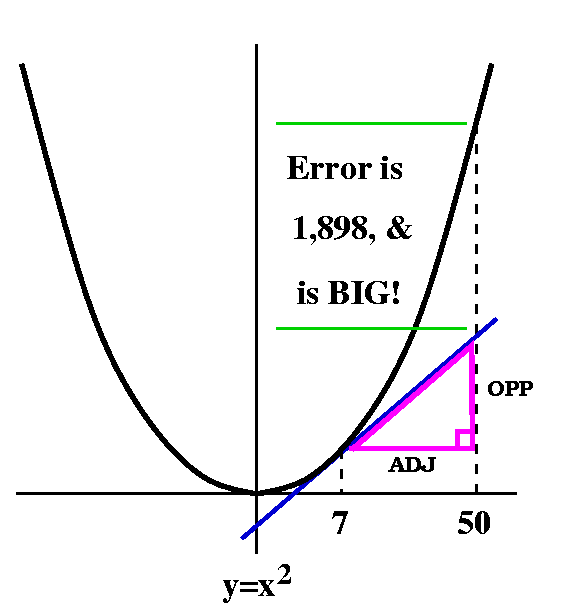 What is (50)2?
Well, we can use the same x: x=7. Now if I want x+h to be 50, h should
be 43. Then the statement, f(x+h) is approximately f(x)+f´(x)h,
becomes
What is (50)2?
Well, we can use the same x: x=7. Now if I want x+h to be 50, h should
be 43. Then the statement, f(x+h) is approximately f(x)+f´(x)h,
becomes
72+14(43) and this is 49+602, which is 651.
The true value is, of course, 502=2,500. The real picture is
even worse than what is shown here. I don't think that 651 is a very
good approximation to 2,500.
So it seems that sometimes it can be a good idea to use this tangent
line approximation, and sometimes it can be a silly idea, or even a
dreadful idea. There are ways of analyzing the error, and I will
mention some, but if you use this approximation (and most of you
really will use it!) you need to be careful, and worry about the
error (the discrepancy between f(x+h) and f(x)+f´(x)h) and
worry sometimes about the relative error: if the "true" value
of f(x+h) is 128,453,201, an error of, say, 6,500 doesn't matter very
much, but if the true value is 8,000 an error of 6,500 is
horrible.
The chip company again
We actually have already used the idea of the tangent line
approximation, which the textbook frequently calls the
differential We first saw this wnen we analyzed the finances of
the chip company. Let me reproduce just the
beginning of the information we had:
CHIPCO
INVESTMENT DOLLARS & PRODUCTION
Capital Invested Chips produced Marginal chips produced
$ in millions 1,000's of units 1,000's of units per
millions of $'s
200 3,000 .23
A key word here is marginal. Major vocabulary step:
| "Marginal" means "rate of change" means "derivative". |
Here is how to read the data quoted above about the chip company.
If M=capital invested, and C=chips produced (all quoted in the
units at the top of the table) then we kow:
C=C(M), C is a function of M, and more precisely, C(200)=3,000
and [dC/dM](200)=.23.
We then used this information to try to predict what chip production
would be if we increased the invested capital by, say, 6 million
dollars. This means that we'd like to approximate C(206). So here we
go:
C(206) is approximately C(200)+C´(200)·6=3,000+(.23)6 thousands of
chips. Here x is 200 and h is 6.
 Biological systems are complicated
Biological systems are complicated
I wanted to give an example of the sometimes paradoxical responses of
biological systems, and this becomes very evident if this linear
approximation idea is carried out thoughtlessly.
An easy example of the possible complications is determining dosages
of psychoactive drugs. Unfortunately this example is also quite
technical and intricate. I've been unable to find simple data
(numbers, graphs) on the web to support the following assertions which
I believe after reading various sources.
Both human drugs (Prozac is an example) and animal drugs (certain
horse tranquilizers) show the following effect in relief of
symptoms. An initial dose of the drug gives some help, and then small
additional doses give more help. Mathematically, f(x)>0 and
f'(x)>0, so f(x+h), for suitably small h, is even better. However,
in both cases, if h is sufficiently large, f(x+h) is actually much
worse than f(x). In practice, as I mentioned in class, there are
usually initial doses suggested mostly by the recipient's weight
(mass) and metabolism (fast/slow, adolescent/mature adult, etc.). Then
there are recommended changes (the derivative). But careless or
impatient or unknowing people can just "assume" that the linear
approximation holds for relatively large h's. Sometimes the results
can be very unpleasant.
Apology I hope I have not gotten the general idea wrong. I am
definitely not an expert in this material, which is quite
complicated. Please let me know if you think I have communicated
something wrong. Thank you in advance.
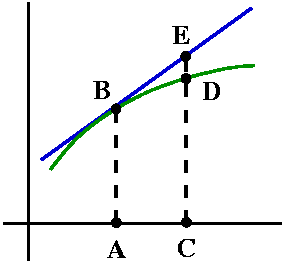 The formula and a picture
The formula and a picture
The formula is, again,
f(x+h) is approximately f(x)+f´(x)h
I asked people to try to identify as many of the terms possible in a
picture like the one shown here. Here is how I would assign
things:
A is x.
The length of the line AB is f(x).
The length of the line AC is h.
The length of the line CD is f(x+h).
The length of the line CE is f(x)+f´(x)h.
The length of the line DE is the
error, the difference between the true value and the tangent
line approximation.
This curve bends down (we will call it "concave down" later)
and this bending causes the tangent line approximation (the
differential) to be an overestimate.
If you flip the curve, the approximation will be an underestimate, and
this occurs when the curve bends up.
Still ahead
I will try to give you more information about the error later when we
have more specific language. The bending has to do with how
f´(x) changes (the tangent lines have changing slopes), so it is
actually governed by f´´(x), the second derivative. The
bending has specific names which we will learn: concave up and
concave down. The picture shown is concave down, and
f´´(x) is negative.
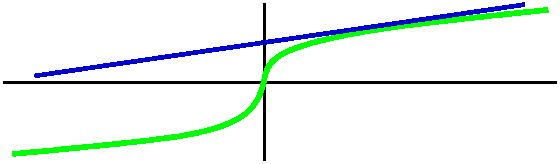 QotD
QotD
What is the approximate value of (7.98)1/3? here I asked
that people not use calculators, and use the linear approximation
scheme discussed above.
I drew the picture shown, and asked if the approximate value would be
more or less than the true value.
Solution
Here f(x)=x1/3, so f´(x) is (1/3)x-2/3. The
specific x value I'd use would be x=8 and I'd take h to be -.02. I was
"nasty" and tried to surprise people with a negative value of
h. The famous (?) f(x+h) is approximately
f(x)+f´(x)h becomes:
x=8, so f(8)=81/3=2 and
f´(8)=(1/3)2-2/3=(1/3)(1/4)=1/12. Therefore
(7.98)1/3 is approximately 2+(1/12)(-.02).
The picture tells us that the approximation will be an
overestimate. The true value is less than the approximation
because the tangent line in this case is above the curve.
Indeed, an electronic friend of mine reports that this tangent line
approximation is 1.998333333 while the "true" value is 1.998331943.
HOMEWORK
Please read sections 3.7 and 3.8 and hand in these problems on
Thursday:
3.7: 4, 15, 24, 25;
3.8: 19, 20, 25, 35.
x2+xy+2y2=11 (1,2) is on this ellipse. dy/dx=-(2x+y)/(x+4y)I asked for an equation of a line tangent to the ellipse at (1,2). I wanted people to use the information in front of them and insert (1,2) into the equation for dy/dx. The result is -(2(1)+2)/(1+4(2))=-4/9, the slope of the line. Then a valid answer is:
Why QotD?
I guess I can see who is coming to class. Wow. This is not too
valuable, since what I really report on via the student grades for
the course is whether you have learned the
material, principally as shown by work done on exams. The QotD is a
way to communicate between the instructor and the students. I will try
to give a meaningful, hopefully interesting problem. You try to show
me what you do or don't know. I will try to give feedback in the next
class. I can learn if I did a "good" job teaching. You can learn about
your learning and your own mastery of the work, and see what I think
of this. I honestly believe this is a good deal for you.
Differentiating a few more things ...
What about y=10x? Take logs. Rather, take lns. This becomes
ln(y)=x ln(10). Then d/dx this equation. The Chain Rule applies
to the left side and gives: (1/y)(dy/dx). The right side is
CONSTANTx, so its derivative is just the
CONSTANT. Therefore we know that (1/y)(dy/dx)=ln(10), and
dy/dx=ln(10)y, and since y=10x,
dy/dx=ln(10)10x=(2.30258)10x. Most
people don't like that weird number, so they stick to ex
in calculus.
Here is (approximately) a problem from WeBWorK. Suppose
f(x)=(5x2+7x+9)sin x. What is f´(x)? First
I will take ln's and then I will d/dx:
ln(f(x))=ln((5x2+7x+9)sin x)=(sin x)ln(5x2+7x+9) because
ln(AB)=Bln(A). Now let us d/dx the equation, not forgetting
either the Chain Rule or the Product Rule.
Left side So d/dx of ln(f(x)) is [1/f(x)]f´(x).
Right side d/dx of (sin x)ln(5x2+7x+9) is:
(cos x)ln(5x2+7x+9)+(sin
x)[1/(5x2+7x+9)](10x+7).
Together So [1/f(x)]f´(x)=(cos x)ln(5x2+7x+9)+(sin
x)[1/(5x2+7x+9)](10x+7) and if we multiply by
f(x) and use the original formula for f(x) we get:
f´(x)=((5x2+7x+9)sin x)[(cos x)ln(5x2+7x+9)+(sin
x)[1/(5x2+7x+9)](10x+7)]
You will never see formulas like this outside of
a first semester calculus course.
Squares that grow
I began with this problem:
| Suppose we have a square whose edges are increasing at 2 cm/min. How fast is the area of the square increasing when the area of the square is 100 cm2? |
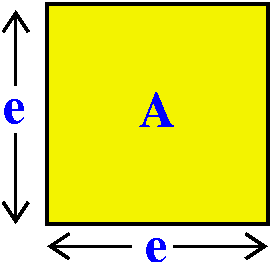 We discussed this problem. I emphasized the need to translate things
to mathspeak, including the dialect calcspeak.
We discussed this problem. I emphasized the need to translate things
to mathspeak, including the dialect calcspeak.
Roger and Jane
Here's the statement:
| Suppose Roger is driving north from the center of town at 40 miles per hour, and Jane is driving east from the town center at 50 miles per hour. How quickly is the distance between them changing when Roger is 10 miles north of town and Jane is 30 miles east of town? |
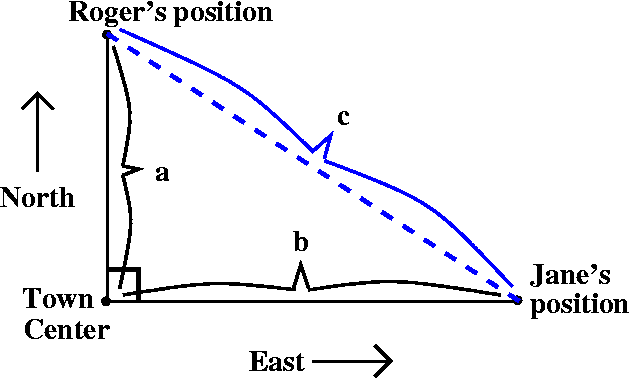 This outline doesn't always work, and sometimes parts of it aren't
needed. To the right is a diagram (P). The
basic outline is a right triangle. The "legs" of the triangle are
Roger and Jane's distances, which vary with time, from the town
center. This distance certainly varies. I have labeled (L) the two distances a and b. The distance
between them is called c. What I know (I)
from the problem statement is that da/dt=40 and db/dt=50, and that at
a certain time (which is not known) a=10 and b=30. I would like to
know (W) what dc/dt is at that "certain
time". There is one more ingredient (E).
Since north and east are perpendicular, we have Pythag:
a2+b2=c2.
This outline doesn't always work, and sometimes parts of it aren't
needed. To the right is a diagram (P). The
basic outline is a right triangle. The "legs" of the triangle are
Roger and Jane's distances, which vary with time, from the town
center. This distance certainly varies. I have labeled (L) the two distances a and b. The distance
between them is called c. What I know (I)
from the problem statement is that da/dt=40 and db/dt=50, and that at
a certain time (which is not known) a=10 and b=30. I would like to
know (W) what dc/dt is at that "certain
time". There is one more ingredient (E).
Since north and east are perpendicular, we have Pythag:
a2+b2=c2.
Let us d/dt the equation. The result is
2a(da/dt)+2b(db/dt)=2c(dc/dt). We know values of a, da/dt, b, and
db/dt, so that 2(10)(40)+2(30)(50)=2c(dc/dt). What is c? We can get c
from the original equation: a2+b2=c2
becomes (10)2+(30>2=c2, so that
c=sqrt((10)2+(30>2). This allows us to solve for dc/dt:
dc/dt=[2(10)(40)+2(30)(50])/sqrt((10)2+(30>2)
Comment I remarked that this problem is not as silly as it might seem. One simple problem dealing with cell phone networks is similar to this. Maybe imagine a cell phone user driving (or even, as I mentioned, flying). How fast is the distance changing between the user and the network of cell phone towers? That sort of information could be rather important in practice.
Pulling taffy
We all know that pulling taffy is a principal industry at the New
Jersey shore. There are these wonderful machines that take sticky
stuff and, well, pull it. Why? The pulling changes the texture. I hope
some of you will try
this. Let me make a simple model of taffy pulling.
|
|

|
|
Is pressure really measured in ATM's? |
Solution of the QotD
So d/dt the equation PV1.6=kT
remembering both the product rule and the Chain Rule, and that
k is a constant. This is the result:
(dP/dt)V1.6+P[1.6V.6(dV/dt)]=k(dT/dt)
The .6 comes from 1.6-1, I think. Let's insert all of the known
values:
(+.2)101.6+2[1.6(10.6)(-3)]=.398(dT/dt).
Wow. I get -76.02, approximately, for dT/dt. So my number states
that T is decreasing, and the rate of decrease is the that
number.
Another way to solve the QotD
Well, some students took the equation PV1.6=kT and ln'd it
first. They should have gotten ln(P)+1.6ln(V)=ln(k)+ln(T). If this
equation is d/dt'd, we get
(1/P)[dP/dt]+1/6(1/V)[dV/dt]=0+(1/T)[dT/dt]. Notice that dk/dt is
still 0, since k is still a constant. Then insert the various values
of everything and solve for dT/dt. The result should be the same.
HOMEWORK
Please read sections 3.6 and 3.7 and do homework problems.
| Function | Its derivative |
|---|---|
| sqrt(1+5x2) | (1/2)(1+5x2)-1/2(0+10x) |
| tan(78x) | (sec(78x))2(78) |
| Comment The squaring of secant is part of the derivative of tangent. Sometimes this can give irritation if you forget it. | |
| ln(37+sin(8x)) | [1/(37+sin(9x))](0+cos(8x)8) |
| Comment This is a composition inside of a composition! You need to use the Chain Rule twice. | |
| (3e2x-7e5x)500 | 500(3e2x-7e5x)499(3e2x2-7e5x5) |
| Comment Another double composition. Even if you know what you are doing, you can make "bookkeeping" errors quite easily. Please use many, many pairs of parentheses. The person you are helping is yourself! | |
| cos(3sin(5cos(x2))) | (-sin(3sin(5cos(x2))))(3cos(5cos(x2)))(-5sin(x2))(2x) |
| Comment Hey -- how many compositions does this function have? Who cares? Just try to keep track of the layer of the onion you are "traversing". | |
| sqrt(7+sqrt(2+x2)) | (1/2)(7+sqrt(2+x2))-1/2(0+(1/2)(2+x2)-1/2(2x)) |
| Comment Another double composition. | |
| Suppose f(x) is differentiable, and you know that f(1)=3 and f´(1)=7. Define F(x)=f(x2). What is G´(1)? | F´(x)=2(f´(x))1(2x) so that F´(1)=2(f´(1))(2·1)=2·7·2·1=28. |
| Suppose f(x) is differentiable, and you know that f(1)=3 and f´(1)=7. Define G(x)=(f(x))2. What is G´(1)? | G´(x)=2(f(x))1(f´(x)) so that G´(1)=2(f(1))(f´(1))=2·3·7=42. |
| Comment The two examples just done are an effort to show you that order of composition matters a great deal in using the Chain Rule. Look: the answers, 42 and 14, are not the same! | |
Important!!
The Chain Rule is probably the most useful differentiation algorithm.
algorithm [Math.] a process or set of rules used for calculation or problem-solvingIt will be used in many ways in this course. The idea of multiplication of rates, as I discussed last time in the chip example, is also one which you will use in real life (if you are alert enough to recognize it!).
3.5 #52
I discussed an actual textbook homework problem. Let me first quote
what problem writers call the stem of the problem, the
descriptive material which sets the stage:
| Assume that a spherical snowball melts in such a way that its radius decreases at a constant rate (that is the radius is a linear function of time). Suppose it begins as a sphere with radius 10 cm and takes 2 hours to disappear. |
| a) What is the rate of change of its volume after one hour? (Recall V=(4/3)Pi r3.) |
| b) At what rate is the surface area changing of after one hour? (Recall S=4Pi r2.) |
d/dt
"Let's get rid of the bugs, and use d/dt ..."
This
was supposed to be a joke. Oh well, here is more than I
know about ddt.
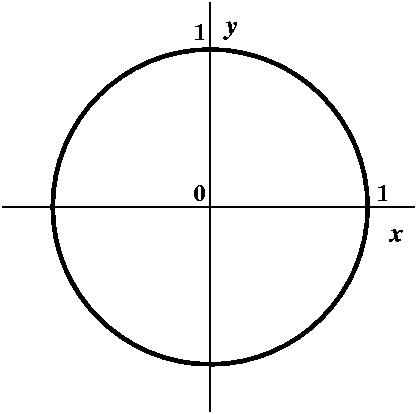
A useful trick: implict differentiation
The circle with center at (0,0) and radius 1 is
x2+y2=1. Suppose we wanted to find the slope of
the tangent line. Most of what we have done in this course applies to
graphs of functions, and the unit circle is definitely not the
graph of a function. How could we find the slopes of tangent lines?
Well, we could "solve" for y as a function of x:
y=+/-sqrt(1-x2)
and then differentiate, using the Chain
Rule. The +/- sign there is the algebraic version of the idea of
splitting the unit circle into its TOP half and its bottom HALF. Each
of these is the graph of a function.
What the heck can we do to minimize work? And what can we do if
we don't have an equation that's so easy to solve for x?
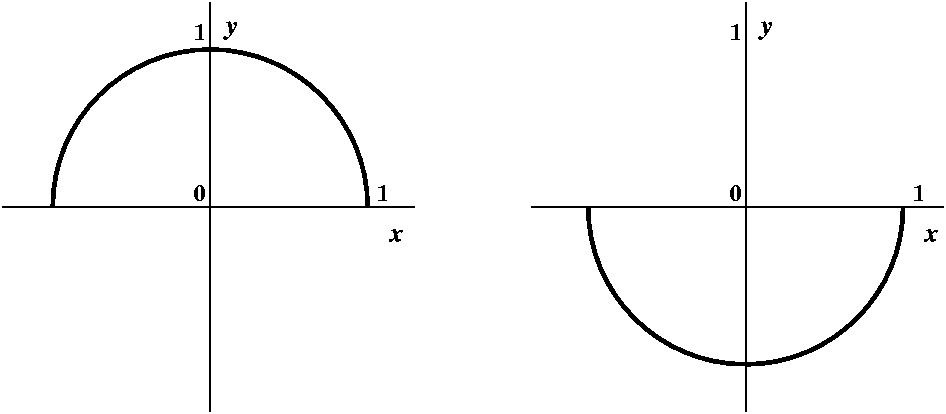
What we can do is d/dx the whole equation
x2+y2=1. The right-hand side offers no
problem. The first term on the left gives 2x. The problematical one
is y2. Here we need to realize that y is some "implicit"
function of x.
implicit implied though not plainly expressed.Therefore d/dx(y2) must be (Chain Rule!) 2y·dy/dx. Thus we have 2x+2y(dy/dx)=0, and so dy/dx=(-2x)/(2y)
How about another one?
I did something ugly. Maybe it was sort of like this:
Assume that y is implicitly defined as a function of x by the
equation
5x3+7x2y5-9y7=38.
What is dy/dx? I would try to d/dx the entire equation, and try
to remember to use the Product Rule and Chain Rule as appropriate.
Again, it is easy to lose one's way in all this.
|
5x3 + 7x2y5 -9y7 = 38
Just d/dx The product rule The Chain Rule
& the Chain Rule
15x2 + 14xy5+(7x2)(5y4)(dy/dx) -9·7(y)6(dy/dx) =0
Now collect terms without dy/dx and put them on the other
side. Collect the dy/dx terms and factor out dy/dx:
[(7·5)x2y4-(9·7)y6](dy/dx)=-[15x2+14xy5]
Divide by the dy/dx coefficient and get "the answer":
-[15x2+14xy5]
dy/dx= --------------------
[(7·5)x2y4-(9·7)y6]
|
So here is Implicit differentiation:
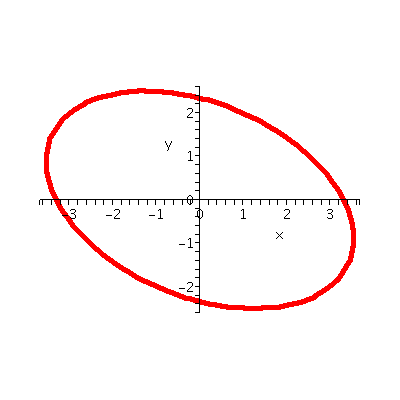 Maybe a more realistic example
Maybe a more realistic exampleHow is "typesetting" done? Many times people want to display paragraphs of type and pictures and tables and ... lots of things. In order to allocate space, each "chunk" of the page has a bounding box. This is the smallest box (rectangle with sides parallel to the coordinate axes) which contains the figure. This bounding box is then used to pack the chunks of the page neatly together. What is the bounding box of this ellipse?
 I've tried to draw the bounding box and the ellipse together here. The
geometry says that there are four points where the box touches the
ellipse. If we knew the coordinates of the points, then we would be
able to find the dimensions of the bounding box.
I've tried to draw the bounding box and the ellipse together here. The
geometry says that there are four points where the box touches the
ellipse. If we knew the coordinates of the points, then we would be
able to find the dimensions of the bounding box.
The top and bottom of the box are determined by two points where the
tangent line is horizontal. Maybe we could find these points. They
would be points on the ellipse where dy/dx is 0. So let's find dy/dx
when x2+xy+2y2=11. I'll use implicit
differentiation. I'll begin by d/dx'ing the whole equation. The result
is:
2x+y+x(dy/dx)+4y1(dy/dx)=0.
On the left-hand side, I used the product rule and Chain Rule on the
second term and the Chain Rule on the third term. Then we can regroup
the terms in the equation">br?
[x+4y](dy/dx)=-(2x+y)
and solve for dy/dx:
dy/dx=-(2x+y)/(x+4y).
When is dy/dx=0? Well, the only way a fraction can be 0 is when the
top is 0. So this means that -(2x+y) is 0, os 2x+y=0 or y=-2x. But the
point (x,y) is also on the ellipse, so that
x2+xy+2y2=11 must also be true. If y is -2x, I
can substitute for y and the equation become:
x2+x(-2x)+2(-2x)2=11
and (if I do the arithmetic correctly!) this is
(1-2+8)x2=11 so that x=+/-sqrt(11/7). At points where the
tangent line is horizontal, we know that y=-2x, so that the two points
of contact on the top and the bottom are (sqrt(11/7),-2sqrt(11/7)) and
(-sqrt(11/7),2sqrt(11/7)). These points are approximately
(1.254,-2.508) and (-1.254,2.508) and to me this looks approximately
correct compared to the pictures.
What about the points of contact of the bounding box on the left and
the right? Well, at those points the tangent line is vertical. It has
no slope. We have a formula for the slope of the tangent line:
dy/dx=-(2x+y)/(x+4y).
The formula doesn't work when the bottom, the denominator, is 0. That
occurs when x+4y=0 of x=-4y. If I insert this value of x in the
equation x2+xy+2y2=11, I'll get
(-4y)2+(-4y)y+2y2=11. Again, if I do the
arithmetic correctly, this means 14y2=11 so that
y=+/-sqrt(11/14). Since x=-4y the left and right touching points are
(-4sqrt(11/14),sqrt(11/14)) and (4sqrt(11/14),-sqrt(11/14)). These are
(-3.545,.886) and (3.545,-.886). Again, this looks about right to me.
QotD Write an equation for a line tangent to the
ellipse x2+xy+2y2=11 at the point (1,2).
(We checked by substituting that (1,2) was on this ellipse!) What's
needed is a point and a slope for the line.
HOMEWORK
Please read 3.5 and 3.6 and hand in these problems on Thursday:
3.5 24, 31, 48a, 53
3.6: 4, 7, 34, 39
CHIPCO
INVESTMENT DOLLARS & PRODUCTION
Capital Invested Chips produced Marginal chips produced
$ in millions 1,000's of units 1,000's of units per
millions of $'s
200 3,000 .23
300 3,040 .28
400 3,070 .42
500 3,100 .78
600 3,190 .31
CHIPCO
SALES & PROFITS
Chips marketed Profit gained Marginal profit
1,000's of units $'s in millions Millions of $'s per
1,000's of units
3,000 1.2 .03
3,050 2.8 .02
3,100 3.6 .05
3,150 4.9 -.01
3,200 5.1 .02
We decided that the pair of numbers 400 3,070 referred to the following phenomenon: if the corporation invests 400 million dollars in capital (building a factory, furnishing it, hiring and training people, etc.) then 3,070,000 chips can be produced.
Then we tried to understand the third column. The word "marginal" is
used in a fairly technical sense, although the use is common in
economics. In the first table above, it refers to the approximate
amount that chip production would increase per each million dollars of
increase in capital investment. Therefore, for example, if $302
million were invested, then (according to this model) chip production
would be 3,040,000 (chip production at the $300 million level)
plus .28(2)(1,000) chips. The 2 comes from the
additional millions of dollars of capital. The 1,000 comes from the
units used for chip production. The .28 is this "marginal"
quantity. In the first table, the marginal quantity is therefore the
approximate amount ![]() P/
P/![]() C, relating the change
in chip production to the change in capital investment. It is
sort of a slope, or, more likely, sort of a derivative: indeed, the
use of "marginal" in economics usually means a derivative. In this
model, if $297 million were invested, the approximate expected chip
production would be 3,040,000 (again, chip production at the $300
million level) plus .28(-3)(1,000). The novelty
here is the use of the minus sign, since the capital investment is
decreasing rather than increasing.
C, relating the change
in chip production to the change in capital investment. It is
sort of a slope, or, more likely, sort of a derivative: indeed, the
use of "marginal" in economics usually means a derivative. In this
model, if $297 million were invested, the approximate expected chip
production would be 3,040,000 (again, chip production at the $300
million level) plus .28(-3)(1,000). The novelty
here is the use of the minus sign, since the capital investment is
decreasing rather than increasing.
The marginal production may vary because at different levels, maybe
different new machines have to be purchased, or different types of
employees need to be hired and trained, etc. Things aren't simple.
I hoped that students would recognize the third column of the table
was actually list of values of the derivative of production with
respect to the "independent variable", capital invested.
The second table describes a similar phenomenon, here connecting the
chip amount, C, with the profit derived from these marketing and sale
of these chips. For example, the profit derived from the sale of
3,000,000 chips (the first line of the second table) is $1.2
million. If we now look at the third column, the model predicts a
marginal profit of .03 (in the given units). Using this, if 3,010,000
chips are marketed (that's 10 more 1,000 units of chips) the
additional profit would be .03(10) million dollars, or $300,000. And
if only 2,970,000 chips were marketed, then the profit would be 1.2
million+(.03)(-30)(1,000)million. (I think I got all the units
correct.) The third column gives ![]() P/
P/![]() C for various
amounts of chip marketing: the change in profits compared to the
change in chips marketed. One of the numbers in the third column is
negative: the -.01 at the 3,150 level. Is that realistic? I tried to
make a metaphor with a much more modest "business". I could deliver
newspapers every morning. Say that I have 100 customers, located on
five consecutive blocks of one street. It is easy and efficient for
newspaper delivery: the costs are low. What if I get 1 additional
customer? Well, if the customer lives "across town", with a half-hour
of driving needed, taking on this additional business will actually
probably cost money. There may be reasons that the business is
desired, but on a direct basis the incremental profit is negative
rather than positive.
C for various
amounts of chip marketing: the change in profits compared to the
change in chips marketed. One of the numbers in the third column is
negative: the -.01 at the 3,150 level. Is that realistic? I tried to
make a metaphor with a much more modest "business". I could deliver
newspapers every morning. Say that I have 100 customers, located on
five consecutive blocks of one street. It is easy and efficient for
newspaper delivery: the costs are low. What if I get 1 additional
customer? Well, if the customer lives "across town", with a half-hour
of driving needed, taking on this additional business will actually
probably cost money. There may be reasons that the business is
desired, but on a direct basis the incremental profit is negative
rather than positive.
Of course the validity of such models can certainly be criticized, but
I really wanted to show you these tables to explain what's in the next
paragraph.
The two tables linked together describe a complicated phenomenon. First we "input" capital, M (M is for money), which produces C, a certain number of chips. Then the chips are marketed (and sold, hopefully!) to obtain a certain amount of profit, P. Here we have a composition of functions. For example, suppose we were asked how much profit there is if we put in M=500 million dollars. From the first table we read off C=3,100,000 chips, and from the second table we can then see that P will be 3.6 million dollars.
I hoped that this was all fairly clear. Now I asked what I thought was
a difficult question. Suppose we increase M from 500 million dollars
to, say, 503 million dollars. What will the model predict the likely
profit will be? We can trace this if we are sufficiently alert. The
first marginal quantity we need to consider is ![]() C/
C/![]() M. For M=500 million dollars,
this is .78. So the new chip production is old chip production +
increase in chip production, and this will be
3,100,000+(.78)(3)(1,000). Now let us consider the chip/profit
table. With C=3,100,000, we see that profit is supposed to be 3.6
million. But we are changing C by adding on the (relatively small)
amount of .78(3)(1,000). The relevant marginal quantity here is
M. For M=500 million dollars,
this is .78. So the new chip production is old chip production +
increase in chip production, and this will be
3,100,000+(.78)(3)(1,000). Now let us consider the chip/profit
table. With C=3,100,000, we see that profit is supposed to be 3.6
million. But we are changing C by adding on the (relatively small)
amount of .78(3)(1,000). The relevant marginal quantity here is ![]() P/
P/![]() C, on the row where C is
3,100(,000). The marginal amount here is .05, so that the new profit
will be the old profit (3.6 million) plus (.05)(.78)(3)(1,000) million
dollars. The 3 comes from perturbing the capital investment. The 1,000
comes from my weird units. The really interesting stuff is (.05)(.78):
indeed, this represents the marginal profit as capital invested
changes, when the capital investment is 500 million
dollars. Symbolically, the multiplication might make sense written
this way:
C, on the row where C is
3,100(,000). The marginal amount here is .05, so that the new profit
will be the old profit (3.6 million) plus (.05)(.78)(3)(1,000) million
dollars. The 3 comes from perturbing the capital investment. The 1,000
comes from my weird units. The really interesting stuff is (.05)(.78):
indeed, this represents the marginal profit as capital invested
changes, when the capital investment is 500 million
dollars. Symbolically, the multiplication might make sense written
this way:
So theP
P
C --- = --- ---
M
C
M
Further comments One student remark was quite clever and
exposed a weakness of the method used. For example, the marginal
cost of chip production at the level M=200 is .23 and at M=300 it is
.28. What do we know about the marginal cost at, say, 350? In my
examples, I was very careful to consider only changes which were quite
small compared to the original quantity. I would not want to use these
ideas far away from the known data. I would need to rethink my
model.
It is easy to get interesting compositions and rates of change in
biology. I remarked that the transmission of influenza through humans
and other species (birds, pigs) provides very interesting examples,
although I think the biology is more difficult to explain than the
economics behind the tables above.
I've tried to present heuristic evidence that would allow us to believe the chain rule.
/heuristic/ adj. 1. allowing or assisting to discover. 2. [Computing] proceeding to a solution by trial and error.The Chain Rule Suppose that f and g are differentiable functions. Then F(x)=f(g(x)) is differentiable, and F´(x)=f´(g(x))·g´(x).
The balance of the lecture was devoted to using the chain rule with
functions defined by formulas.
There is a correct proof of the chain rule in the book. My first
example was something like this (about as simple as I could
imagine):
If F(x)=(x2+7)300, what will F´(x) be? Success
here probably will result from recognizing that the chain rule
applies.
If F(x)=f(g(x)), then g(x) is x2+7 so g´(x)=2x, and f(x) is
x300 so f´(x)=300x299. Thus
F´(x)=f´(g(x))g´(x)=f´(x2+7)(2x)=300(x2+7)299(2x).
Whew!
Alternate strategy You could take the summer off and
"expand" (x2+7)300. It is only a polynomial of
degree 600. And then it will be easy to differentiate this as a sum of
constants multiplying monomials. That's it: take the summer off and
report back next fall on this method. Be sure to get everything
correct.
But now comes the realistic comment. Almost no one ever bothers to
write all of the intermediate steps. That is, in practice
very few f's and g's are actually identified. What happens is that
people see and differentiate the outside most function (f above), put
in the inner function (g) in that derivative, and then multiply by
g'. For example, consider sin(ex+x2). What is
its derivative? The outside function is sine, whose derivative is
cosine. So I begin by writing
cos(what's inside)·the
derivative of what's inside.
The result is
cos(ex+x2)·(ex+2x).
This expression is
a formula for the derivative of
sin(ex+x2). Again, I urge you to consider the
significance and necessity (!) of appropriate parentheses in
these expressions. The "argument" of cosine is ex+x2
and the cosine expression is then multiplied by the expression
(ex+2x).
 I did several other examples, included repeated composition:
I did several other examples, included repeated composition:
If F(x)=cos(e37x2), then
F´(x)=[-sin(e37x2)](e37x2)37·2x.
Here I see F(x) as a composition of three functions. The outermost
function is cosine, whose derivative is -sine. The next function is
"e-to-the", which is what I think when I see the exponential function
written in its usual form. The derivative of e-to-the is e-to-the. The
innermost function is 37x2 which has derivative
37·2x. Maybe using the Chain Rule is like peeling an
onion, layer after layer, and each layer when "peeled"
(differentiated?) contains a copy of the contents of its unpeeled
self. Sigh. Sometimes metaphors are really silly. (Why are you crying?
I don't always cry when I peel an onion.)
QotD What is the derivative of
[sin(x2)]100? I emphasized
that this was not a situation where the 100 and the 2 could
be combined.
This is a triple compositon, but don't worry about it: just handle
each layer as you see it.
The derivative is 100[sin(x2)]99·[-sin(x2)]·2x.
I returned the exam together with an answer sheet. I remarked that detailed statistics and discussion of partial credit can be read here. I also said that about 40% of the course has gone by, and the next 60% would be denser and more technical. Although I believe that the grading is not perfect (I'd check additions first!) my suggestion for treatment of suspected errors in grading has two parts: first read through the partial credit treatment on the web and see if the scoring of your paper was consistent with that, and then if needed, report the suspected errors to me.
HOMEWORK
The material of this lecture is in section 3.5, and certainly the
Chain Rule is the most important differentiation rule. You need to
practice the Chain Rule a great deal. Here is the first time I've made
this suggestion to you: do every problem suggested. If you still can't
get it, do more problems!
Also, the WeBWorK assignment is due tonight.
ATTEND RECITATION!
I have asked Mr. Jin to discuss some matters relating to derivatives:
graphs and certain computations. After his discussions I hope that he
will give a quiz. The material I have suggested to him relates well to
certain exam questions which I have already graded. I was not able to
give
full credit to many students on these problems. Therefore you should
attend recitations on Thursday, and learn from him, please.
Rectilinear motion
 This means motion in a straight line. I'll try to use traditional
notation for this stuff. I'll consider the motion of a bug on a
straight line, here drawn horizontally. The position of the bug at
time t will be s(t). There are now a whole bunch of definitions.
This means motion in a straight line. I'll try to use traditional
notation for this stuff. I'll consider the motion of a bug on a
straight line, here drawn horizontally. The position of the bug at
time t will be s(t). There are now a whole bunch of definitions.
 After a request in this diary for a good New Jersey bug, Mr. Paul Remelgado gallantly suggested the
Brown Marmorated Stink Bug (Halyomorpha halys) as
somehow particularly appropriate for these pages. Perhaps this is
understandable. How wonderful is Cook College, and how wonderful are
its students!
After a request in this diary for a good New Jersey bug, Mr. Paul Remelgado gallantly suggested the
Brown Marmorated Stink Bug (Halyomorpha halys) as
somehow particularly appropriate for these pages. Perhaps this is
understandable. How wonderful is Cook College, and how wonderful are
its students!
Suppose that the position of Fred at time, t, is given by
s(t)=t2-2t.
Question #1 What is the average velocity of Fred from t=5 to
t=7?
Answer s(7)=72-2·7=35, and
s(5)=52-2·5=15. So the average velocity in the
interval from 5 to 7 is (35-15)/(7-5)=10.
Question #2 What is the (instantaneous) velocity of Fred at
time t=6?
Answer Since s(t)=t2-2t, ds/dt=2t-2, and when t=6,
this is 2·6-2=10. I felt really silly when the answers to these
first two questions were the same, and I hope people realize that the
equal answers were just coincidences, and equality won't always be
correct.
Question #3 How far does Fred crawl from time t=-10 to time
t=10? (Fred is a remarkable and immortal bug, and travels from
-infinity time to +infinity time.)
Note This is a very tricky question.
Answer Well, I first computed the positions of Fred at both
times. s(10)=102-2·10=80 and
s(-10)=(-10)2-2·(-10)=120. One guess might be that
we could compute the requested quantity from the numbers 120 and
80. This is not correct! The answer would just be the net
change in position of Fred from -10 to 10. It would not necessarily
be the total distance that Fred crawls in the time interval [-10,10].
Fred's path is much more dynamic than that!
Digression on velocity
Velocity has a sign and a magnitude. The sign describes the direction
of travel. Conventionally (as I've done in the accompanying pictures)
+, plus or positive velocity, means that the bug is moving from
left to right. The opposite, -, minus or negative velocity,
means the bug is traveling right to left. The magnitude of the
velocity, the speed, tells me how fast the bug is moving. (The
speedometer on your car goes up when you move either forward or
backward. My car doesn't have a +/- sign to tell me, on the
speedometer, which direction that the car is moving.)
Question #4 When is Fred moving to the left? (And the right,
etc.)
Answer Since ds/dt=2t-2, Fred moves to the left when 2t-2<0,
so that 2t<2 or t<1. So for times less than 1, Fred moves
left. Similarly, Fred moves right for times greater than 1. Fred's
motion is not very complicated.

Back to question #3
Between -10 and 10 Fred moves to the left from -10 to 1, and then to
the right from 1 to 10. The key positions for Fred's motion are
s(-10)=120, s(1)=12-2·1=-1, and s(10)=80. So Fred
moves left 121 units, and then right 81 units. The total distance that
Fred moves seems to be 121+81=202. The picture above attempts to show
more kinetic (!) view of Fred's motion, with the blue arrows trying to show Fred's path.
As far as I know, the simplest way to answer questions of this type is
to first find all possible turning points, that is, times when
s´(t)=0 and see how they affect the motion during the time
interval of interest.
While standing in a tree ...
I quoted a formula from p.133 in the text:
h(t)=-(1/2)gt2+v0t+s0
Here h(t) is supposed to be the "formula for the height of a
projectile", and g is the acceleration due to gravity, v0
"is the initial velocity" and s0 "is the initial height.
Now, on Earth, if distance is measured in feet (almost obsolete!) and
time is measured in seconds, then g is about 32. Height is measured so
that more + means higher. The g term has a negative sign
because the gravity pulls an object down.
Problem statement Suppose you are standing in a tree on Earth
50 feet above ground, and you throw a ball straight up in the air at
30 feet per second.
Question #1 What is a formula giving the height of the ball for
later times?
Answer Well, s0=50 and v0=30. These
numbers have positive signs because you are in a tree above
ground and because you throw the ball up: those words encode
the signs and you can't do the problem correctly without paying
attention to them. g is 32 because the tree is on Earth. So I use the
textbook's equation and obtain
h(t)=-16t2+30t+50
Question #2 What is the highest that the ball gets, and what
time does it get that high?
Answer The velocity is positive while the ball is on its way
up, and is negative while the ball is on its way down. When the ball
has velocity=0, it is (metaphorically!) stopped, at the tippy-top of
its path. So we need to find when the velocity is 0. Since
h(t)=-16t2+30t+50 we know v(t)=-32t+30, and this is zero
when t=30/32. How high is it at that time? Well, we plug the time into
the formula giving the height:
h(30/32)=-16(30/32)2+30(30/32)+50 and that's the answer.
Question #3 At what time does the ball hit the ground?
Answer The ball hits the ground when the height is 0. That
occurs when -16t2+30t+50=0. I would not waste my
time trying to factor such a silly quadratic. I would just use the
quadratic formula. That tells me the roots are at
[-30+/-sqrt((30)2-4(-16)(50))]/(2·-16).
But which root is the hitting time? We need the positive root. The
bottom of this giant fraction is negative, so we want the top to be
negative also. But the top will be negative when we choose the minus
sign in front of the square root, so the unique answer is
[-30-sqrt((30)2-4(-16)(50))]/(2·-16).
In these exercises I am trying to behave as you should during a Math 135 exam (see the discussion which follows for more detail). In fact, if this really were somehow a problem I needed to solve in the real real world, I would use a calculator freely at various times to approximate the answers to questions 2 and 3. Math 135 is not the real world.
I am writing the following part of this diary entry before class.
WeBWorK
The closing time for WeBWorK assignments will now be 9 PM on
Friday beginning with next week (set 5). This will take into account
our exam, and the subsequent delays in the lectures.
My grading on an exam ...
The QotD from the previous class meeting provides an excellent
opportunity for me to explain in some detail how I will grade exam
problems.
The question was:
Use calculus to find the exact coordinates of the bump on the graph of y=xex.
The setting of the question included a sketch of the graph, and the sketch clearly displayed a "bump" in the third quadrant, where both coordinates of the point would be negative. I'd classify this question as one of medium difficulty for a Math 135 exam, and I'd probably give it a value of 7 or 8 points on a 100 point exam.
What would I look for when I graded this question? I would want recognition by the student that the bump occurred when the tangent line was horizontal. An effort to locate the point would therefore involve setting the derivative equal to 0. But let me be more precise:
| Answers to exam questions must be exact, not calculator approximations. For example, the number 1.732050808 is not a correct answer when the right answer is actually sqrt{3}. |
I assess this question as one of medium difficulty on a Math 135 exam. My reading of the QotD solutions suggests that about half of the students would have received full credit if their answers were graded. Other students would get no points. Also, I will generally insist that students do "my" problem, the one whose statement appears on the exam. Substitutes or simplifications are not acceptable.
Further ...
I've gotten a WeBWorK report on the first four problem
sets. Some students have done little or no work. WeBWorK
scores will be a component of course grades. Here is what one of my
wise colleagues said to me:
| You don't give grades. You report the grades students have gotten. |
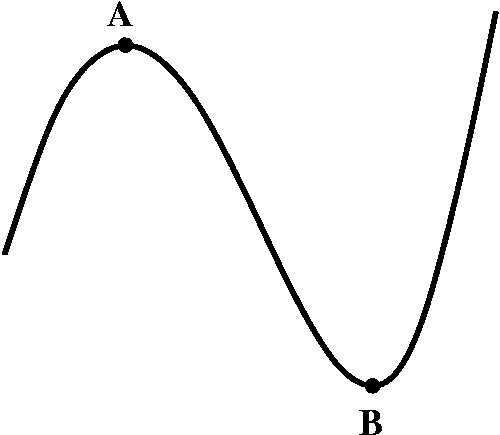
Today's QotD
The graph of y=2x3-3x2-12x+1 is as displayed.
What are the coordinates of A and B?
Comment Yeah, I purposely (and meanly!) did not draw any axes. So part of the problem is to figure out which point has which coordinates. And I even wrote it this way on an exam: another irritation!
I will attempt to have students' solutions graded and available at the review session Monday evening.
HOMEWORK
Study for the exam.
Diary entry in progress! More to
come.
| ... you may not use calculators with QWERTY keypads or symbolic manipulation capabilities. In particular, the TI-89, the TI-89 Titanium, the TI-92 and the Voyage 200 will not be allowed on any exam. |
We will continue to explain how to write (generally, less simple!) formulas for the derivatives of functions which are defined in terms of (simple) formulas.
Opening exercises
| Function | Its derivative |
|---|---|
| 17x44+4sqrt(x)-98 | 17·44x43+4(1/2)x-1/2+0 |
| Comment Hey, there are certain traditional ways to indicate some powers. "Sqrt" (or, better, the usual square root sign) means the half power and should be treated that way for differentiation purposes. | |
| 44x44+9/x2 | 44·x43+9(-2)/x3 |
| Comment And another way powers of x can be written is with a division sign: /. So +9/x2 is "really" 9x-2 and that's the way we'll understand it for differentiation purposes. | |
|
[-5+2x4] ------------------- 5x55+e(Pi)sqrt(2)] |
[0+2·4x3][55x55+e(Pi)sqrt(2)]-[-5+2x4][55·x54+0] ----------------------------------------------- [55x55+e(Pi)sqrt(2)]2 |
| Comment It can be easy to get lost in differentiating a quotient. And the other tricky thing here is to realize that the mess e(Pi)sqrt(2) is just a constant, and its derivative is 0. | |
A quote
L. Kronecker, a 19th century mathematician, declared
that
"God created the integers, all else is the work of man."
This is the standard English statement. I searched on the web and I
can't find the original German. I think this statement is relevant
because, especially in what follows, we will see many coincidences
which will make finding derivatives easy. These aren't
coincidences. These computations have all be arranged over a
few centuries by people so that other people will be able to compute
more easily. So please: There are no coincidences! Everything has
been prepared!!
Sine In all this I cannot constantly review the geometry and algebra of the trig functions. This was done in a rapid way earlier in the course. Also I hope you will recall for computational examples the magical values of various trig functions. I tried to draw an accurate picture of sine and then discussed what properties the derivative of sine would have.
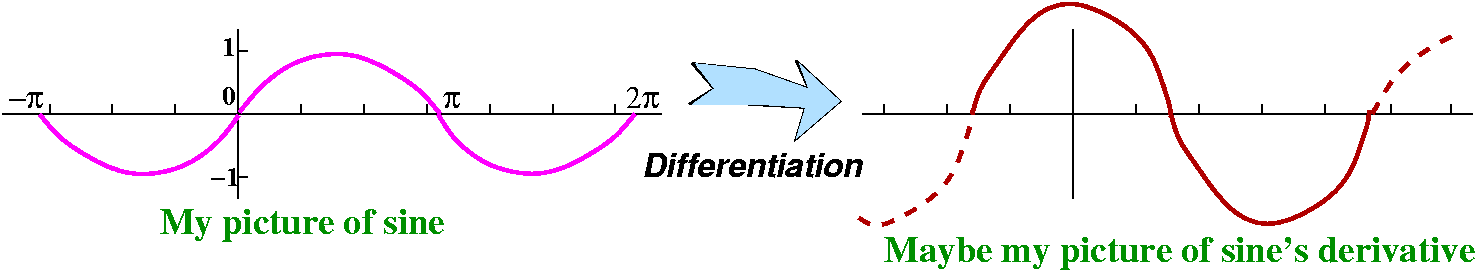
The derivative of sine is cosine.
In the textbook, something like the following is done when f(x)=sin(x):
sin(x+h)-sin(x) sin(x)cos(h)+sin(h)cos(x)-sin(x)
----------------- = ---------------------------------- = PIECE #1 + PIECE #2
h h
where
sin(h)
PIECE #1 = cos(x) --------
h
and as h-->0 this --> cos(x)·1 because we arranged it this
way when we decided to use radian measure! Also,
cos(h)-1
PIECE #2 = sin(x) ----------
h
If we multiply this top and bottom by cos(h)+1, the result on top is
[cos(h)]2-1 which is [sin(h)]2. Then
[sin(h)]2 sin(h) 1
PIECE #2 = sin(x) ------------- = sin(x) -------- sin(h) ------------
h [cos(h)+1] h [cos(h)+1]
Now as h-->0, I claim:
sin(h) 1
sin(x)-->sin(x); ------ -->1; sin(h)-->0; ----------- --> 1/2.
Nothing happens! h [cos(h)+1]
So the result is sin(x)·1·0·1/2=0.
Cosine and the other guys You can play the same game with cosine as with sine. Here is an impression of what the picture might look like:
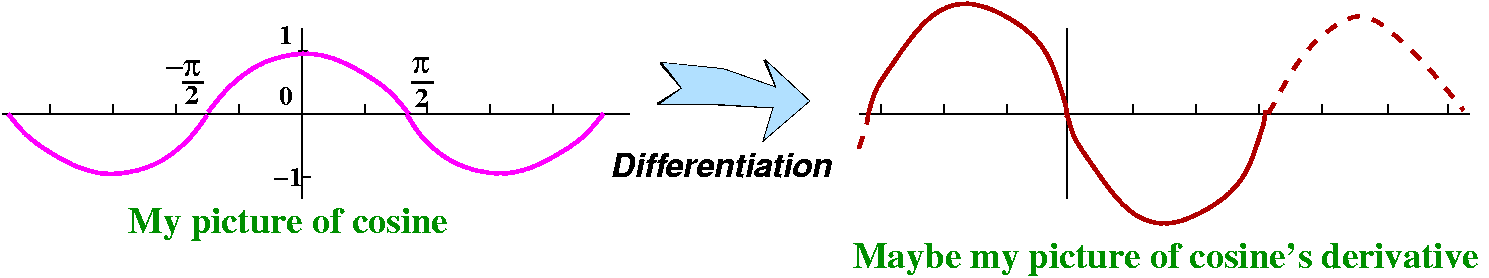
If you look closely at the picture, there is a loose minus sign around. This we can't get rid of: I'm sorry.
The derivative of cosine is -sine.
There are the derivatives of other trig functions. Mostly I don't know
these formulas. O.k.: I do remember the derivative of tangent:
Since tan(x)=sin(x)/cos(x), I can use the Quotient Rule, and
then
tan´(x)=[cos(x)·cos(x)-sin(x)·{-sin(x)}]/[cos(x)]2. The top can be recognized (?) as cos(x)2+sin(x)2 which is 1. So the derivative of tangent is 1/[cos(x)]2. The book calls this [sec(x)]2.
The derivative of tangent is secant squared.
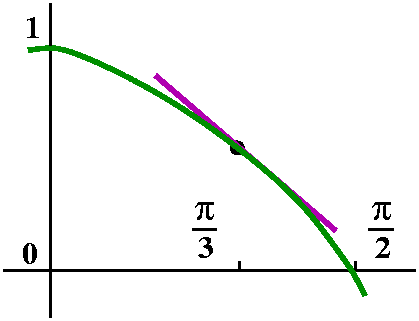 An example
What is the equation of the line tangent to y=cos(x) when
x=Pi/3? We need a point and a slope.
An example
What is the equation of the line tangent to y=cos(x) when
x=Pi/3? We need a point and a slope.
Point Well, f(x)=cos(x), and cos(Pi/3)=1/2 (yes, this is one of
the magical values you should know). Therefore the point (Pi/3,1/2) is
on the graph, and the tangent line we're looking for goes through it.
Slope If f(x)=cos(x), then f´(x)=-sin(x). This derivative
gives the slope of the tangent line. So the specific slope we
are looking for is -sin(Pi/3). This is -sqrt(3)/2 (more magic).
The answer (y-1/2)=[-sqrt(3)/2](x-Pi/3).
Please notice that the picture "confirms" a negative slope on the
tangent line.
Another example so students could do some work!
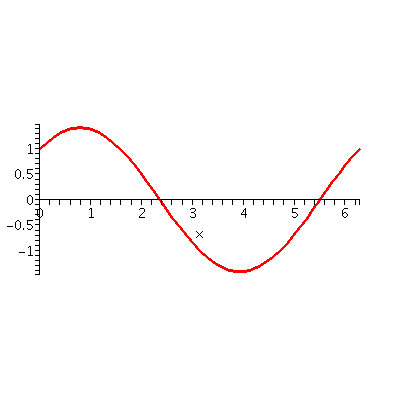 I asked what the graph of y=sin(x)+cos(x) looked like and glared at
the students menacingly. I also asked, yet again, what century we
were in. After what seemed like a long, l-o-n-g, l--o--n--g time to
me, some people got the idea and decided to use their graphing
calculators. I expected, I hoped, I wanted people to get a picture
similar to the one displayed to the right, which was done by a
machine.
I asked what the graph of y=sin(x)+cos(x) looked like and glared at
the students menacingly. I also asked, yet again, what century we
were in. After what seemed like a long, l-o-n-g, l--o--n--g time to
me, some people got the idea and decided to use their graphing
calculators. I expected, I hoped, I wanted people to get a picture
similar to the one displayed to the right, which was done by a
machine.
Question What is the point on the top of the first bump?
Well, the bump's top has a horizontal tangent, an x where
f´(x)=0. So let's compute this derivative. If f(x)=sin(x)+cos(x),
then f´(x)=cos(x)-sin(x). Where is this equal to 0? Well, then
cos(x)-sin(x)=0 or cos(x)=sin(x) or (dividing by cosine) we get
tan(x)=1. Hey: yet another magic value! (Is that happiness?) Well, if
tan(x)=1 then x=Pi/4. But what is that point? To answer that we
need to figure our the coordinates, an ordered pair, of the point.
The bump is on y=sin(x)+cos(x). So we need to "stuff in" x=Pi/4 into
the original function, sin(x)+cos(x). The value we get is
sin(Pi/4)+cos(Pi/4), and (magic, magic ...) this is 2/sqrt(2) or just
sqrt(2). The coordinates of the bump are (Pi/4,sqrt(2)). This does
look approximately correct on the graph.
Exp Well, if f(x)=ex, then f(x+h)=ex+h. Now look:
f(x+h)-f(x) ex+h-ex exeh-ex eh-1
------------- = -------- = --------- = ex ------
h h h h
But we chose the number e exactly so that as
h-->0, [eh-1]/h-->1. This makes things much
easier.
The derivative of the exponential function is the exponential function: (ex)´=ex.
So (yawn) the 37th derivative of ex is ex. So ex, called the exponential function, should truly be the favorite function of the lazy individual.
Flipping exp to get ln
Suppose we look at y=ex. On this graph is a point whose
coordinates are, not surprisingly, (x,ex). There's a line
tangent to y=ex at this point, and this line has slope
ex since we previously arranged all this. The slope is
OPPOSITE/ADJACENT because slope is a tangent of an
angle.

Now ... here's the hard or amusing or whatever idea: take this
picture: coordinate axes, curve, tangent line, and flip it: pick the picture up and flip it
over the diagonal line y=x. The coordinate axes get interchanged. The
exponential curve gets changed to another curve which we will
identify. The tangent line is flipped but still remains tangent to the
flipped curve. Algebraically, what we are doing is exchanging the
first and second coordinates of each point. So the point
(x,ex) becomes (ex,x). Hey: if the ex
is a new variable, say, w, then since w=ex, ln(w)=x, and
the flipped point (ex,x) wonderfully becomes the point
(w,ln(w)). The tangent line's slope flips (because ADJACENT
and OPPOSITE get interchanged). The flipped line's slope is
1/ex which is 1/w in terms of w. Hey: the slope of the
tangent line is the derivative.
The derivative of the natural log function, ln(x), is 1/x: (ln)´(x)=1/x.
An example Find the equation of a line tangent to y=3ln(x)-x when x=e. Now f(x)=3ln(x)-x. Since f(e)=3ln(e)-e=3-e. The tangent line goes through the point (e,3-e). The slope of the tangent line comes from the derivative. Now f´(x)=3(1/x)-1 (I am using the differentiation rules and the derivative fact stated above). Therefore the slope is f´(e)=[3/e]-1 and an equation for the tangent line is (y-[3-e])={[3/e]-1}(x-e).
Yet another picture request
I asked what the graph of y=xex looked like. Here some
people had learned and took out their calculators. Although I
really like these electronic helpers, I will also admit that they need
to be used with some care.
| Here's a picture of y=xex with both x and y in the interval -20 to 20. It is difficult to see much "fine structure" at this scale. I guess (for my purposes) this is a bad picture. | 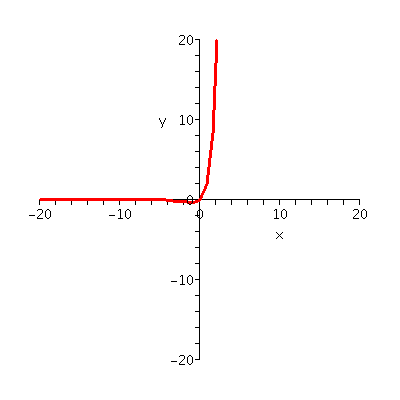 |
| Now this is a picture of y=xex with both x and y in the
interval -3 to 3. In this picture the curve, which goes through the
point (0,0), has a sort of bump in the third quadrant.
The "correct scale" to look at a curve is not always clear, and this can be a problem both in classroom examples and in real-life applications. Experiments may be necessary. | 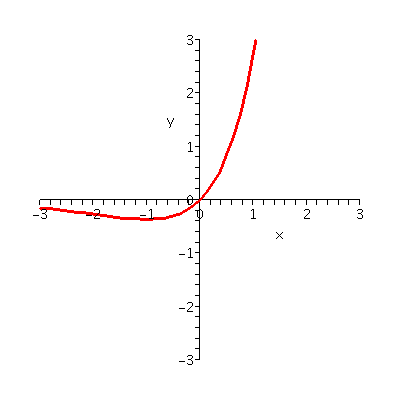 |
QotD Use calculus to find the exact coordinates of the bump on the graph of y=xex.
What should you have gotten out of this lecture?
There's lots of stuff here. But let's cut to the chase, the
bottom line, summarize, winterize, whatever ... what should you
remember?
| First, remember this: | |
|---|---|
| Function | Derivative |
| sin(x) | cos(x) |
| cos(x) | -sin(x) |
| tan(x) | (sec(x))2 |
| ex | ex |
| ln(x) | 1/x |
| Comment If you really need the derivative of secant (and this to me is very unlikely outside of a calculus course!), you can look it up or, heck, since secant=1/cosine, use the quotient rule: sec´(x)=[0·cos(x)-1·(-sin(x))]/(cos(x))2 which is sec(x)tan(x) as books would tell you. | |
| And really remember this: | |
|---|---|
| If curve looks like: | then the derivative is |
 |
Tangent lines go up Tangent slopes are positive Derivative is positive |
 |
Tangent lines go down Tangent slopes are negative Derivative is negative |
 |
If the top is round ("differentiable") then the tangent line is flat Derivative is zero |
 |
If the bottom is round ("differentiable") then the tangent line is flat Derivative is zero |
| Comment This is what I'd like you to take from this lecture's discussion. These are extremely important qualitative properties of derivative. This is what people use in practice. I don't think many veterinarians or financial analysts need to compute tangent lines to sec(x), but many of these people must work with graphs and with data: when do these things increase, decrease, have maxima ("tops") and have minima ("bottoms"). This is the important stuff! | |
HOMEWORK
Please read the assigned sections of chapter 3 and do the suggested
homework problems Students should also look at the review material
for the first exam. The Thursday recitation will include a short quiz
on differentiation of formulas. Please hand in these problems:
3.2: 37, 43
3.3: 52, 54
|
lim f(x+h)-f(x) h-->0 ------------ = f´(x) h |
Hey: I am aware that in the text h is usually written ![]() x, but I
find that sometime confusing. It is supposed to indicate a small
amount of x, but the writing of two symbols is sort of weird.
x, but I
find that sometime confusing. It is supposed to indicate a small
amount of x, but the writing of two symbols is sort of weird.
The derivative is supposed to indicate the slope of a non-vertical tangent line. Geometrically, you should be able to "see" this, especially with the help of a graphing calculator and zooming in on the suggested point of tangency. Here are some pictures which might help.
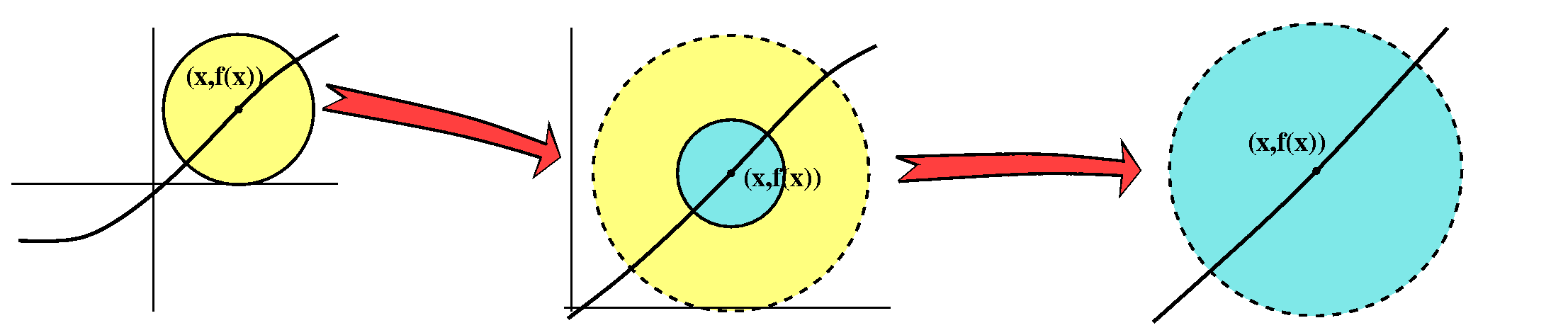
After a few zooms, the graph of the function should look more and more like a straight line through (x,f(x)), and the straight line will have slope equal to f´(x).
How to recognize from the graph whether the derivative
exists
Pick a point on the graph to investigate. Zoom in, and zoom in, and
zoom in. If what you get does not look like a (non-vertical)
line, that the derviative does not exist: the function is not
differentiable at that point.
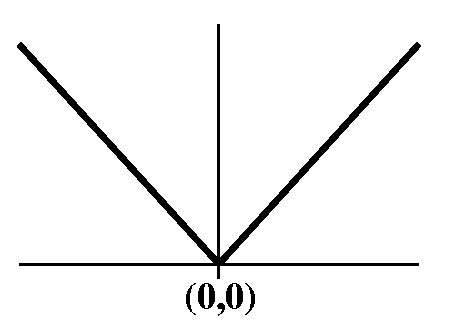 An example: absolute value
An example: absolute value
I hope that the graph of f(x)=|x|, the absolute value function, is
slightly familiar to you. It is a big V. Officially from the algebraic side, absolute
value is a piecewise -defined function, but I am interested here in
pictures. The point of interest on the graph of |x| is (0,0). If you
zoom in on it, the graph looks just the same: it is still a V. It never "straightens out" into a
line. So this function is not differentiable at x=0. The textboook
verifies algebraically that the limit defining the derivative does not
exist at x=0 ifyou prefer that approach. You should see, I hope, that
for non-zero x's, |x| is differentiable. Actually the derivative is 1
for x>0 and is -1 for x<0.
This is not such a weird example. The continuous tax functions we discussed in the second lecture have exactly the same problems. So Schedule X of form 1040 is continuous, but not differentiable. Indeed, the behavior of absolute value under magnification occurs in a variety of biological applications (the idea is that this picture is what's called scale-free: it occurs at any length).
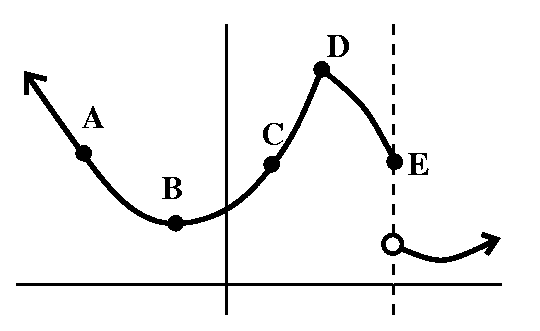 A problem
A problem
To the right is drawn a portion of the graph of y=f(x). Five points
on the graph are labeled: A, B, C, D, E. Here are some questions about
the function. My answers are below. Please try to get your answers
without looking at mine. Answers should have some reasoning supporting
them.
Questions
Some answers
As I remarked in class, I am filled now with trepidation, which was accurately defined by a student as worry, fear, concern. The algebraic density of the course is about to increase abruptly. You'll need to practice what we'll discuss a great deal. I will tell you how to find the derivative of many functions which are defined by simple algebraic formulas. I will not cover everything in the syllabus scheduled for today!
What's the derivative of ...
A wonderful aspect of our intelligence is pattern recognition. But
certainly we need to train ourselves when we see new things. So here
are some new things.
... x7
Now [f(x+h)-f(x)]/h is [(x+h)7-x7]/h. What can we
do? I guess I could multiply out the (x+h)7 but I
prefer to be a bit more lazy. I'll think about it:
seven times
(x+h)(x+h)···(x+h)
Now one thing I certainly get from this product of sums, by selecting
the x term from each of them, is an x7. What else can I
get? Since I've already taken the product with all of the x's, the
other terms will have at least one h. For example, I could select the
h in the first partentheses, and then x's from all the others: this
gets me hx6. But I could get something similar by selecting
one h from the second term and x's from the first and all the
others. In fact, I can select one h seven ways. So I will get
7hx6. I'm not done yet, of course (if you know the Binomial
Theorem, you can see the rest of the swamp). I don't want to
write all of the other terms. I do know that there will be at least
two h's in all the rest. So in fact, here is what I will write:
(x+h)7=x7+7hx6+h2JUNK
It will turn out that the term I called JUNK doesn't matter
very much. Why is that? Well, I want to understand the behavior of
[(x+h)7-x7]/h as h-->0? So that is:
x7+7hx6+h2JUNK-x7 7hx6+h2JUNK
------------------ = ------------ = 7x6+hJUNK
h h
First the x7's cancel, and then the h's drop out. Now we
can see that as h-->0, this easily -->7x6.The derivative of xn is nxn-1
... x7+x32
Now [f(x+h)-f(x)]/h is
[(x+h)7+(x+h)32-(x7+x32]/h.
What should we do? Always first try to be lazy. Look:
[(x+h)7+(x+h)32-(x7+x32)] [(x+h)7-x7]+[(x+h)32-x32]
-------------------------- = -------------------------
h h
Now stop writing and starting thinking. There are two pieces to this,
and one you should recognize, because we just analyzed it. It comes
from the definition of derivative applied to x7. The other
piece comes from the definition of derivative applied to
x32. I bet that after acres of algebraic manipulation, the
result will be the sum, that is, 7x6+32x31. This
is actually correct. You might worry, though, about whether algebraic
manipulation can be measured in acres. I am not sure.
If f(x) has derivative f´(x) and g(x) has derivative g´(x), then the derivative of f(x)+g(x) is f´(x)+g´(x)
... products?
The situation with regard to products is much more subtle, and I tried
to motivate the answer by looking at some invented economic data.
| Category | This year | Next year |
|---|---|---|
| Cars per househould in the U.S. | 2.2 | 2.3 |
| Number of households in the U.S. | 93 million | 95 million |
If f(x) has derivative f´(x) and g(x) has derivative g´(x), then the derivative of f(x)·g(x) is f´(x)·g(x)+f(x)·g´(x)
If you still think this is too complicated, a simpler example may
convince you that the complexity is needed. The derivative of
x5 is 5x4. But
x5=x2·x3. Now x2 has
derivative 2x and x3 has derivative 3x2. How can
we construct 5x4 out of x2 and 2x and
x3 and 3x2? Well, the product rule (that's the
name of the result above) does the construction correctly:
5x4=2x·x3+x2middot;3x2.
... 17
If f(x)=17 for all x, then [f(x+h)-f(x)]/h is ... uhhhh ... 0. This is
almost embarrassing. The derivative of the function which is always 17
is 0 always. (Well, the tangent line is horizontal!)
The derivative of a constant is 0.
... 17x7
We could apply the product rule to computing the derivative of
17x7, and the result would be
0·x7+17·7x6. This sort of thing
arises so frequently (in, for example, polynomials) that we should
note it.
If k is a constant and f(x) is a differentiable function with derivative f´(x), then the derivative of kf(x) is kf´(x).
Many derivatives
If f(x)=5x8-9x2+38, then
f´(x)=5·8x7-9·2x+0. Wow! So
f´(x)=40x7-18x. We can take a derivative of this
derivative, and get
f´´(x)=40·7x6-18=280x6-18. And even
another (here people mostly use a different notation) to get
f(3)(x)=280·6x5. Etc. Well, etc. except that I can tell you that the
50th derivative of f(x) is 0. Can you see why?
The quotient rule, which describes the derivative of a quotient of differentiable functions, is almost silly, and it is complicated. Here it is:
If f(x) has derivative f´(x) and g(x) has derivative g´(x), then the derivative of f(x)/g(x) is [f´(x)·g(x)-f(x)·g´(x)]/(g(x))2
The derivative of (3x-7)/(4x2+2) is therefore
[3·(4x2+2)-(3x-7)(8x)]/(4x2+2)2.
We could find the second derivative and the third, but things would
get increasingly messy, very, very messy. The opportunity for error
would get large.
An equation for a tangent line
Suppose that f(x) and g(x) are differentiable functions, and the
following is known:
f(1)=2, f´(1)=3, g(1)=5, and g´(1)=-2.
What is an equation of the line tangent to the graph of y=f(x)g(x) when
x=1?
Since f(1)g(1)=10, the tangent line goes through the point (1,10). And
the derviative of f(x)g(x) at x=1 is
f´(1)g(1)+f(1)g´(1)=3(5)+2(-2)=11. So the slope of the
tangent line is 11. The equation therefore must be:
(y-10)=11(x-1).
QotD
Use the same data, and find an equation of the line tangent to
the graph of y=f(x)/g(x) when x=1?
Now when x=1, y=f(1)/g(1)=2/5. And the derivative of f(x)/g(x) when
x=1 is [f´(1)g(1)-f(1)g´(1)]/[g(1)]2, which is
[3(5)-2(-2)]/52=19/25. So an equation for the tangent line
is (y-[2/5])=[19/25](x-1).
HOMEWORK
Keep up! There's an exam coming!
Maintained by greenfie@math.rutgers.edu and last modified 2/13/2005.