Carl Friedrich Gauss worked in many different fields in mathematics and physics during his life, including astronomy. His work was highly influential in many areas [5,7].
Born in 1777 in Brunswick, Germany, Gauss quickly showed his ability in mathematics. He supposedly corrected his father's arithmetic when he was three [1,5]. When he was seven, he startled his teachers by being able to quickly sum up the integers from 1 to 100, by spotting that the sum was 50 pairs of numbers, each summing to 101 [5,7]. The Duke of Brunswick noticed him in 1792 and sponsored the rest of his education. Gauss attended the Collegium Carolinium from 1792 to 1795, where he independently discovered the binomial theorem, the arithmetic-geometric mean, and the prime number theorem (which he was not able to prove) [5].
In 1795, Gauss left Brunswick and entered the University of Goettingen. While there he discovered how to construct a 17-sided polygon with ruler and compass. Gauss left the university in 1798 without a degree. [1,5] In 1799, Gauss developed the concept of complex numbers and also submitted a dissertation to the University of Helmstedt providing a proof for the fundamental theorem of algebra. [1,5] This dissertation won Gauss a doctoral degree in abstentia. In 1801, Gauss completed "Disquisitiones Arithmeticae," a major volume on number theory. [1,2,3,5]
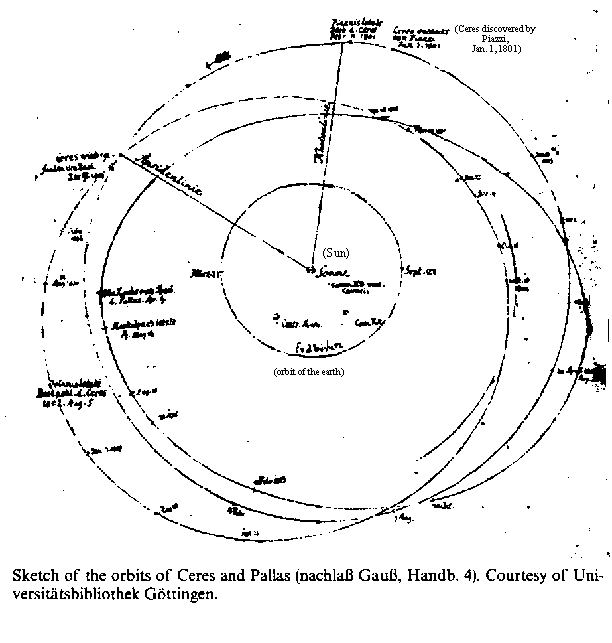
When Uranus was discovered in 1781, it was found to fit the Titius-Bode law. The only unexplained number left in the series would correspond to a planet between Mars and Jupiter. In 1800, an informal society was formed to find this missing planet, and Zach, the director of the Seeberg observatory and editor of the Monatliche Correspondenz, the main German astronomical periodical at the time, used his periodical to gain support for this effort [8,10]. Then,on January 1, 1801, the Italian astronomer Joseph Piazzi discovered a planetoid, working from an observatory in Palermo, Italy. This object, which he christened Ceres, was moving in the constellation Taurus. Astronomers were only able to observe the planetoid for 41 days, during which its orbit swept out an angle of only 9 degrees. Ceres was then lost to sight when its light vanished in the rays of the sun, and the astronomers could no longer find it. There was now a challenge of calculating Ceres' orbit using only the observations Piazzi made, so that astronomers would be able to sight Ceres when it reemerged. [1,5,6,8,10]
Zach published Piazzi's observations of Ceres in June of 1801. Most of the leading astronomers in Europe already knew of these observations when they were published, and were scrambling to determine its orbit. [1,6]
The main problem with calculating Ceres's orbit was a lack of precedent to draw upon [5]. The only possible precedent was William Herschel's discovery of Uranus in 1781. In the case of Uranus, however, astronomers were able to observe its position on many different nights, and could record many position changes of Uranus with respect to the Earth [11]. Astronomers also made the simplifying assumption that its orbit was circular, which luckily was nearly correct. The reigning method for calculating orbits assumed that the orbit of a planet was circular, and that the orbit of a comet was parabolic [5]; that is, in both cases the eccentricity of the orbit was taken as known. The general form of a planet's or a comet's orbit depends on its eccentricity, which is the measurement of the orbit's deviation from circularity. A circle's eccentricity is 0, and the eccentricity of a parabola is 1. The problem of Ceres's orbit was that since no one knew the shape of its orbit, it could only be assumed to be an ellipse, with eccentricity between 0 and 1. This case had been dealt with by Euler, Lambert, Lagrange, and Laplace, but they used difficult methods which did not allow for a complete determination of the orbit from observations (necessarily involving observational errors) over only a short period of time. Laplace, among many others, thought the problem would be unsolvable in this form. Apparently, the orbit of Ceres could not be determined accurately from the data, at least with known methods. [5]
At this point, Gauss had already worked with astronomical questions, such as the theory of the motion of the moon. At 18, Gauss had developed, but not published, his method of least squares, which made it possible to determine an orbit as long as it is assumed that it is a conic section [10]. He decided to work out a more useful method for determining orbits, and was soon ready [5,11]. Gauss differed from his contemporaries by avoiding any arbitrary assumption for the eccentricity of the initial orbit. His ellipse was based only on some of the available observations, without any additional hypotheses. The original computation was based largely on heuristic considerations. Gauss used methods similar to those used for the theory of the motion of the moon, especially the approximation of the elements of the orbit by finite parts of Taylor and trigonometric series. He also used the method of least squares to minimize the inevitable errors of observation [1,4,10].
Gauss first adopted Kepler's hypothesis that the motion of a celestial object is determined solely by its orbit. No information is needed about the mass, velocity, or any other details of the object itself. Gauss adopted a secondary hypothesis also, which was also derived from Kepler. It basically states that the orbit of an object that does not pass extremely close to another body in the solar system has the form of a conic section with its focal point at the center of the sun. Under these conditions, the motion of the object is determined by a set of 5 parameters, or elements, which specify the form and position of the orbit in space. Once the elements of an orbit are specified, the motion of the object is determined, as long as it remains in that orbit [11] (unperturbed by large planets such as Jupiter).
The elements of an orbit consist of the following: 2 parameters determining the position of the plane of the object's orbit relative to the Earth's orbit; the relative scale of the orbit; the eccentricity of the orbit or perihelial distance, the shortest distance from the orbit to the center of the sun; the relative "tilt" of the main axis of the orbit. In addition to these 5 parameters, a single time when the object was, or will be, in a particular point in the orbit is needed, so that its location at a given time can be computed [11].
Gauss had a total of 22 observations made by Piazzi over 41 days. The data from these observations consisted of a specific moment in time together with 2 angles defining the direction in which the object had been seen relative to an astronomical system of reference defined by the sphere of fixed stars. In principle, each of these observations defined a line in space, starting from the location of Piazzi's location at the moment of observation and directed along the direction defined by the 2 angles. Gauss had to make corrections for various effects such as the rotation of the Earth's axis, the motion of the Earth's orbit around the sun, and possible errors in Piazzi's observations or in their transcription [11].
The technical execution of Gauss's method is very involved, and required over 100 hours of calculation for him. His first tactic was to determine a rough approximation to the unknown orbit, and then refine it to a high degree of precision. Gauss initially used only 3 of Piazzi's 22 observations, those from January 1, January 21, and February 11. The observations showed an apparent retrograde motion from January 1 to January 11, around which time Ceres reversed to a forward motion. Gauss chose one of the unknown distances, the one corresponding to the intermediate position of the 3 observations, as the target of his efforts. After obtaining that important value, he determined the distances of the first and third observations, and from those the corresponding spatial positions of Ceres. From the spatial positions Gauss calculated a first approximation of the elements of the orbit. Using this approximate orbital calculation, he could then revise the initial calculation of the distances to obtain a more precise orbit, and so on, until all the values in the calculation became coherent with each other and with the three selected observations. Subsequent refinements in his calculation adjusted the initial parameters to fit all of Piazzi's observations more smoothly [11].
In September of 1801, Zach published several forecasts of the prospective orbit, his own and Gauss's among them; Gauss's prediction was quite different from the others and expanded the area of the sky to be searched [1]. Using Gauss's ephemeris for Ceres (astronomical almanac showing its predicted location at various times), astronomers found Ceres again between November 25 and December 31. Zach, on December 7, and then Olbers, on December 31, located Ceres very close to the positions predicted by Gauss. Between the discovery of Ceres in 1801 and the present day, over 1,500 planetoids have been identified, with Ceres remaining the largest [5,10]. While continually improving and simplifying his methods, Gauss calculated ephemerides for the new planetoids as they were discovered. When Olbers found Vesta in 1807, Gauss calculated the elements of its orbit in only 10 hours. His calculations of parabolic orbits were even faster, as is natural. He could calculate the orbit of a comet in a single hour, where it had taken Euler 3 days using the previous methods [5,6].
Gauss published his methods in 1809 as "Theoria motus corporum coelestium in sectionibus conicus solem ambientium," or, "Theory of the motion of heavenly bodies moving about the sun in conic sections." [1,2,3,5,6,11]. Gauss first wrote this work in German, but his well-known publisher, Perthes, requested he change it to Latin to make it more widely accessible (sic). In fact, the astronomical methods described in Theoria Motus Corporum Coelestium are still in use today, and only a few modifications have been necessary to adapt them for computers [11]. Gauss's determination of Ceres's orbit made him famous in academic circles worldwide, established his reputation in the scientific and mathematical communities, and won him a position as director at the Gottingen Observatory. [5,10]

Note (2006)
The last site is unavailable. At present the image used can be found at
http://webdoc.sub.gwdg.de/ebook/e/2005/gausscd/html/kapitel_astro_ceres.htm.
It is referenced as follows
Skizze der Bahnen der Kleinplaneten Ceres, Pallas und Vesta.
(In: "Astronomische Untersuchungen und Rechnungen vornehmlich über die Ceres Ferdinandea". 1802.)
SUB Göttingen: Cod. Ms. Gauß Handbuch 4, Bl. 1