A History of Mathematics at Princeton University
Michael E. Seip
History of Mathematics Term Paper, Rutgers, Spring 1999

(old Fine Hall, Princeton University)

(old Fine Hall, Princeton University)
Mathematics is a subject taught at every major academic institution in America. Some institutions foster and develop mathematical research in addition to providing instruction to their constituent student bodies in the subject. Of these research institutions, some have distinguished themselves by both the quantity and the caliber of the research conducted there.
One such is Princeton University, which has distinguished itself in the development of mathematics throughout its two hundred and fifty three-year history, and was a pioneer in higher education in the late nineteenth and early twentieth centuries. Fine Hall's rapid ascent in academic stature, starting in approximately 1880, has attracted some of the most talented and productive scholars in America. In is instructive to look at the emergence of Princeton's mathematics program as a world leader because it reflects the impact that its success had on American higher education as a whole
Two major arenas for mathematical thought exist at Princeton, one being the mathematics department at the university, and the other being the Institute for Advanced Study, which, while technically separate from the university, maintains close ties with it. The department of mathematics at Princeton University is dedicated both to research and to the education and instruction of its undergraduate and graduate students, whereas the Institute for Advanced Study is a post-doctoral research center dedicated the general advancement of learning. The two institutions comprise an academic duality, the notion of separate institutions performing independent research on similar topics promotes collaboration and diversity of thought. These characteristics have made the two entities successful in many fields of study.
The Institute's primary mission is to advance the cutting edge of theory in a number of academic disciplines. Within the Institute, there are schools of mathematics, natural science, and historical studies and social sciences. The schools of mathematics and natural sciences often have collaborating faculty members working in fields such as theoretical physics and astrophysics.
The Institute has up to 130 visiting scientists, called faculty fellows, that are assembled from academic institutions and laboratories around the world. These fellows join the 21 full time faculty members of the Institute in conducting pioneering research in their respective fields. The faculty fellows are all eminent scholars and are offered a fellowship only after a lengthy and thorough worldwide search to fill a vacant position. Some notable past members of the Institute are Albert Einstein, John von Neumann, Kurt Göel.
The mathematics department at Princeton is located in Fine Hall, and it is this term that is often used to refer to the faculty that comprises the department. ( e.g. Fine Hall held Kodaira's work in geometry in high regard) This department went from a primarily teaching institution in 1896 to "a world center in mathematical research and advanced education" (Asprey, p. 195) by the 1930's. The ascent in stature of Fine Hall began in earnest in 1905 with the appointment of Henry Buchard Fine to Dean of the Sciences in 1903. Being firmly established by 1935, the Princeton mathematical community was poised to assume a larger share of war related research than any other university in America, and a larger share of the publicity.
Government agencies like the Department of War found that research into weapons systems and tactics created a more efficient and successful military force. Mathematicians found themselves working on many physical problems encountered in war, such as the optimal trajectories to fire artillery to maximize distance and accuracy, and engineering problems, such as the design of an effective wing for faster fighter aircraft and bombers.
The creation of the Manhattan project began the most intensive and ambitious collaborations in the history of science, and one which Fine Hall played an integral role. Princeton physicists and mathematicians played a key role in the development of the bomb, a fact noted after the successful deployment of the weapon and consequent end of the war in 1945. The scientists were heroes, and many of them were from Princeton.
Those in the scientific community in America would soon find that Princeton scientists were virtually everywhere in the development scheme of the A-bomb. Some were professors in the faculty, others were researchers at the Institute, and many were educated there before moving to other institutions (one account placed 35% of the staff of Los Alamos as either Princeton faculty members, or Ph.D. recipients). This placed both Fine Hall and the Institute in the spotlight and led to its dramatic increase in selectivity and reputation. Life at Fine Hall became a benchmark for academic stimulation, though not without its own difficulties, as is discussed in section 3.
Mathematics has been prominent in the Princeton curriculum since the founding of the college. In 1760, entering students were required, "to have an understanding of the rules of arithmetic, and underclassmen learned algebra, trigonometry, geometry, and conic sections."(Leitch, Mathematics, p.1) Prior to the turn of the twentieth century, the education provided was a largely classical one, where students were instructed by methods similar to those used in ancient Greece. The first trained mathematician to be a member of the Princeton faculty was appointed in 1787. After him it was another century until Princeton "again had a mathematician who had studied at the European mathematical centers" (Leitch, Mathematics, p. 1); the mathematician was H. B. Fine.
Fine completed his undergraduate work in classics at Princeton in 1880, and stayed until 1884 as a fellow in experimental physics and mathematics. He traveled to Germany to study under eminent geometer Felix Klein, returning to Princeton with his Ph.D. in 1885 to become an assistant professor. He had a thorough knowledge of the German philosophy and method of academia, and he progressed rapidly through the ranks, becoming the senior member of the department in 1900. He was a close personal friend of Woodrow Wilson, the president of the university, and encouraged the adoption of the preceptorial method of student instruction, especially in mathematics. The success of the method was immediate and marked, and the ideas took root.
Fine was successively chairman of the department(1904), dean of the faculty(1903), and dean of the science departments(1909) under university president Woodrow Wilson. It is said that Fine's fondness for the German style is what made him successful not only as a scientist, but also as an educator. This style stresses open exchange of ideas and efficient work methods. Wilson and Fine pioneered the development of the preceptorial system of instruction in America. This system provides for smaller classes with more personalized instruction by faculty members. "The term `preceptor' appears in An Account of the College of New Jersey(1764): `In the instruction of the youth, care is taken to.encourage their right of private judgement, without.demanding an implicit assent to the decisions of the preceptor' " (Leitch, Preceptorial method, p. 2). It is still today used at Princeton and at most other Ivy League schools, but at the time was a radical change in the course of instruction and directly led to the establishment of American higher education as comparable to that in Europe.
Fine also was directly responsible for the creation of a strong mathematics department at Princeton. When he was appointed department chair, the mathematics faculty was comprised of only eight members. Undergraduate teaching loads were heavy, salaries were low, and the opportunities for research were very limited. All in all, it was not an environment conducive to productive research. Fine planned to establish credibility and strength within the department by appointing young mathematicians with great promise to preceptorial positions. In 1905, he appointed, among others, Gilbert Bliss, Luther Eisenhart, Oswald Veblen, and John Wesley Young.
In a move "entirely uncharacteristic of American mathematical institutions" (Aspray, p. 197), Fine appointed English-speaking European mathematicians. In 1905, he appointed James Jeans to a position in applied math, and offered a position to Arthur Eddington, who subsequently declined to work at the Greenwich Observatory. As members left the department for other institutions, they were replaced by other notable mathematicians, so that between 1905 and 1925 many of the young mathematicians who were to become leaders in American math studies were at one time members of the Princeton faculty. Fine had a keen skill in spotting mathematical talent and the accomplishments of professors he appointed stands testament to his judgement.
Once the faculty had been sufficiently developed, Fine recognized the need to develop other areas of administration. Research was still somewhat subordinate to instruction, so Fine sought to strengthen the research environment in the department. He recognized that several requirements had to be fulfilled in order to promote an effective research program. Fine, along with Veblen and Eisenhart, surmised that 7 criteria were necessary as "means to the full realization for the purposes of the Mathematics Department". These seven criteria were (Fine, 1926):
Veblen made similar arguments to the General Education Board (GEB) of the Rockefeller Foundation; however, he placed greater stress on the notion of an institute dedicated to advanced research and instruction. This was the beginning of the Institute for Advanced Study. The GEB awarded Princeton a $1million dollar grant for advancement of the fundamental sciences(with an agreement to raise $2 million in alumni donations), of which the mathematics department received $600,000. This money served to buy library materials, support the journal Annals of Mathematics, reduce teaching loads, and pay visiting professors salaries.
In 1926, Fine approached Thomas Jones, a former classmate, about endowing a chair in the mathematics department. What resulted was the Fine professorship, and three chairs, including the Jones chair in mathematical physics. This demonstrated Fine's ability to unite commercial and academic ventures into a single productive process, which has since been much duplicated. The money obtained from this grant also helped fulfill some of the desired criteria by paying for top-notch academics to come to Princeton.
The last remaining criterion on Fine's list was permanent space for offices and the pursuit of research. In an ironic twist of fate, Henry Fine's accidental death in 1928 prompted the Jones family to donate funds for the construction of old Fine Hall, the home of the Princeton Mathematics Department. Design of the building was referred to Veblen and Wedderburn, and Veblen took charge to create an environment that, as Jones said, "any mathematician would be loath to leave"(Princeton University Alumni Weekly 1931, pg 113).
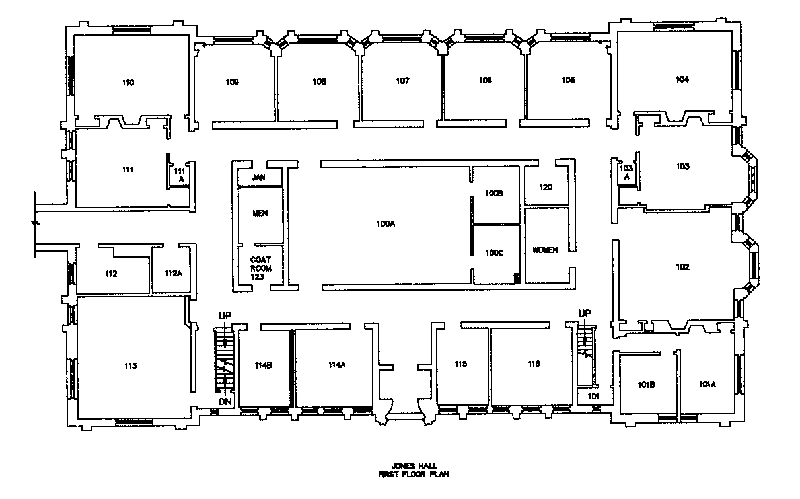
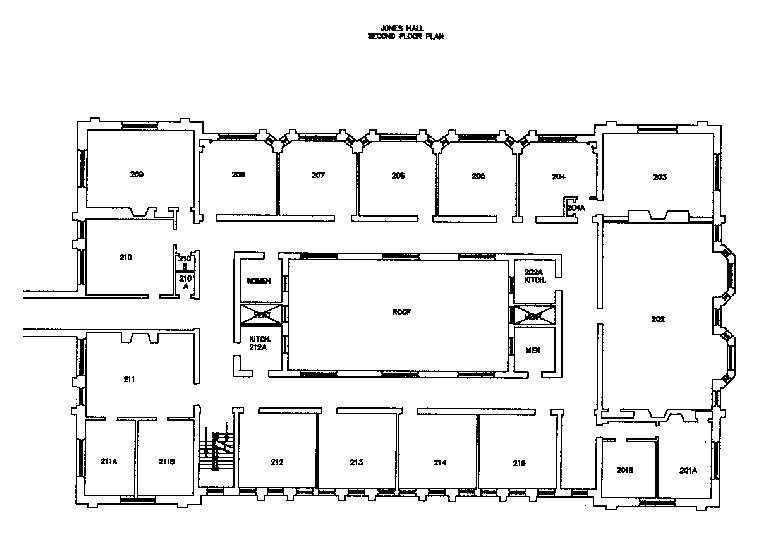
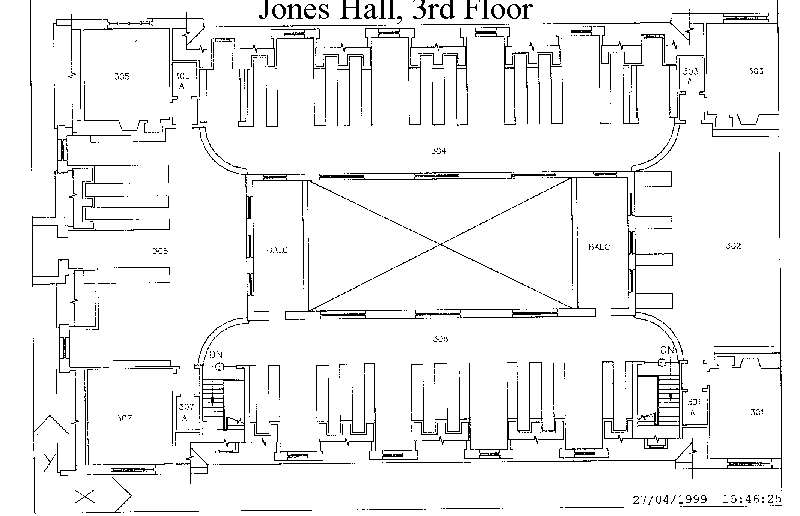
The building itself is an architectural masterpiece, stressing communication in its very design. Veblen placed the common room on the first floor so that every visitor had to pass through it on the way to the library, located on the third floor. An open central court near the library allowed groups to congregate, though Asprey states that most mathematicians usually used the room to read. If discussion was desired, faculty members could use chalkboards located on the periphery of the interior.
Professors were not given offices, but rather studies. This creates an informal environment wherein students and professors could meet and talk. These studies were often lavish, with some having fireplaces, ornate rugs and vases, and anterooms. The subtleties of the building were as important as the fundamental design; the leaded design of the windows bearing mathematical symbols, and Einstein's famous remark placed over the fireplace in what is now the lounge: Raffiniert ist der Herr Gott, aber boshaft ist Er nicht (The Lord is sophisticated, but He is not malicious)." (Leitch, Jones Hall, p. 2) These studies were highly desirable, and were a factor in leading mathematicians at other institutions to strive to teach at Princeton. The floorplan of old Fine Hall is attached(see Appendix), and the open forum design of the building is evident.

In 1969, the department grew to five times its size in 1931,
prompting the construction of a new
building (shown on the right) with larger facilities. The
new design "was a sharp contrast to
the old building, but to mark fittingly the continuity
of what had by now become the
distinguished tradition of Princeton mathematics, the name
"Fine Hall" was transferred
to the new facility" (Leitch, Mathematics, p. 3), and the old
building was renamed Jones Hall
in honor of the original donors. Jones Hall is currently
used to house the Language Studies
Laboratory and the Asian Studies Department.
Princeton continued to appoint established leaders in mathematics along with promising young faculty members. Fine established the well known non-tenure track process of professorship. When an addition to the faculty is desired, a notice is sent out in leading mathematical publications announcing the fact. A selection committee will appoint the mathematician to a 6-year term as associate professor. The unique facet of this process is that the new faculty member is told that he/she cannot expect to receive tenure. However if the mathematician produces work of outstanding impact and caliber during his time as associate professor, he may be considered for tenure.
Often when a senior faculty appointment is being made, the department will hire from outside the university, rather than promote from within. If a tenured professorship becomes available, a worldwide search is initiated for the best candidates available. It is through this selectivity and rigidity that Princeton has maintained the caliber of mathematician that it has.
In an environment where leading intellectuals met and worked, it is no surprise to say that the Princeton mathematical community was a unique and interesting one in the growth years of the early 20th century. The feeling of the time was that of great change and upheaval. The balance of world power was in question throughout the first 50 years of the century, and entirely new ideas on the nature of the universe were being set forth and developed. Many of the scientists at Princeton were directly involved in scientific decisions by the government, and this played no small part in the drama and tension of the era.
J. Robert Oppenheimer, for example, was the director of the Manhattan project, and later appointed director of the Institute for Advanced Study. H.B. Fine was president of the New York Mathematical Association, which later, under his leadership, became the American Mathematical Society (AMA). This organization has large control over federal funds devoted to mathematical research. Nuclear physics was experiencing a renaissance following the atomic bomb, and theorists were scrambling to work in the field.
Gian Carlo Rota was an undergraduate mathematics major at Princeton in the early 1950's, a period marked by growth of the department and political upheaval in academia. He describes another, more ugly, notion of the times; nationality prejudices in academia. Many notable professors from Europe and the Far East were allowed to visit American universities, but not many were granted tenure. This bias was expressed at Princeton in several notable instances. The geometer Kunihiko Kodaira, from Japan, was appointed to a six-year term but was not awarded tenure. Though Kodaira, "whose work in geometry was revered by everyone in the Princeton main line", (Rota, p. 226) was an accomplished and productive researcher, several members of the Princeton faculty were opposed to his award of tenure on the basis of his nationality and he was voted down.
Bias also existed towards those men who studied subjects considered intangible at the time. The great example of this mindset, which seemingly violates everything that an academic institution embodies, lies in the story of Alonzo Church. Church was a world leader in mathematical logic, a subject looked down upon at Princeton because of its close relation with pure philosophy. He encountered opposition from fellow faculty members who studied more traditional and "accepted" disciplines, to the point where many refused to review his papers prior to publication. This treatment of an eminent scholar led John Kemeny, another respected mathematician at Princeton, to say to lecture of graduate students, "There is no reason why a great mathematician should not also be a great bigot. Look at your teachers in Fine Hall, at how they treat one of the greatest living mathematicians, Alonzo Church." (Rota, p. 226) Kemeny later left Princeton for Dartmouth, where he later became president. These instances reinforce the belief of some in the field that " a good mathematician is not necessarily a "nice" guy."(Rota, p. 226)
Despite some shortcomings, the mood around Fine Hall in the earlier half of this century was overwhelmingly positive. The faculty of the mathematics department and many theoretical physicists would meet in the common area of Fine Hall on Fridays for tea, and the opportunity to discuss progress in their work. The "hot" topics of the day were analytical number theory, set theory, abstract algebra, and the theory of rings. Faculty in both Fine Hall and the Institute were eager to work in three areas especially, operator theory, topology and analytical number theory. John von Neumann was the world authority in operator theorem, and it was considered vital to the continued development of quantum mechanics. G.H Hardy developed the definitive treatise on analytical number theory while at Cambridge in 1917, and was later offered a position at the Institute, which he declined because of both loyalty to Cambridge and apprehension in changing affiliations late in his career.
When the Institute for Advanced Study was founded in 1933. It became a matter of concern that it would detract from the quality of mathematicians in Fine Hall. Of the initial six faculty members of the Institute, three originally taught at Princeton. These three, John von Neumann, Oswald Veblen, and Marston Morse, were eminent scholars and the University took notice of their departure. Fine Hall struck an agreement to limit the number of mathematicians that were appointed to the institute from Fine Hall. While the institute still insisted on choosing men according to their qualifications and not affiliations, they agreed to only offer appointments as such that will not diminish the department's stature.
Princeton University, through the vision and guidance of several men, has established itself one of the best and most productive mathematical centers in the world. The attached U.S. News and World Report ranking of mathematics departments (see the Appendix) cites Princeton as the number one department in the country, tied with the University of California at Berkeley.
The 1950's and 1960's "heightened national interest in science and mathematics following Sputnik and the space program led to a further expansion of the department" (Leitch,Mathematics, p.3). Today, research continues on areas at the forefront of modern mathematical thought. Appointments in the 1970's of scholars such as Charles Feffernan, himself a Princeton Ph.D. recipient, and John MacLean, " gave Princeton a strong faculty in topology (traditionally an area of excellence at Princeton)" (Leitch, Mathematics, p.3). The Fine Hall of today has strength in many of the same fields as in the 1940's.
The attached faculty fields list shows that the majority of research at Fine Hall today lies in either topology, number theory, and analysis, just as it did over sixty years ago (see the Princeton University Mathematics Department site for a list of current faculty and their interests). The tradition of excellence at Fine Hall has made it something of a Mecca for mathematicians internationally, and the number and quality of distinguished visiting faculty members supports this.
Princeton's position today at the apex of mathematical thought is a direct result of innovative thinking in the past. Many historians of American education look at Princeton's success as a blueprint for a practical and prosperous schooling.
| Atlantic Coast | Big West | Qd 3 | Pacific Ten | Ivy League |
| 18. Maryland | 106. North Texas | 36. Utah | 1. Berkeley | 1. Princeton |
| 34. Duke | 111. NM State | -- Brigham Young | 6. Stanford | 4. Harvard |
| 42. North Carolina | -- Idaho | -- New Mexico | 12. UCLA | 7. Yale |
| 44. Georgia Tech | -- Nevada | -- UTEP | 26. Washington | 9. Columbia |
| 45. Virginia | -- Utah State | Qd 4 | 43. Southern Cal | 15. Cornell |
| 58. NC State | -- Boise State | 98. Hawaii | 47. Oregon | 16. Brown |
| 82. Florida State | -- San Diego State | 54. Arizona | 22. Pennsylvania | |
| 92. Clemson | Southeastern | -- San Jose State | 85. Arizona State | 53. Dartmouth |
| -- Wake Forest | East | -- Fresno State | 90. Oregon State | Big East |
| Big Twelve | 55. Florida | Div 1A Independents | 104. Wash. State | 19. Rutgers |
| South | 58. Georgia | 46. Notre Dame | Mid-American | 61. Pittsburgh |
| 23. Texas | 71. Kentucky | 134. SW Louisiana. | East | 66. Virginia Tech |
| 63. Texas A&M | 75. South Carolina | -- Central Florida | 66. Buffalo | 72. Temple |
| 99. Oklahoma | 77. Tennessee | -- Louisiana Tech | 86. Kent State | 73. Syracuse |
| 107. Texas Tech | 88. Vanderbilt | -- NE Louisiana | 114. Bowling Green | 103. Miami |
| -- Oklahoma State | West | -- Navy | 124. Ohio | -- W. Virginia |
| -- Baylor | 69. LSU | -- Arkansas State | -- Akron | -- Boston College |
| North | 93. Auburn | Big Ten | -- Marshall | Atlantic 10 |
| 65. Colorado | 126. Alabama | 9. Michigan | -- Miami | 51. Boston University |
| 77. Iowa State | 136. Mississippi | 13. Wisconsin | West | 58. Massachusetts |
| 87. Nebraska | -- Arkansas | 14. Minnesota | 113. W. Michigan | 101. Connecticut |
| 91. Kansas State | -- Mississippi State | 21. Illinois | 118. N. Illinois | 124. Rhode Island |
| 94. Missouri | 24. Purdue | -- Ball State | -- New Hampshire | |
| -- Kansas | Western Athletic | 28. Northwestern | -- Central Michigan | -- Maine |
| Conf. USA | Qd 1 | 29. Ohio State | -- Eastern Michigan | Middle Atlantic |
| 68. Houston | 24. Rice | 34. Indiana | -- Toledo | 80. Delaware |
| 77. Tulane | 116. SMU | 37. Penn State | 81. Northeastern | |
| 106. Cincinnati | -- Texas Christian | 48. Michigan State | -- Richmond | |
| -- Army | -- Tulsa | 62. Iowa | -- James Madison | |
| -- Birmingham | Qd 2 | -- William and Mary | ||
| -- East Carolina | 96. Colorado State | -- Villanova | ||
| -- Memphis | 125. Wyoming | |||
| -- Southern Miss | -- UNLV | |||
| -- Louisville | -- Air Force |
Alexander Leitch, A Princeton Companion, Princeton University Press, 1978
William Aspray and Philip Kitcher, The Emergence of Princeton as a World Center for Mathematical Research, 1896-1939, from History and Philosophy of Modern Mathematics, University of Minnesota Press, Minneapolis, 1988
Gian-Carlo Rota, Fine Hall in its golden age: Rembrances of Princeton in the early fifties, Sloan Science Series
Princeton University Alumni Weekly, vol. 1931
Mathematics Departments, Ranked and Sorted by Conference, US News and World Report, February 1997
Floorplans obtained from the Princeton University website